POJ1037 A decorative fence 【动态规划】

A decorative fence

Time Limit: 1000MS		Memory Limit: 10000K
Total Submissions: 6489		Accepted: 2363

Description

Richard just finished building his new house. Now the only thing the house misses is a cute little wooden fence. He had no idea how to make a wooden fence, so he decided to order one. Somehow he got his hands on the ACME Fence Catalogue 2002, the ultimate resource
on cute little wooden fences. After reading its preface he already knew, what makes a little wooden fence cute.

A wooden fence consists of N wooden planks, placed vertically in a row next to each other. A fence looks cute if and only if the following conditions are met:

�The planks have different lengths, namely 1, 2, . . . , N plank length units.

�Each plank with two neighbors is either larger than each of its neighbors or smaller than each of them. (Note that this makes the top of the fence alternately rise and fall.)

It follows, that we may uniquely describe each cute fence with N planks as a permutation a1, . . . , aN of the numbers 1, . . . ,N such that (any i; 1 < i < N) (ai − ai−1)*(ai − ai+1) > 0 and vice versa, each such permutation describes a cute fence.

It is obvious, that there are many di erent cute wooden fences made of N planks. To bring some order into their catalogue, the sales manager of ACME decided to order them in the following way: Fence A (represented by the permutation a1, . . . , aN) is in the
catalogue before fence B (represented by b1, . . . , bN) if and only if there exists such i, that (any j < i) aj = bj and (ai < bi). (Also to decide, which of the two fences is earlier in the catalogue, take their corresponding permutations, find the first
place on which they differ and compare the values on this place.) All the cute fences with N planks are numbered (starting from 1) in the order they appear in the catalogue. This number is called their catalogue number.

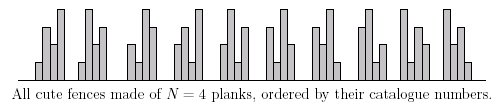
After carefully examining all the cute little wooden fences, Richard decided to order some of them. For each of them he noted the number of its planks and its catalogue number. Later, as he met his friends, he wanted to show them the fences he ordered, but
he lost the catalogue somewhere. The only thing he has got are his notes. Please help him find out, how will his fences look like.

Input

The first line of the input file contains the number K (1 <= K <= 100) of input data sets. K lines follow, each of them describes one input data set.

Each of the following K lines contains two integers N and C (1 <= N <= 20), separated by a space. N is the number of planks in the fence, C is the catalogue number of the fence.

You may assume, that the total number of cute little wooden fences with 20 planks fits into a 64-bit signed integer variable (long long in C/C++, int64 in FreePascal). You may also assume that the input is correct, in particular that C is at least 1 and it
doesn抰 exceed the number of cute fences with N planks.

Output

For each input data set output one line, describing the C-th fence with N planks in the catalogue. More precisely, if the fence is described by the permutation a1, . . . , aN, then the corresponding line of the output file should contain the numbers ai (in
the correct order), separated by single spaces.

Sample Input

Sample Output

1 2

2 3 1

⊙﹏⊙ 这题做了整整一天，看着讲义做的，理解起来比扫描线还难..算是我眼下碰到的最难的动规题了..

题意：给定长度依次为1到n的木棒n个。摆放规则为除了两边的木棒。剩下的木棒必需要比相邻的两个都高或者都低。求从小到大排列的第c个序列。

题解：状态方程dp[i][j][0]用来求在i个木棒中以第j根木棒打头且前两根为升序的排列方案数，dp[i][j][1]用来求在i个木棒中以第j根木棒打头且前两根为降序的排列方案数。详解在讲义代码的凝视里。

#include <stdio.h>

#include <string.h>

#define maxn 22

#define UP 0

#define DOWN 1

int vis[maxn], ans[maxn];

__int64 dp[maxn][maxn][2];

void Init(int n)

{

	int i, j, k;

	dp[1][1][UP] = dp[1][1][DOWN] = 1;

	for(i = 2; i <= n; ++i){ //i is the amount of total sticks

		for(j = 1; j <= i; ++j){ //j is the first stick's position

			for(k = j; k < i; ++k) //k is the subSolution of dp array

				dp[i][j][UP] += dp[i-1][k][DOWN];

			for(k = 1; k < j; ++k)

				dp[i][j][DOWN] += dp[i-1][k][UP];

		}

	}

}

void Print(int n, __int64 c)

{

	memset(vis, 0, sizeof(vis));

	int i, j, k, rank;

	__int64 skip = 0, pre = 0;

	for(i = 1; i <= n; ++i){ //i is the position to select now

		rank = 0; //the rank's min stick

		for(j = 1; j <= n; ++j){ //j is the stick to be select

			pre = skip;

			if(!vis[j]){

				++rank;

				if(i == 1) skip += dp[n][rank][UP] + dp[n][rank][DOWN];

				else if(j > ans[i-1] && (i == 2 || ans[i-2] > ans[i-1]))

					skip += dp[n-i+1][rank][DOWN];

				else if(j < ans[i-1] && (i == 2 || ans[i-2] < ans[i-1]))

					skip += dp[n-i+1][rank][UP];

				if(skip >= c) break;

			}

		}

		ans[i] = j;

		vis[j] = 1;

		skip = pre;

	}

	for(i = 1; i <= n; ++i)

		if(i != n) printf("%d ", ans[i]);

		else printf("%d\n", ans[i]);

}

int main()

{

	int t, n;

	__int64 c;

	Init(20);

	scanf("%d", &t);

	while(t--){

		scanf("%d%I64d", &n, &c);

		Print(n, c);

	}

	return 0;

}

附讲义代码：

#include <stdio.h>

#include <string.h>

#define UP 0

#define DOWN 1

#define maxn 25

long long C[maxn][maxn][2];

//C[i][k][DOWN] 是S(i)中以第k短的木棒打头的DOWN方案数,C[i][k][UP]

//是S(i)中以第k短的木棒打头的UP方案数,第k短指i根中第k短

void Init(int n)

{

    memset(C, 0, sizeof(C));

    C[1][1][UP] = C[1][1][DOWN] = 1;

    int i, k, M, N;

    for(i = 2; i <= n; ++i){

        for(k = 1; k <= i; ++k){ //枚举第一根木棒的长度

            //枚举第二根长度

            for(M = k; M < i; ++M) C[i][k][UP] += C[i-1][M][DOWN];

            //枚举第二根长度

            for(N = 1; N < k; ++N) C[i][k][DOWN] += C[i-1][N][UP];

        }

    }

    //总方案数是 Sum{ C[n][k][DOWN] + C[n][k][UP] } k = 1.. n;

}

void Print(int n, long long cc)

{

    long long skipped = 0, oldVal; //已经跳过的方案数

    int seq[maxn]; //终于要输出的答案

    int used[maxn]; //木棒是否用过

    memset(used, 0, sizeof(used));

    for(int i = 1, k; i <= n; ++i){ //依次确定位置i的木棒序号

        int No = 0;

        for(k = 1; k <= n; ++k){ //枚举位置i的木棒

            oldVal = skipped;

            if(!used[k]){

                ++No; //k是剩下木棒里的第No短的

                if(i == 1) skipped += C[n][No][UP] + C[n][No][DOWN];

                //下面寻找合法位置

                else if(k > seq[i-1] && (i == 2 || seq[i-2] > seq[i-1]))

                    skipped += C[n-i+1][No][DOWN];

                else if(k < seq[i-1] && (i == 2 || seq[i-2] < seq[i-1]))

                    skipped += C[n-i+1][No][UP];

                if(skipped >= cc) break;

            }

        }

        used[k] = 1;

        seq[i] = k;

        skipped = oldVal;

    }

    for(int i = 1; i <= n; ++i)

        if(i < n) printf("%d ", seq[i]);

        else printf("%d\n", seq[i]);

}

int main()

{

    int T, n; long long c;

    Init(20);

    scanf("%d", &T);

    while(T--){

        scanf("%d%lld", &n, &c);

        Print(n, c);

    }

    return 0;

}