数学（矩阵乘法）：HDU 4565 So Easy!

So Easy!

Time Limit: 2000/1000 MS (Java/Others)    Memory Limit: 32768/32768 K (Java/Others)
Total Submission(s): 3804    Accepted Submission(s): 1251

Problem Description

　　A sequence S_n is defined as:

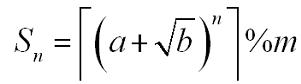
Where a, b, n, m are positive integers.┌x┐is the ceil of x. For example, ┌3.14┐=4. You are to calculate S_n.
　　You, a top coder, say: So easy!

 

Input

　　There are several test cases, each test case in one line contains four positive integers: a, b, n, m. Where 0< a, m < 2¹⁵, (a-1)²< b < a², 0 < b, n < 2³¹.The input will finish with the end of file.

 

Output

　　For each the case, output an integer S_n.

 

Sample Input

2 3 1 2013
2 3 2 2013
2 2 1 2013

 

Sample Output

4
14
4

　　这道题还是有点门路的，需要一些巧法。

　　由于(a-1)²< b < a²，可以得出a-1<sqrt(b)<a,0<a-sqrt(b)<1。

　　这时构建E(n)=(a+sqrt(b))^n+(a-sqrt(b))^n,发现E(n)是整数，而且(a-sqrt(b))^n小于1，那么(a+sqrt(b))^n向上取整就是E(n)。

　　通过推导可以得出E(0)=2,E(1)=2*a,E(n)=2*a*E(n-1)-(a*a-b)*E(n-2),用矩阵乘法快速求出即可。

 #include <iostream>
 #include <cstring>
 #include <cstdlib>
 #include <cstdio>
 using namespace std;
 const int maxn=;
 int a,b,n,m;
 struct Array{
     int a[maxn],L;
     void Init(int x){L=x;memset(a,,sizeof(a));}
     int *operator[](int x){return &a[(x-)*L];}
 };
 struct Matrix{
     int R,C;
     Array mat;
     void Init(int r,int c){mat.Init(c);R=r;C=c;}
     int *operator[](int x){return mat[x];}
     friend Matrix operator*(Matrix a,Matrix b){
         Matrix c;c.Init(a.R,b.C);
         for(int i=;i<=a.R;i++)
             for(int j=;j<=b.C;j++)
                 for(int k=;k<=a.C;k++)
                     (c[i][j]+=1ll*a[i][k]*b[k][j]%m)%=m;
         return c;
     }
     friend Matrix operator^(Matrix a,int k){
         Matrix c;c.Init(a.R,a.C);
         for(int i=;i<=a.R;i++)c[i][i]=;
         while(k){if(k&)c=c*a;k>>=;a=a*a;}
         return c;
     }
 }A,B;

 int main(){
     while(scanf("%d%d%d%d",&a,&b,&n,&m)!=EOF){
         A.Init(,);
         A[][]=*a%m;A[][]=(b-1ll*a*a%m+m)%m;
         A[][]=;A[][]=;

         B.Init(,);
         B[][]=*a%m;
         B[][]=;
         A=A^(n-);B=A*B;
         printf("%d\n",B[][]);
     }
     return ;
 }