[SDOI2011]消耗战 题解

题面

虚树的模板题：

虚树的思想是只保留有用的点（在这道题目里面显然是标记点和lca），然后重新构建一棵树，从而使节点大大减少，优化复杂度

我们维护一条链(以1号点为根)，这条链左边的所有在虚树上的位置都已经处理完毕；而这条链右边的和下面的都未处理；

这条链我们用栈来维护；

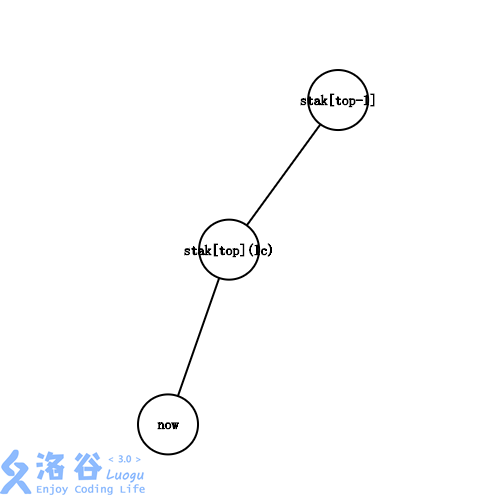
对于要新加的询问点now，对于虚树的影响有四种情况：(lc表示x与st[top]的LCA)

1.lc==st[top]      ：  在虚树上连接st[top]与now

.

2.lc在st[top]与st[top-1]之间；在虚树上连接lc与st[top],--top,然后now进栈；

3.lc==st[top-1]    ：--top，然后now进栈；

4.lc在st[top-1]之上 ：在虚树上连接st[top-1]与st[top]，然后退栈，重复以上步骤知道出现情况1、2、3；

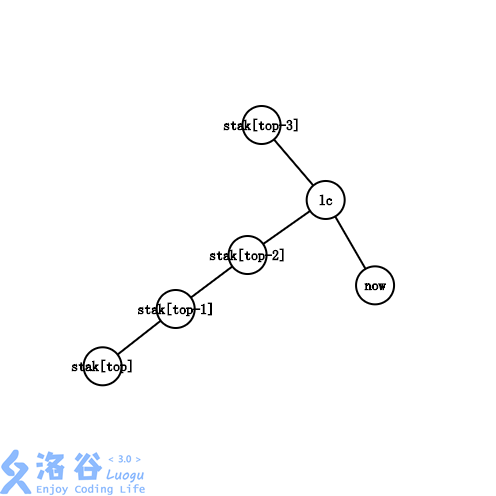
在最后，我们把这条链加入到虚树中，这样一颗完美的虚树就建成了；

在每次建立虚树的时候，我们要实时清空虚树，否则时间复杂度会退化成O(n^2);

然后在这个虚树上跑dp，就可以了；

#include <bits/stdc++.h>
#define int long long
#define inc(i,a,b) for(register int i=a;i<=b;i++)
#define dec(i,a,b) for(register int i=a;i>=b;i--)
using namespace std;
int head1[500010],cnt1,head2[500010],cnt2;
class littlestar{
	public:
	int to,nxt;
	long long w;
	void add1(int u,int v,long long gg){
		to=v; nxt=head1[u];
		head1[u]=cnt1; w=gg;
	}
	void add2(int u,int v){
		to=v; nxt=head2[u];
		head2[u]=cnt2;
	}
}star1[500010*2],star2[500010*2];
int n,f[500010][25],dfn[500010],cur,dep[500010];
long long minv[500010];
void dfs(int u)
{
	inc(i,0,19){
		f[u][i+1]=f[f[u][i]][i];
	}
	dfn[u]=++cur;
	for(int i=head1[u];i;i=star1[i].nxt){
		int v=star1[i].to;
		if(!dfn[v]){
			f[v][0]=u;
			dep[v]=dep[u]+1;
			minv[v]=min(minv[u],star1[i].w);
			dfs(v);
		}
	}
}
int lca(int x,int y)
{
	if(dep[x]<dep[y]) swap(x,y);
	dec(i,20,0){
		if(dep[f[x][i]]>=dep[y]) x=f[x][i];
	}
	if(x==y) return x;
	dec(i,20,0){
		if(f[x][i]!=f[y][i]){
			x=f[x][i]; y=f[y][i];
		}
	}
	return f[x][0];
}
bool cmp(int x,int y){return dfn[x]<dfn[y];}
int num;
int judge[500001],h[500010];
int st[500010],top;
int dp(int u)
{
	long long summ=0;
	for(int i=head2[u];i;i=star2[i].nxt){
		int v=star2[i].to;
		summ+=dp(v);
	}
	long long ans=0;
	if(judge[u]) ans=minv[u];
	else ans=min(minv[u],summ);
	judge[u]=0;
	head2[u]=0;
	return ans;
}
signed main()
{
	cin>>n;
	inc(i,1,n-1){
		int u,v; long long w;
		scanf("%lld%lld%lld",&u,&v,&w);
		star1[++cnt1].add1(u,v,w);
		star1[++cnt1].add1(v,u,w);
	}
	minv[1]=1e17+21;
	dfs(1);
	int q; scanf("%lld",&q);
	while(q--){
		scanf("%lld",&num);
		inc(i,1,num){
			scanf("%lld",&h[i]);
			judge[h[i]]=1;
		}
		sort(h+1,h+1+num,cmp);
		top=1;
		st[top]=h[1];
		inc(i,2,num){
			int LCA=lca(h[i],st[top]);
			while(true){
				if(dep[LCA]>=dep[st[top-1]]){
					if(LCA!=st[top]){
						star2[++cnt2].add2(LCA,st[top]);
						if(LCA!=st[top-1]){
							st[top]=LCA;
						}
						else{
							--top;
						}
					}
					else{
						break;
					}
				}
				else{
					star2[++cnt2].add2(st[top-1],st[top]);
					--top;
				}
			}
			st[++top]=h[i];
		}
		while(--top){
			star2[++cnt2].add2(st[top],st[top+1]);
		}
		cout<<dp(st[1])<<endl;
		cnt2=0;
	}
}