普林斯顿微积分读本 大纲与重点 (by zzd)
普林斯顿微积分读本 大纲重点
由于博客园太菜,所以我用图片上传。
当前更新状态:未完待续,挖坑暂时不填了。
UPD(2018-07-08): 稍微更一下,加一个本书的某一版本下载链接:https://pan.baidu.com/s/12DWtOkB2IjmQjnpwVZ_VvA
UPD(2019-02-27):
我决定草率的更新一下。(之前的在后面……)
有些简单的结论我就不证明了。
$$\frac {d}{dx}(x^a) = ax^{a-1}$$
乘积法则:若 $h(x) = f(x) g(x) $ ,则 $h'(x) = f'(x) g(x) =f(x) g'(x) $ .
证明:
$$h'(x) = \lim_{d\rightarrow 0} \frac {f(x+d)g(x+d) -f(x)g(x)}{d}\\=\lim_{d\rightarrow 0}\frac{(f(x+d)-f(x)+f(x))(g(x+d)-g(x)+g(x))-f(x)g(x)}{d}\\=\lim_{d\rightarrow 0}\frac{(f(x+d)-f(x))g(x)+f(x)(g(x+d)-g(x))+(f(x+d)-f(x))(g(x+d)-g(x))}{d}\\=\lim_{d\rightarrow 0}\frac{f(x+d)-f(x)}{d}g(x) + \frac{g(x+d)-g(x)}d f(x)\\=f'(x) g(x) =f(x) g'(x) $$
商法则:若 $h(x) = \frac {f(x)}{g(x)} $ ,则 $h'(x) = \frac{f'(x)g(x) -f(x)g'(x)}{g^2(x)}$ .
证明:
$$h'(x) = \lim_{d\rightarrow 0} \cfrac{\frac{f(x+d)}{g(x+d)}-\frac{f(x)}{g(x)}}{d}\\= \lim_{d\rightarrow 0} \cfrac{\frac{f(x+d)g(x)-f(x)g(x+d)}{g(x+d)g(x)}}{d}\\= \lim_{d\rightarrow 0} \frac{(f(x+d)-f(x))g(x)-(g(x+d)-g(x))f(x)}{g(x+d)g(x)d}\\= \lim_{d\rightarrow 0}h'(x) = \frac{f'(x)g(x) -f(x)g'(x)}{g^2(x)}$$
链式求导法则:若 $h(x) =f(g(x))$ ,则 $h'(x) = f'(g(x))g'(x)$.
证明:
$$h'(x) = \lim_{d\rightarrow 0} \frac{f(g(x+d))-f(g(x))}{d}\\=\lim_{d\rightarrow 0} \frac{(f(g(x))+f'(g(x))(g(x+d)-g(x)))-f(g(x))}{d}\\=\lim_{d\rightarrow 0} \frac{f'(g(x))(g(x+d)-g(x))}{d}\\=f'(g(x))g'(x)$$
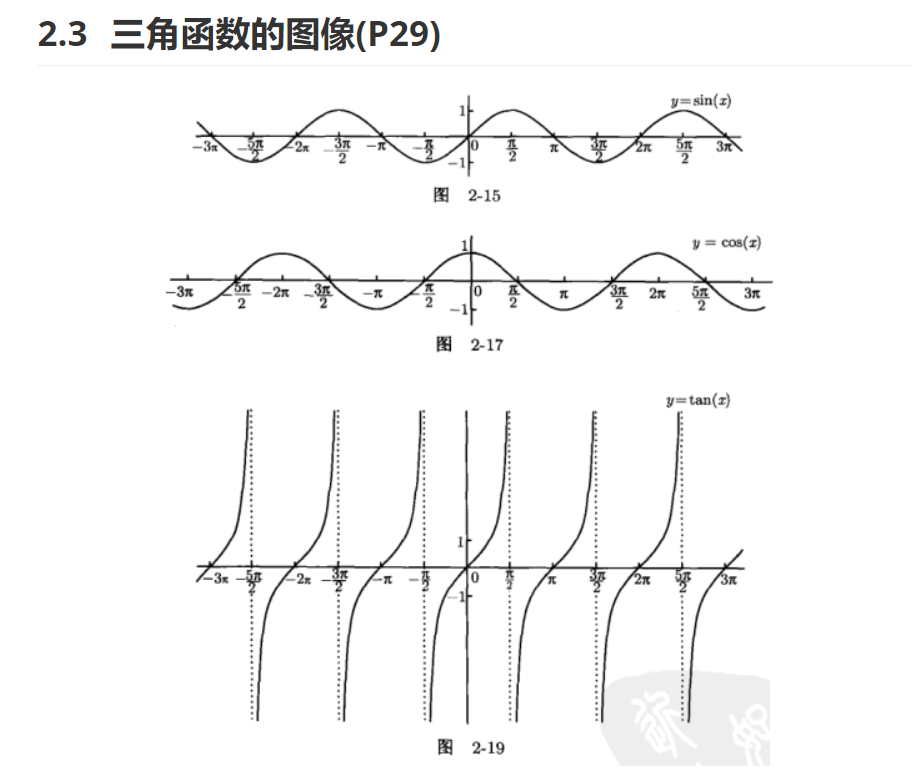
三角函数相关
$$\lim_{x\rightarrow0}\frac{\sin(x)}{x}=1$$
证明:
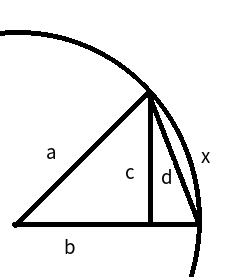
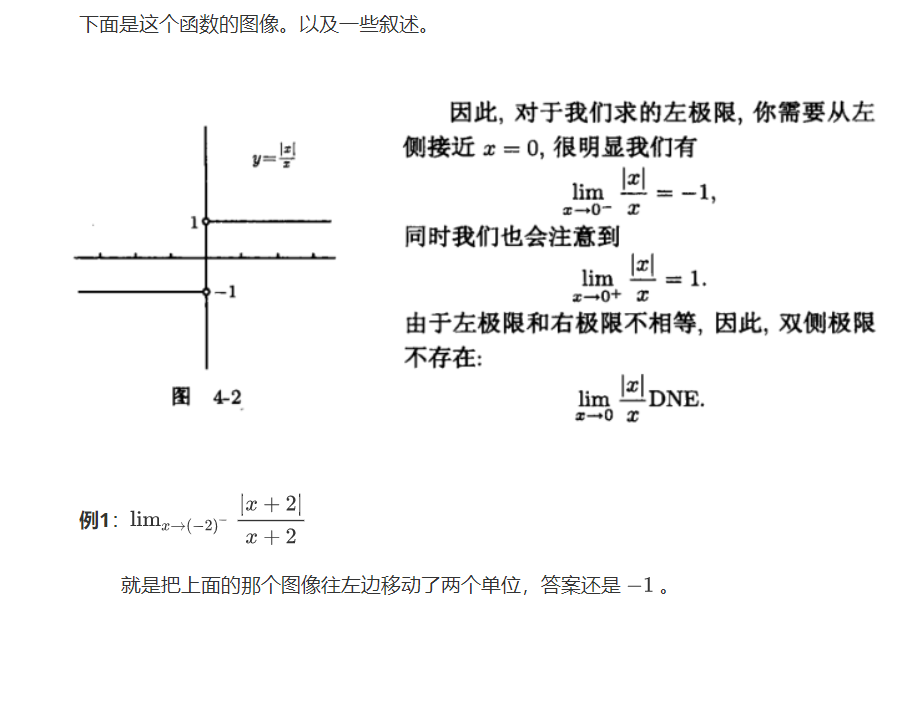
首先画个单位圆(半径为1的圆),在上面画个三角形,设x是那个圆心角的弧度(数值上也等于对应的圆弧的长度),现在我们看看 $sin(x)$ 是什么:
$sin(x) = c/a = c$
当 $x$ 趋于0的时候,$c/d$ 和 $d/x$ 都趋于 1 ,所以 $c/x = (c/d)/(d/x)$ 也趋于1 ,所以这个结论得证。
$$\lim_{x\rightarrow0}\frac{1-\cos(x)}{x}=0$$
证明:
$$\lim_{x\rightarrow0}\frac{1-\cos(x)}{x} = \lim_{x\rightarrow0}\frac{(1-\cos(x))(1+\cos(x))}{x}\cdot \frac{1}{1+\cos(x)}\\=\lim_{x\rightarrow0}\frac{\sin^2(x)}{x}\cdot 0.5\\=0$$
$$\sin'(x) = \cos(x)$$
证明:
$$\sin'(x) = \lim_{d\rightarrow 0 }\frac{\sin(x+d) -\sin(x)}{d} \\= \lim_{d\rightarrow 0 }\frac{\sin(x)\cos(d)+\sin(d)\cos(x)-\sin(x)}{d}\\= \lim_{d\rightarrow 0 } \frac{\sin(x)(1-\cos(d))+\cos(x)\sin(d)}{d}\\=\lim_{x\rightarrow0}0+\cos(x)\\=\cos(x)$$
类似的证明方法可得:
$$\cos'(x) =-\sin(x)$$
$$\tan'(x) = \sec^2(x)$$
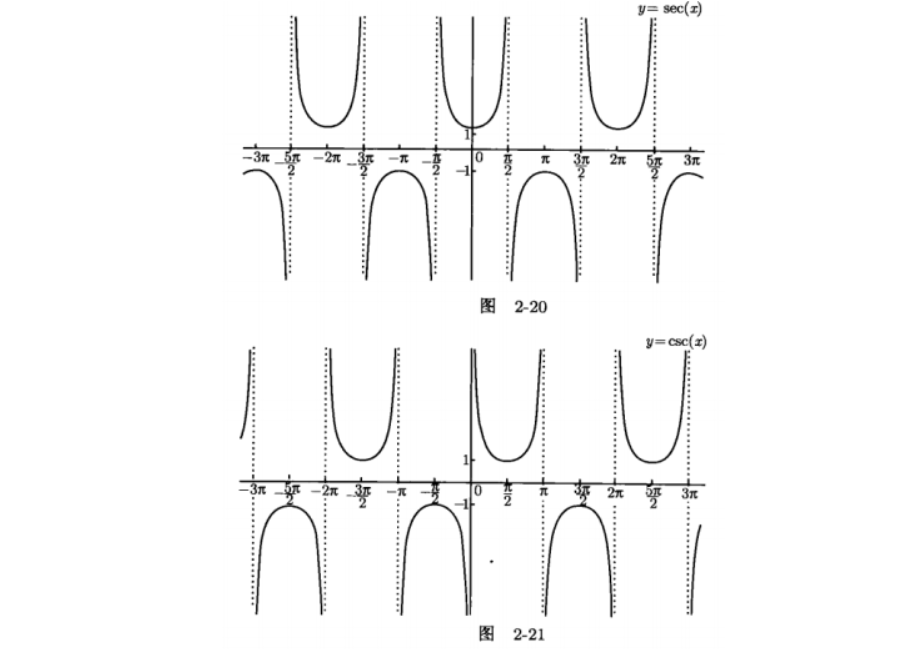
$$\sec'(x) = \sec(x)\tan(x)$$
$$\csc'(x) = -\csc(x)\cot(x) $$
$$\cot'(x) = -\csc^2(x)$$
定义:
$$e^r= \lim_{h\rightarrow 0^+}((1+h)^{1/h})^r = \lim_{n\rightarrow \infty}(1+\frac rn )^n$$
$$\ln(x) = \log _e(x)$$
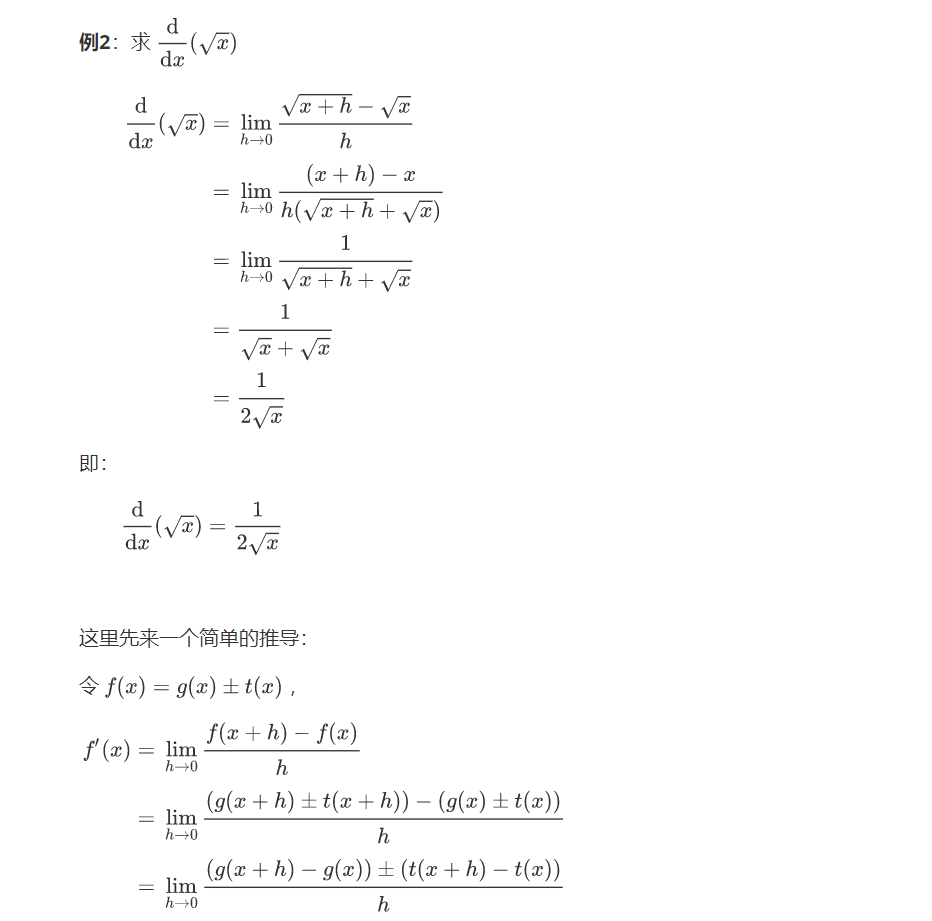
$$\ln'(x) = \frac 1 x $$
证明:
$$\ln'(x) = \lim_{d\rightarrow 0 }\frac{\ln(x+d)-\ln(x)}{d}\\=\lim_{d\rightarrow 0 }\frac{1}{d}\ln\left(\frac{x+d}{x}\right)\\=\lim_{d\rightarrow 0 }\ln\left(1+\frac{d}{x}\right)^{1/d}\\=\lim_{d\rightarrow 0 }\ln e^{1/x} \\= \frac 1 x $$
$$\log'_a(x) = \frac 1 {x\ln(b)}$$
$$\frac {\rm d} {{\rm d}x} (e^x ) = e^x $$
证明:
$$\frac{{\rm d}(\ln(x))}{{\rm d}y} = \frac{1}{y}$$
$$\frac{{\rm d}y}{{\rm d}(\ln(x))} = y$$
$$\frac{{\rm d}(e^{\ln(x)})}{{\rm d}(\ln(x))} = e^{\ln(x)}$$
所以
$$\frac {\rm d} {{\rm d}x} (e^x ) = e^x $$
洛必达法则



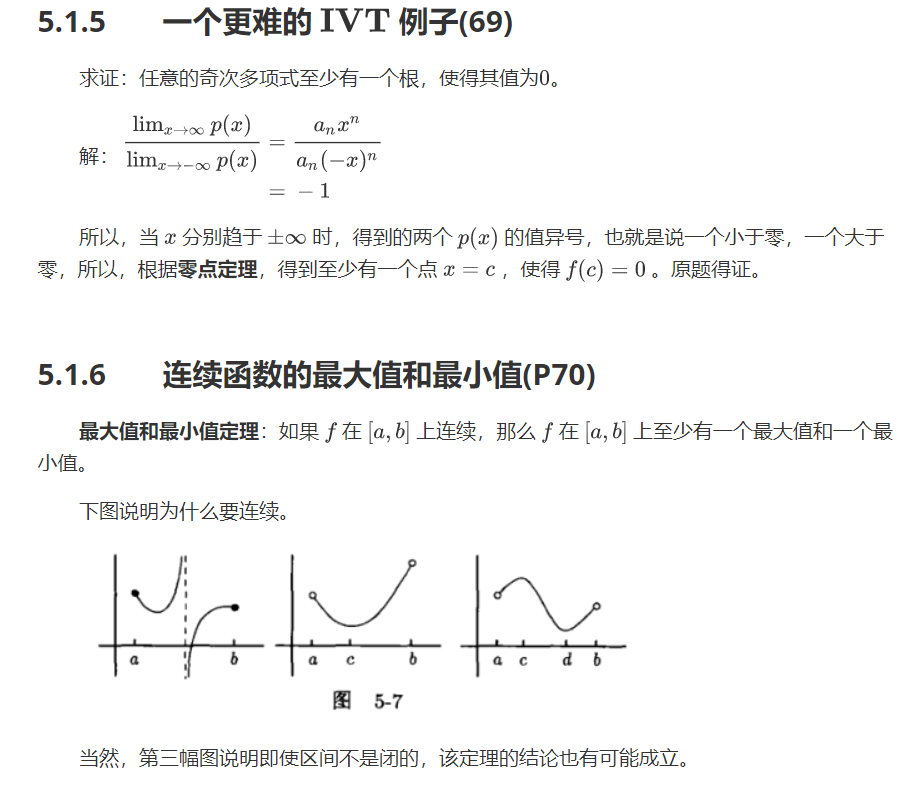
对 $f(x)$ 求不定积分就是求一个函数 $g(x)$ 满足 $g'(x)=f(x)$ 。
$$\begin{array} { l } { \frac { \mathrm { d } } { \mathrm { d } x } x ^ { a } = a x ^ { a - 1 } } \\ { \frac { \mathrm { d } } { \mathrm { d } x } \ln ( x ) = \frac { 1 } { x } } \\ { \frac { \mathrm { d } } { \mathrm { d } x } \mathrm { e } ^ { x } = \mathrm { e } ^ { x } } \\ { \frac { \mathrm { d } } { \mathrm { d } x } b ^ { x } = b ^ { x } \ln ( b ) } \\ { \frac { \mathrm { d } } { \mathrm { d } x } \sin ( x ) = \cos ( x ) } \\ { \frac { \mathrm { d } } { \mathrm { d } x } \cos ( x ) = - \sin ( x ) } \\ { \frac { \mathrm { d } } { \mathrm { d } x } \tan ( x ) = \sec ^ { 2 } ( x ) } \\ { \frac { \mathrm { d } } { \mathrm { d } x } \sec ( x ) = \sec ( x ) \tan ( x ) } \\ { \frac { \mathrm { d } } { \mathrm { d } x } \cot ( x ) = - \csc ^ { 2 } ( x ) } \\ { \frac { \mathrm { d } } { \mathrm { d } x } \csc ( x ) = - \csc ( x ) \cot ( x ) } \\ { \frac { \mathrm { d } x } { \mathrm { d } x } \sin ^ { - 1 } ( x ) = \frac { 1 } { \sqrt { 1 - x ^ { 2 } } } } \\ { \frac { \mathrm { d } x } { \mathrm { d } x } \tan ^ { - 1 } ( x ) = \frac { 1 } { 1 + x ^ { 2 } } } \\ { \frac { \mathrm { d } x } { \mathrm { d } x } \sec ^ { - 1 } ( x ) = \frac { 1 } { | x | \sqrt { x ^ { 2 } - 1 } } } \\ { \frac { \mathrm { d } x } { \mathrm { d } x } \sinh ( x ) = \cosh ( x ) } \\ { \frac { \mathrm { d } x } { \mathrm { d } x } \cosh ( x ) = \sinh ( x ) } \\ { \int x ^ { a } \mathrm { d } x = \frac { x ^ { a + 1 } } { a + 1 } + C \quad ( \text { if } a \neq - 1 ) } \\ { \int \frac { 1 } { x } \mathrm { d } x = \ln | x | + C } \\ { \int \mathrm { e } ^ { x } \mathrm { d } x = \mathrm { e } ^ { x } + C } \\ { \int b ^ { x } \mathrm { d } x = \frac { b ^ { x } } { \ln ( b ) } + C } \\ {\int \cos ( x ) \mathrm { d } x = \sin ( x ) + C } \\ { \int \sin ( x ) \mathrm { d } x = - \cos ( x ) + C } \\ { \int \sec ^ { 2 } ( x ) \mathrm { d } x = \tan ( x ) + C } \\ { \int \sec ( x ) \tan ( x ) \mathrm { d } x = \sec ( x ) + C } \\ { \int \csc ^ { 2 } ( x ) \mathrm { d } x = - \cot ( x ) + C }\\{ \int \csc ( x ) \cot ( x ) \mathrm { d } x = - \csc ( x ) + C } \\ { \int \frac { 1 } { \sqrt { 1 - x ^ { 2 } } } \mathrm { d } x = \sin ^ { - 1 } ( x ) + C } \\ { \int \frac { 1 } { 1 + x ^ { 2 } } \mathrm { d } x = \tan ^ { - 1 } ( x ) + C } \\ { \int \frac { 1 } { | x | \sqrt { x ^ { 2 } - 1 } } \mathrm { d } x = \sec ^ { - 1 } ( x ) + C } \\ { \int \cosh ( x ) \mathrm { d } x = \sinh ( x ) + C } \end{array}$$
一个函数 $f$ 的麦克劳林级数定义为:
$$f(x) = \sum_{n=0}^{\infty} \frac{f^{(n)}(a)}{n!}(x-a)^n$$
效果就是把它转成多项式。
$$\mathrm { e } ^ { x } = \sum _ { n = 0 } ^ { \infty } \frac { x ^ { n } } { n ! } = 1 + x + \frac { x ^ { 2 } } { 2 ! } + \frac { x ^ { 3 } } { 3 ! } + \cdots$$
$$\sin ( x ) = \sum _ { n = 0 } ^ { \infty } \frac { ( - 1 ) ^ { n } x ^ { 2 n + 1 } } { ( 2 n + 1 ) ! } = x - \frac { x ^ { 3 } } { 3 ! } + \frac { x ^ { 5 } } { 5 ! } - \frac { x ^ { 7 } } { 7 ! } + \cdots$$
$$\cos ( x ) = \sum _ { n = 0 } ^ { \infty } \frac { ( - 1 ) ^ { n } x ^ { 2 n } } { ( 2 n ) ! } = 1 - \frac { x ^ { 2 } } { 2 ! } + \frac { x ^ { 4 } } { 4 ! } - \frac { x ^ { 6 } } { 6 ! } + \cdots$$
$$\frac { 1 } { 1 - x } = \sum _ { n = 0 } ^ { \infty } x ^ { n } = 1 + x + x ^ { 2 } + x ^ { 3 } + \cdots$$
$$ { \ln ( 1 + x ) = \sum _ { n = 1 } ^ { \infty } - \frac { ( - 1 ) ^ { n } x ^ { n } } { n } = x - \frac { x ^ { 2 } } { 2 } + \frac { x ^ { 3 } } { 3 } - \frac { x ^ { 4 } } { 4 } + \cdots } $$
$$ { \ln ( 1 - x ) = \sum _ { n = 1 } ^ { \infty } - \frac { x ^ { n } } { n } = - x - \frac { x ^ { 2 } } { 2 } - \frac { x ^ { 3 } } { 3 } - \frac { x ^ { 4 } } { 4 } - \cdots } $$
$$\tan ^ { - 1 } ( x ) = x - \frac { x ^ { 3 } } { 3 } + \frac { x ^ { 5 } } { 5 } - \frac { x ^ { 7 } } { 7 } + \cdots = \sum _ { n = 0 } ^ { \infty } \frac { ( - 1 ) ^ { n } x ^ { 2 n + 1 } } { 2 n + 1 }$$
有什么用?
一个例子:
证明欧拉等式:
$$\mathrm { e } ^ { i \theta } = \cos ( \theta ) + i \sin ( \theta )$$
证明:
$$\begin{aligned} \mathrm { e } ^ { i \theta } & = 1 + ( i \theta ) + \frac { ( i \theta ) ^ { 2 } } { 2 ! } + \frac { ( i \theta ) ^ { 3 } } { 3 ! } + \frac { ( i \theta ) ^ { 4 } } { 4 ! } + \frac { ( i \theta ) ^ { 5 } } { 5 ! } + \frac { ( i \theta ) ^ { 6 } } { 6 ! } + \frac { ( i \theta ) ^ { 7 } } { 7 ! } + \cdots \\ & = 1 + i \theta - \frac { \theta ^ { 2 } } { 2 ! } - i \frac { \theta ^ { 4 } } { 3 ! } + \frac { \theta ^ { 4 } } { 4 ! } + i \frac { \theta ^ { 5 } } { 5 ! } - \frac { \theta ^ { 7 } } { 6 ! } - i \frac { \theta ^ { 7 } } { 7 ! } + \cdots \end{aligned}$$
我们把他们的实部和虚部分开,可以得到:
实部:
$$1 - \frac { \theta ^ { 2 } } { 2 ! } + \frac { \theta ^ { 4 } } { 4 ! } - \frac { \theta ^ { 6 } } { 6 ! } + \cdots = \cos ( \theta )$$
虚部:
$$\theta - \frac { \theta ^ { 3 } } { 3 ! } + \frac { \theta ^ { 5 } } { 5 ! } - \frac { \theta ^ { 7 } } { 7 ! } + \cdots = \sin ( \theta )$$
证完了。










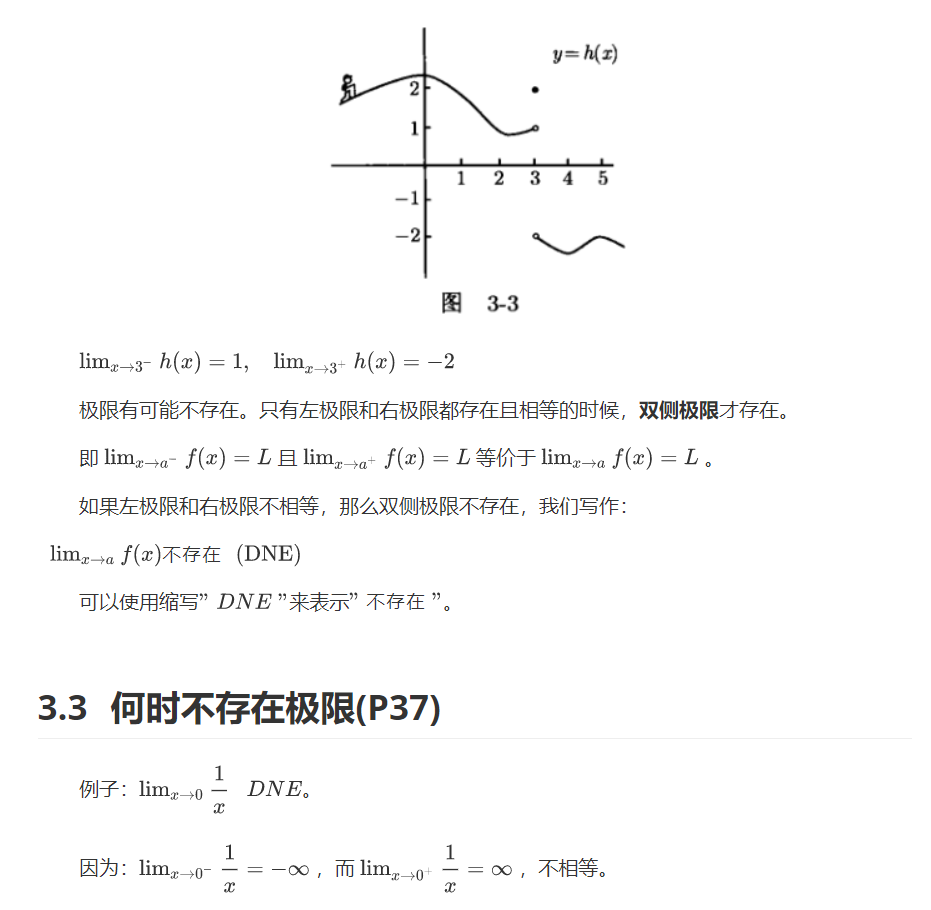
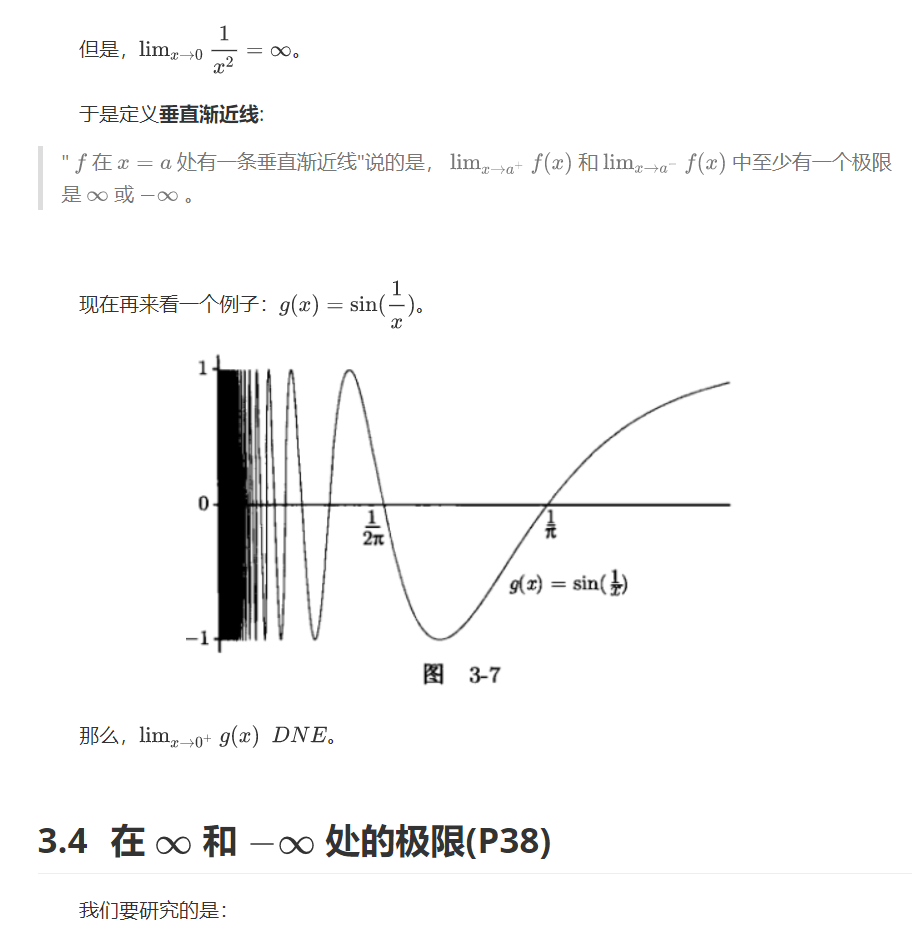
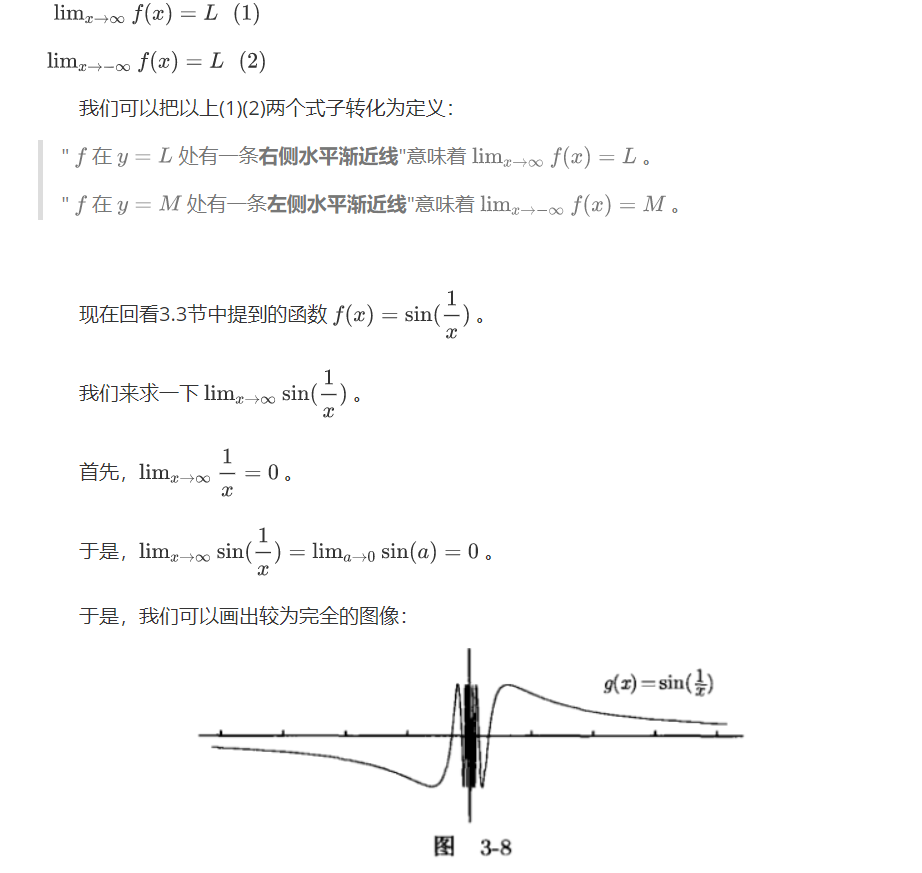
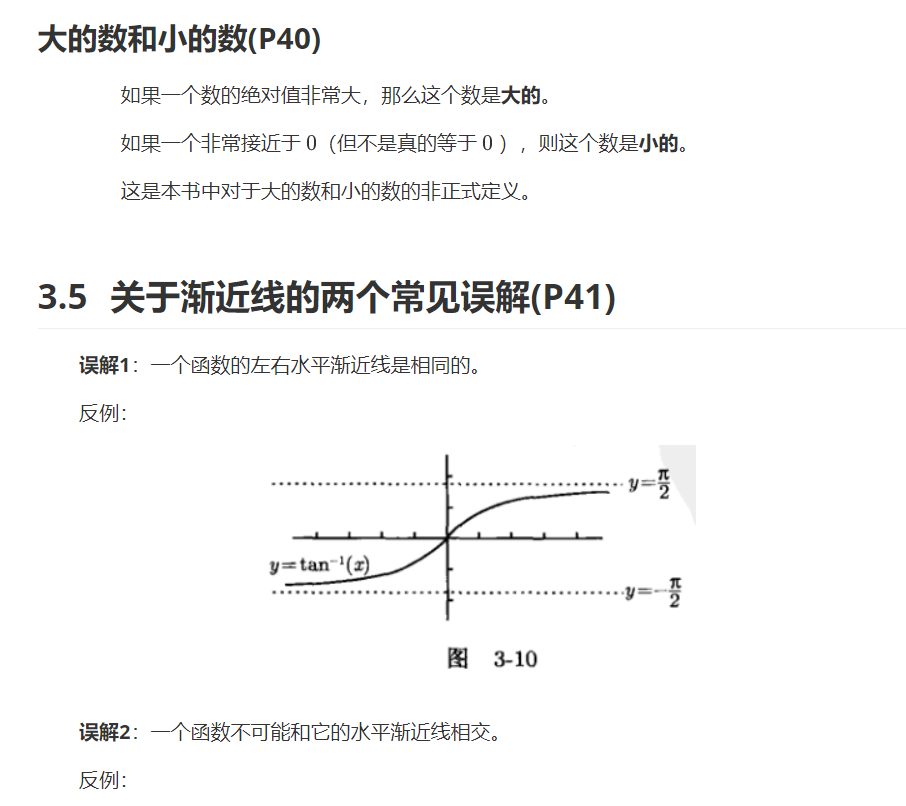



















普林斯顿微积分读本 大纲与重点 (by zzd)的更多相关文章
- 图灵数学·统计学丛书.PDF(53本全)
图灵数学·统计学丛书01-概率论及其应用(第1卷·第3版)-[美]William.Feller-人民邮电出版社.pdf 图灵数学·统计学丛书01-金融数学:衍生产品定价引论-[英]M·巴克斯特& ...
- 高斯消元法(Gauss Elimination)【超详解&模板】
高斯消元法,是线性代数中的一个算法,可用来求解线性方程组,并可以求出矩阵的秩,以及求出可逆方阵的逆矩阵.高斯消元法的原理是:若用初等行变换将增广矩阵 化为 ,则AX = B与CX = D是同解方程组. ...
- [转]RPA流程自动化-Blueprism认证考试介绍
本文转自:https://www.cnblogs.com/digod/p/9190186.html RPA流程自动化-Blueprism认证考试介绍 接触RPA有一段时间了,几种RPA相关工具也都试用 ...
- RPA流程自动化-Blueprism认证考试介绍
RPA流程自动化-Blueprism认证考试介绍 接触RPA有一段时间了,几种RPA相关工具也都试用过,BluePrism是RPA工具的一种,今天跟大家分享考Blueprism的一些经验. RPA(R ...
- Of efficiency and methodology
There are only too many articles and books which pertains to the discussion of efficiency and method ...
- Java面试大纲-java面试该做哪些准备,java开发达到这样的水平可以涨工资
Java培训结束,面临的就是毕业找工作.在找工作时,就要针对性地做充分的面试准备.准备不充分的面试,完全是浪费时间,更是对自己的不负责. 上海尚学堂Java培训整理出Java面试大纲,其中大部分都是面 ...
- 【OCP、OCM、高可用等】小麦苗课堂网络班招生简章(从入门到专家)--课程大纲
[OCP.OCM.高可用等]小麦苗课堂网络班招生简章(从入门到专家)--课程大纲 小麦苗信息 我的个人信息 网名:小麦苗 QQ:646634621 QQ群:618766405 我的博客:http:// ...
- Objective-C代码学习大纲(4)
2011-05-11 14:06 佚名 otierney 字号:T | T 本文为台湾出版的<Objective-C学习大纲>的翻译文档,系统介绍了Objective-C代码,很多名词为台 ...
- 金三银四跳槽季,Java面试题大纲
跳槽时时刻刻都在发生,但是我建议大家跳槽之前,先想清楚为什么要跳槽.切不可跟风,看到同事一个个都走了,自己也盲目的开始面试起来(期间也没有准备充分),到底是因为技术原因(影响自己的发展,偏移自己规划的 ...
随机推荐
- CodeVs 3150 (大数 + 递推)
#include<iostream> #include<cstdio> #include<cstring> #include<string> #incl ...
- mysql设计表时注意事项
说明:本文是对项目过程中的一些要求的简单汇总整理,主要是供个人本身参考... 一.表设计 1. 在创建表结构时,表名.字段需要见名知意,不采用拼音 create table `tb_abc` ( ...
- [正则表达式]匹配Unicode
一.PHP[PCRE]之Unicode PCRE支持的16进制字符编码转义符有 \x00-\xFF,或\x{num},num为任意位16进制数 但并不支持\u0000-\uFFFF这的形式 PCRE运 ...
- 查看ubuntu版本信息
参考文章:https://blog.csdn.net/qq_27818541/article/details/75207986 版本信息lsb_release -a No LSB modules ar ...
- LuoGu P1083 借教室
题目传送门 借教室这个题有两种做法,差分+前缀和或者是线段树 线段树维护区间最小值,因为能不能借教室使用是由这几天中可用教室最少的一天决定的 其实这题是个很裸的线段树维护区间最小值,但有一点需要注意, ...
- swift 实践- 10 -- UIProgressView
import UIKit class ViewController: UIViewController { override func viewDidLoad() { super.viewDidLoa ...
- EasyUI Layout 添加、删除、折叠、展开布局
<!DOCTYPE html> <html> <head> <title>吹泡泡的魚-主页</title> <link rel=&qu ...
- Java 并发类
java.util.concurrent包里 提供了一批线程安全的类 一. java.util.concurrent.atomic java.util.concurrent.atomic包里的原子处理 ...
- CentOS7+mysql(yum)
1.现在centos上默认是没有yum源的,yum安装的是 MariaDB.所以我们需要自己先配置yum源.配置yum源步骤如下: 下载yum源:wget 'https://dev.mysql.com ...
- Spring Boot的Listener机制的用法和实现原理详解
之前在介绍了在spring-boot启动过程中调用runner的原理,今天我们介绍另外一种可以实现相似功能的机制:spring-boot的Listener机制. 通过注册Listener,可以实现对于 ...
