题解报告：poj 3070 Fibonacci

Description

In the Fibonacci integer sequence, F₀ = 0, F₁ = 1, and F_n = F_{n − 1} + F_{n − 2} for n ≥ 2. For example, the first ten terms of the Fibonacci sequence are:

0, 1, 1, 2, 3, 5, 8, 13, 21, 34, …

An alternative formula for the Fibonacci sequence is

.

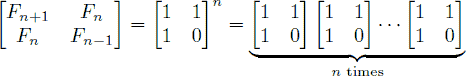Given an integer n, your goal is to compute the last 4 digits of F_n.

Input

The input test file will contain multiple test cases. Each test case consists of a single line containing n (where 0 ≤ n ≤ 1,000,000,000). The end-of-file is denoted by a single line containing the number −1.

Output

For each test case, print the last four digits of F_n. If the last four digits of F_n are all zeros, print ‘0’; otherwise, omit any leading zeros (i.e., print F_n mod 10000).

Sample Input

0
9
999999999
1000000000
-1

Sample Output

0
34
626
6875

Hint

As a reminder, matrix multiplication is associative, and the product of two 2 × 2 matrices is given by

.

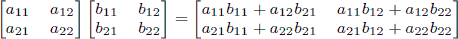Also, note that raising any 2 × 2 matrix to the 0th power gives the identity matrix:

.

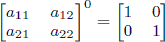解题思路：题目已经帮我们构造出矩阵行列式的关系了，这里就讲讲思路吧。通过给出的递推式我们可以推导得到题目那个n次幂的矩阵的通项公式，记那个矩阵为A，所以问题转化成求Aⁿ，很自然就想到了矩阵快速幂，没错，无论n有多大，采用矩阵快速幂的做法就是一件很简单的事情。不过如果n值超过int最大值，要改成long long类型，避免数据溢出，其余代码不变。最后取结果矩阵右上角的值即为F_n对应的结果。

AC代码：

 #include<iostream>
 #include<string.h>
 using namespace std;
 const int mod=;
 const int maxn=;//2行2列式
 struct Matrix{int m[maxn][maxn];}init;int n;
 Matrix mul(Matrix a,Matrix b){//矩阵相乘
     Matrix c;
     for(int i=;i<maxn;i++){//第一个矩阵的行
         for(int j=;j<maxn;j++){//第二个矩阵的列
             c.m[i][j]=;
             for(int k=;k<maxn;k++)
                 c.m[i][j]=(c.m[i][j]+a.m[i][k]*b.m[k][j])%mod;
         }
     }
     return c;
 }
 Matrix POW(Matrix a,int x){
     Matrix b;memset(b.m,,sizeof(b.m));
     for(int i=;i<maxn;++i)b.m[i][i]=;//单位矩阵
     while(x){
         if(x&)b=mul(b,a);
         a=mul(a,a);
         x>>=;
     }
     return b;
 }
 int main(){
     while(cin>>n&&(n!=-)){
         init.m[][]=;init.m[][]=;init.m[][]=;init.m[][]=;//初始化矩阵
         Matrix res = POW(init,n);//矩阵快速幂
         cout<<res.m[][]<<endl;//取右上角的值即可
     }
     return ;
 }