Codeforces Round #843 (Div. 2) Problem C

C. Interesting Sequence

time limit per test

1 second

memory limit per test

256 megabytes

input

standard input

output

standard output

Petya and his friend, robot Petya++, like to solve exciting math problems.One day Petya++ came up with the numbers nn and xx and wrote the following equality on the

board:

 

n & (n+1) & … & m=x,n & (n+1) & … & m=x

 

where && denotes the bitwise AND operation. Then he suggested his friend Petya find such a minimal mm (m≥nm≥n) that the equality on the board holds. Unfortunately, Petya couldn't solve this problem in his head and decided to ask for computer help. He quickly wrote a program and found the answer.Can you solve this difficult problem?

Input

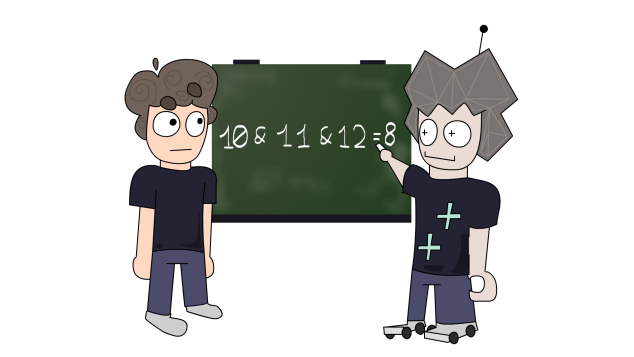
Each test contains multiple test cases. The first line contains the number of test cases t (1≤t≤2000). The description of the test cases follows.The only line of each test case contains two integers n, x (0≤n,x≤10^18).

Output

For every test case, output the smallest possible value of mm such that equality holds.If the equality does not hold for any m, print −1 instead.We can show that if the required m exists, it does not exceed 5⋅10^18.

 

Example

input

5

10 8

10 10

10 42

20 16

1000000000000000000 0

output

12
10
-1
24
1152921504606846976

Note

In the first example, 10 & 11=10, but 10 & 11 & 12=8, so the answer is 12.

In the second example, 10=10, so the answer is 10.

In the third example, we can see that the required m does not exist, so we have to print −1.

思路：

　　我们可以

按位考虑。如果

• n 在这一位上是 0 ， x 在这一位上是 0
• 选取任何的 m 都可行。
• n 在这一位上是 0 ， x 在这一位上是 1
• 不可能实现。
• n 在这一位上是 1 ， x 在这一位上是 0
• 必须等到某一个在这一位为 0 的数出现，才能满足要求。
• 设这个数最小为 k ，则可行域与 [k,+∞] 取交集。
• n 在这一位上是 1 ， x 在这一位上是 1
• m 必须在某一个在这一位为 0 的数出现之前，才能满足要求。
• 设这个数最小为 k ，则可行域与 [n,k) 取交集。

最后，如果可行域不为空，输出最小元素。时间复杂度是 Θ(log⁡max(n,x))

代码：

 1 #include<bits/stdc++.h>
 2 #define N 70
 3 using namespace std;
 4 typedef long long ll;
 5
 6 void solve()
 7 {
 8     ll n,x;
 9     scanf("%lld%lld",&n,&x);
10     bitset<64> bn(n),bx(x);
11     ll l=n,r=5e18;
12     for(int i=63;i>=0;i--)
13     {
14         if(bn[i]==0 && bx[i]==1)
15         {
16             puts("-1");
17             return;
18         }
19         if(bn[i]==0 && bx[i]==0) continue;
20         if(bn[i]==1 && bx[i]==0)
21         {
22             l=max(l,((n/(1ll<<i))+1)*(1ll<<i));
23             //二进制 1010 * 10 = 10100
24             //一个数乘 100...00 相当于左移相应的位数
25             //一个数整除 100...00 相当于把这个1右边的所有位数变成0
26         }
27         else{
28             r=min(r,((n/(1ll<<i))+1)*(1ll<<i)-1);
29         }
30     }
31
32     if(l<=r) printf("%lld\n",l);
33     else puts("-1");
34
35     return ;
36 }
37
38 int main()
39 {
40     int _;
41     cin>>_;
42     while(_--) solve();
43     return 0;
44 }

---

Noted by DanRan02

2023.1.11