Yandex.Algorithm 2011 Round 2 D. Powerful array 莫队

题目链接：点击传送

D. Powerful array

time limit per test

5 seconds

memory limit per test

256 megabytes

input

standard input

output

standard output

An array of positive integers a1, a2, ..., an is given. Let us consider its arbitrary subarray al, al + 1..., ar, where 1 ≤ l ≤ r ≤ n. For every positive integer s denote by Ks the number of occurrences of s into the subarray. We call the power of the subarray the sum of products Ks·Ks·s for every positive integer s. The sum contains only finite number of nonzero summands as the number of different values in the array is indeed finite.

You should calculate the power of t given subarrays.

Input

First line contains two integers n and t (1 ≤ n, t ≤ 200000) — the array length and the number of queries correspondingly.

Second line contains n positive integers ai (1 ≤ ai ≤ 106) — the elements of the array.

Next t lines contain two positive integers l, r (1 ≤ l ≤ r ≤ n) each — the indices of the left and the right ends of the corresponding subarray.

Output

Output t lines, the i-th line of the output should contain single positive integer — the power of the i-th query subarray.

Please, do not use %lld specificator to read or write 64-bit integers in C++. It is preferred to use cout stream (also you may use %I64d).

Examples

input

3 2
1 2 1
1 2
1 3

output

3
6

input

8 3
1 1 2 2 1 3 1 1
2 7
1 6
2 7

output

20
20
20

Note

Consider the following array (see the second sample) and its [2, 7] subarray (elements of the subarray are colored):

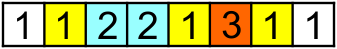Then K1 = 3, K2 = 2, K3 = 1, so the power is equal to 32·1 + 22·2 + 12·3 = 20.

莫队板子题；

#pragma comment(linker, "/STACK:1024000000,1024000000")
#include<iostream>
#include<cstdio>
#include<cmath>
#include<string>
#include<queue>
#include<algorithm>
#include<stack>
#include<cstring>
#include<vector>
#include<list>
#include<set>
#include<map>
using namespace std;
#define ll long long
#define pi (4*atan(1.0))
#define eps 1e-14
#define bug(x)  cout<<"bug"<<x<<endl;
const int N=2e5+,M=1e6+,inf=;
const ll INF=1e18+,mod=1e9+;
///   数组大小
int pos[N],k,a[N],ji[M];
struct is
{
    int l,r,p;
    bool operator <(const is &b)const
    {
        if(pos[l]==pos[b.l])
            return r<b.r;
        return pos[l]<pos[b.l];
    }
}s[N];
ll ans;
void add(int x)
{
    ans-=1LL*ji[a[x]]*ji[a[x]]*a[x];
    ji[a[x]]++;
    ans+=1LL*ji[a[x]]*ji[a[x]]*a[x];
}
void del(int x)
{
    ans-=1LL*ji[a[x]]*ji[a[x]]*a[x];
    ji[a[x]]--;
    ans+=1LL*ji[a[x]]*ji[a[x]]*a[x];
}
ll out[N];
int main()
{
    int n,q;
    scanf("%d%d",&n,&q);
    k=sqrt(n);
    for(int i=;i<=n;i++)
        scanf("%d",&a[i]),pos[i]=(i-)/k+;
    for(int i=;i<=q;i++)
        scanf("%d%d",&s[i].l,&s[i].r),s[i].p=i;
    sort(s+,s++q);
    int L=,R=;
    for(int i=;i<=q;i++)
    {
        while(L<s[i].l)
        {
            del(L);
            L++;
        }
        while(L>s[i].l)
        {
            L--;
            add(L);
        }
        while(R>s[i].r)
        {
            del(R);
            R--;
        }
        while(R<s[i].r)
        {
            R++;
            add(R);
        }
        out[s[i].p]=ans;
    }
    for(int i=;i<=q;i++)
        printf("%lld\n",out[i]);
    return ;
}