关于Java大整数是否是素数

题目描述

请编写程序，从键盘输入两个整数m，n，找出等于或大于m的前n个素数。

输入格式:

第一个整数为m，第二个整数为n；中间使用空格隔开。例如： 103 3

输出格式:

从小到大输出找到的等于或大于m的n个素数，每个一行。例如： 103 107 109

输入样例:

9223372036854775839 2

输出样例:

9223372036854775907

9223372036854775931

用到的Api：

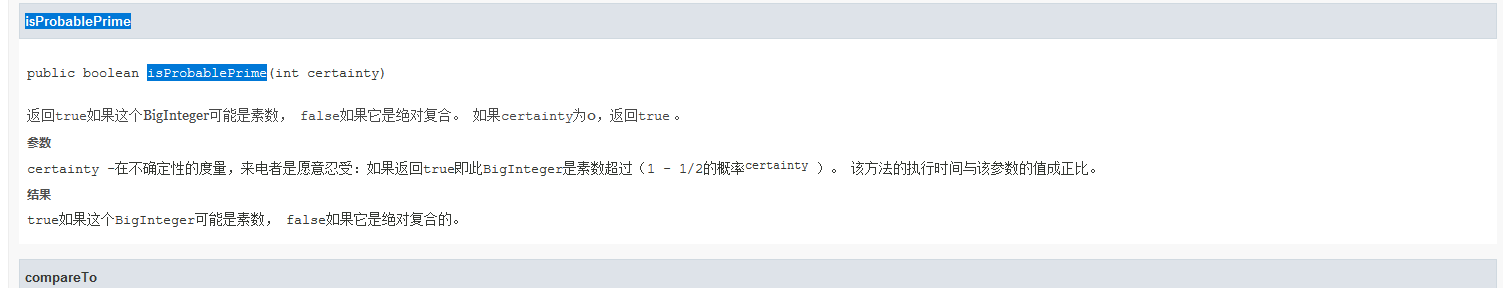
本题代码：

import java.math.BigInteger;
import java.util.ArrayList;
import java.util.List;
import java.util.Scanner;

public class Main{
 	public static void main(String args[]){
		Scanner in=new Scanner(System.in);
		String sc = in.next();
		BigInteger m = new BigInteger(sc);
		int  n = in.nextInt();
		int i=0;
		while(i<n){
			if(isPrime(m)){
				System.out.println(m);
				i++;
			}
			m=m.add(BigInteger.ONE);
		}
	}
	public static boolean isPrime(BigInteger num) {
		return num.isProbablePrime(50);
	}

	}

api的相关实现代码：

  /**
     * Returns {@code true} if this BigInteger is probably prime,
     * {@code false} if it's definitely composite.  If
     * {@code certainty} is ≤ 0, {@code true} is
     * returned.
     *
     * @param  certainty a measure of the uncertainty that the caller is
     *         willing to tolerate: if the call returns {@code true}
     *         the probability that this BigInteger is prime exceeds
     *         (1 - 1/2<sup>{@code certainty}</sup>).  The execution time of
     *         this method is proportional to the value of this parameter.
     * @return {@code true} if this BigInteger is probably prime,
     *         {@code false} if it's definitely composite.
     */
    public boolean isProbablePrime(int certainty) {
        if (certainty <= 0)
            return true;
        BigInteger w = this.abs();
        if (w.equals(TWO))
            return true;
        if (!w.testBit(0) || w.equals(ONE))
            return false;

        return w.primeToCertainty(certainty, null);
    }

    // Single Bit Operations

    /**
     * Returns {@code true} if and only if the designated bit is set.
     * (Computes {@code ((this & (1<<n)) != 0)}.)
     *
     * @param  n index of bit to test.
     * @return {@code true} if and only if the designated bit is set.
     * @throws ArithmeticException {@code n} is negative.
     */
    public boolean testBit(int n) {
        if (n < 0)
            throw new ArithmeticException("Negative bit address");

        return (getInt(n >>> 5) & (1 << (n & 31))) != 0;
    }

    /**
     * Returns {@code true} if this BigInteger is probably prime,
     * {@code false} if it's definitely composite.
     *
     * This method assumes bitLength > 2.
     *
     * @param  certainty a measure of the uncertainty that the caller is
     *         willing to tolerate: if the call returns {@code true}
     *         the probability that this BigInteger is prime exceeds
     *         {@code (1 - 1/2<sup>certainty</sup>)}.  The execution time of
     *         this method is proportional to the value of this parameter.
     * @return {@code true} if this BigInteger is probably prime,
     *         {@code false} if it's definitely composite.
     */
    boolean primeToCertainty(int certainty, Random random) {
        int rounds = 0;
        int n = (Math.min(certainty, Integer.MAX_VALUE-1)+1)/2;

        // The relationship between the certainty and the number of rounds
        // we perform is given in the draft standard ANSI X9.80, "PRIME
        // NUMBER GENERATION, PRIMALITY TESTING, AND PRIMALITY CERTIFICATES".
        int sizeInBits = this.bitLength();
        if (sizeInBits < 100) {
            rounds = 50;
            rounds = n < rounds ? n : rounds;
            return passesMillerRabin(rounds, random);
        }

        if (sizeInBits < 256) {
            rounds = 27;
        } else if (sizeInBits < 512) {
            rounds = 15;
        } else if (sizeInBits < 768) {
            rounds = 8;
        } else if (sizeInBits < 1024) {
            rounds = 4;
        } else {
            rounds = 2;
        }
        rounds = n < rounds ? n : rounds;

        return passesMillerRabin(rounds, random) && passesLucasLehmer();
    }

    /**
     * Returns true iff this BigInteger passes the specified number of
     * Miller-Rabin tests. This test is taken from the DSA spec (NIST FIPS
     * 186-2).
     *
     * The following assumptions are made:
     * This BigInteger is a positive, odd number greater than 2.
     * iterations<=50.
     */
    private boolean passesMillerRabin(int iterations, Random rnd) {
        // Find a and m such that m is odd and this == 1 + 2**a * m
        BigInteger thisMinusOne = this.subtract(ONE);
        BigInteger m = thisMinusOne;
        int a = m.getLowestSetBit();
        m = m.shiftRight(a);

        // Do the tests
        if (rnd == null) {
            rnd = ThreadLocalRandom.current();
        }
        for (int i=0; i < iterations; i++) {
            // Generate a uniform random on (1, this)
            BigInteger b;
            do {
                b = new BigInteger(this.bitLength(), rnd);
            } while (b.compareTo(ONE) <= 0 || b.compareTo(this) >= 0);

            int j = 0;
            BigInteger z = b.modPow(m, this);
            while (!((j == 0 && z.equals(ONE)) || z.equals(thisMinusOne))) {
                if (j > 0 && z.equals(ONE) || ++j == a)
                    return false;
                z = z.modPow(TWO, this);
            }
        }
        return true;
    }

 /**
     * Returns true iff this BigInteger is a Lucas-Lehmer probable prime.
     *
     * The following assumptions are made:
     * This BigInteger is a positive, odd number.
     */
    private boolean passesLucasLehmer() {
        BigInteger thisPlusOne = this.add(ONE);

        // Step 1
        int d = 5;
        while (jacobiSymbol(d, this) != -1) {
            // 5, -7, 9, -11, ...
            d = (d < 0) ? Math.abs(d)+2 : -(d+2);
        }

        // Step 2
        BigInteger u = lucasLehmerSequence(d, thisPlusOne, this);

        // Step 3
        return u.mod(this).equals(ZERO);
    }