[14th CSMO Day 1 <平面几何>]
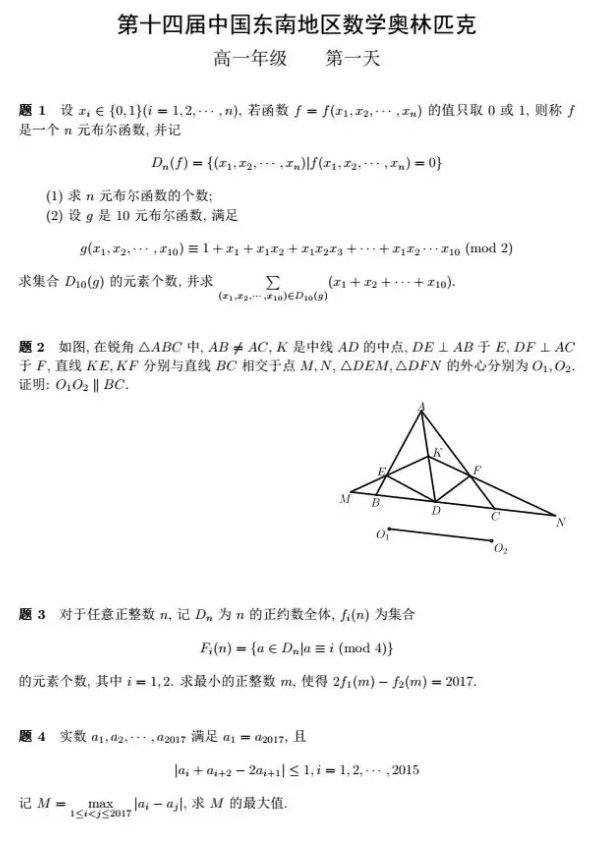
关于LowBee苦思冥想的结果(仅供参考):
[14th CSMO Day 1 <平面几何>]的更多相关文章
- 14TH本周工作量及进度统计
14TH本周工作量及进度统计 本周psp: C(类别) C(内容) S(开始时间) ST(结束时间) I(中断时间) T(实际时间) 活动 本周会议 1 ...
- We will be discontinuing the Nitrous Development Platform and Cloud IDE on November 14th, 2016.
我表示我很难过 Nitrous We will be discontinuing the Nitrous Development Platform and Cloud IDE on November ...
- HDU 4793 2013 Changsha Regional Collision[简单的平面几何]
圆形奖章给定半径的半径和圆形区域.另一个硬币的半径,然后在桌面上平稳.给定硬币的速(的大小和方向,vx,vy)和坐标(奖牌同心圆形区域,圆和心脏为源),Q币在一个圆形区域和多少下滑(不管是什么圆形区域 ...
- The 14th tip of DB Query Analyzer
The 14th tip of DB Query Analyzer Ma Genfeng (Guangdong Unitoll Services incorporated, Guangzhou 5 ...
- May 14th 2017 Week 20th Sunday
A smooth sea never made a skillful mariner. 平静的海洋练不出熟练的水手. A smooth sea never made a skillful marine ...
- ACM学习历程—HDU5476 Explore Track of Point(平面几何)(2015上海网赛09题)
Problem Description In Geometry, the problem of track is very interesting. Because in some cases, th ...
- ACM/ICPC 之 平面几何-两直线关系(POJ 1269)
题意:给定四点的坐标(x,y),分别确定两直线,求出其交点,若重合or平行则输出相应信息 用四个点的坐标算出直线通式(ax+by+c=0)中的a,b,c,然后利用a,b,c计算出交点坐标(其他公式不够 ...
- October 14th 2016 Week 42nd Friday
Who am I? Coming October 18, 2016! 我是谁?2016.10.18 拭目以待! Don't worry. You will be a wow. Don't worry. ...
- August 14th, Week 34th Sunday, 2016
To live is to function, that is all there is in living. 活着就要发挥作用,这就是生活的全部内容. I often joke that my dr ...
随机推荐
- Golang闭包的坑
team leader 发现一个Golang程序的bug,是由不正确使用闭包引起.记载一下,以作备忘. 猜猜一下程序的结果: import ( "fmt" "time&q ...
- Babel编译:动态计算的属性名
ES2015允许使用表达式作为属性名. 编译前: const HELLO = 'hello'; let dog = { [HELLO](){ console.log('hello'); } } 编译后 ...
- 【Unity Shader】---CG标准函数库
1.CG标准函数库 和C的标准函数库类似,CG也提供了一系列的内建函数库,这些函数用于计算数学上的通用计算或算法(如纹理映射).例如:求取入射光线的反射光线用Reflect函数,求折射光线用Refla ...
- Cors 跨域 共享
CORS是一个W3C标准,全称是"跨域资源共享"(Cross-origin resource sharing). 它允许浏览器向跨源服务器,发出XMLHttpRequest请求,从 ...
- Implement Queue using Stacks(用两个栈实现队列)
来源:https://leetcode.com/problems/implement-queue-using-stacks Implement the following operations of ...
- 扩展欧几里得算法详解(exgcd)
一.前言 本博客适合已经学会欧几里得算法的人食用~~~ 二.扩展欧几里得算法 为了更好的理解扩展欧几里得算法,首先你要知道一个叫做贝祖定理的玄学定理: 即如果a.b是整数,那么一定存在整数x.y使得$ ...
- 持续集成工具——Jenkins
一.jenkins简介 1.持续集成工具 2.基于JAVA环境 二.环境搭建 1.安装JDK 2.安装配置git 3.安装配置tomcat Tomcat是针对Java的一个开源中间件服务器(容器),基 ...
- [AtCoder ARC076] F Exhausted?
霍尔定理 + 线段树? 咱学学霍尔定理... 霍尔定理和二分图完美匹配有关,具体而言,就是定义了二分图存在完美匹配的充要条件: 不妨设当前二分图左端集合为 X ,右端集合为 Y ,X 与 Y 之间的边 ...
- 3486 ( Interviewe )RMQ
Problem Description YaoYao has a company and he wants to employ m people recently. Since his company ...
- 【JAVA】Java 异常中e的getMessage()和toString()方法的异同
参考链接 CSDN: Java 异常中e的getMessage()和toString()方法的异同 示例代码1: public class TestInfo { private static ...
