poj-----(2528)Mayor's posters(线段树区间更新及区间统计+离散化)

Mayor's posters

Time Limit: 1000MS		Memory Limit: 65536K
Total Submissions: 43507		Accepted: 12693

Description

The citizens of Bytetown, AB, could not stand that the candidates in the mayoral election campaign have been placing their electoral posters at all places at their whim. The city council has finally decided to build an electoral wall for placing the posters and introduce the following rules:

• Every candidate can place exactly one poster on the wall.
• All posters are of the same height equal to the height of
  the wall; the width of a poster can be any integer number of bytes (byte
  is the unit of length in Bytetown).
• The wall is divided into segments and the width of each segment is one byte.
• Each poster must completely cover a contiguous number of wall segments.

They have built a wall 10000000 bytes long (such that there is
enough place for all candidates). When the electoral campaign was
restarted, the candidates were placing their posters on the wall and
their posters differed widely in width. Moreover, the candidates started
placing their posters on wall segments already occupied by other
posters. Everyone in Bytetown was curious whose posters will be visible
(entirely or in part) on the last day before elections.

Your task is to find the number of visible posters when all the
posters are placed given the information about posters' size, their
place and order of placement on the electoral wall.

Input

The
first line of input contains a number c giving the number of cases that
follow. The first line of data for a single case contains number 1 <=
n <= 10000. The subsequent n lines describe the posters in the order
in which they were placed. The i-th line among the n lines contains two
integer numbers l_i and ri which are the number of the wall
segment occupied by the left end and the right end of the i-th poster,
respectively. We know that for each 1 <= i <= n, 1 <= l_i <= ri <= 10000000. After the i-th poster is placed, it entirely covers all wall segments numbered l_i, l_i+1 ,... , ri.

Output

For each input data set print the number of visible posters after all the posters are placed.

The picture below illustrates the case of the sample input.

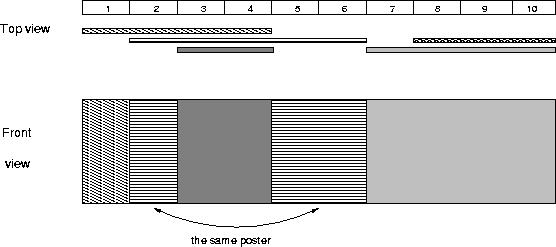
Sample Input

1
5
1 4
2 6
8 10
3 4
7 10

Sample Output

4

Source

关于区间离散的一些知识：

通俗点说，离散化就是压缩区间，使原有的长区间映射到新的短区间，但是区间压缩前后的覆盖关系不变。举个例子：

有一条1到10的数轴（长度为9），给定4个区间[2,4] [3,6] [8,10] [6,9]，覆盖关系就是后者覆盖前者，每个区间染色依次为 1 2 3 4。

现在我们抽取这4个区间的8个端点，2 4 3 6 8 10 6 9

然后删除相同的端点，这里相同的端点为6，则剩下2 4 3 6 8 10 9

对其升序排序，得2 3 4 6 8 9 10

然后建立映射

2     3     4     6     8     9   10

↓     ↓      ↓     ↓     ↓     ↓     ↓

1     2     3     4     5     6     7

那么新的4个区间为 [1,3] [2,4] [5,7] [4,6]，覆盖关系没有被改变。新数轴为1到7，即原数轴的长度从9压缩到6，显然构造[1,7]的线段树比构造[1,10]的线段树更省空间，搜索也更快，但是求解的结果却是一致的。

离散化时有一点必须要注意的，就是必须先剔除相同端点后再排序，这样可以减少参与排序元素的个数，节省时间。

代码：

 /*poj 2528 线段树+离散化*/
 //#define LOCAL
 #include<stdio.h>
 #include<string.h>
 #include<stdlib.h>
 #include<iostream>
 #include<algorithm>

 #define MAXN 10000010
 #define maxn 10005
 using namespace std;

 struct node
 {
     int st;
     int en;
 }ss[maxn];

 int lis[maxn<<];  //离散化素组
 int hash[MAXN];  //运用哈希表
 int ans;
 int vis[maxn];

 struct post
 {
     int lef,rig;
     int mid(){
       return lef+((rig-lef)>>);
     }
   int id;  //颜色种类
   int type; //用于延迟
 }poster[maxn<<];

 void build_seg(int left,int right,int pos)
 {
     poster[pos].lef=left;
     poster[pos].rig=right;
     poster[pos].id=;
     poster[pos].type=;
     if(left==right)  return ;
     int mid=poster[pos].mid();
     build_seg(left,mid,pos<<);
     build_seg(mid+,right,pos<<|);
 }

 void Update(int left,int right,int pos,int id)
 {
     if(poster[pos].lef>=left&&poster[pos].rig<=right)
     {
       poster[pos].id=id;
       poster[pos].type=id;
       return ;
     }
     if(poster[pos].type&&poster[pos].lef!=poster[pos].rig)
     {
       //向下更新一次
       poster[pos<<].type=poster[pos<<|].type=poster[pos].type;
       poster[pos<<].id=poster[pos<<|].id=poster[pos].id;
       poster[pos].type=;
     }
     int mid=poster[pos].mid();
     if(mid>=left)
         Update(left,right,pos<<,id);
     if(mid<right)
         Update(left,right,pos<<|,id);
      if(poster[pos].lef!=poster[pos].rig)
      {
       if(poster[pos<<].id==poster[pos<<|].id)
           poster[pos].id=poster[pos<<].id;
       else
          poster[pos].id=;  //说明有多种可能，需要再向下查询统计
      }
 }

 void query(int left,int right,int pos)   //进行统计
 {
     if(poster[pos].lef<left||poster[pos].rig>right)
          return ;
     if(poster[pos].id)
     {
       if(!vis[poster[pos].id])
       {
        ans++;
        vis[poster[pos].id]=true;
       }
       return;
     }
     if(poster[pos].lef!=poster[pos].rig){
      query(left,right,pos<<);
      query(left,right,pos<<|);
     }
 }

 int main()
 {
  #ifdef LOCAL
    freopen("test.in","r",stdin);
  #endif
   int cas,n;
   scanf("%d",&cas);
   while(cas--)
   {
       scanf("%d",&n);
       int k=;
       memset(hash,,sizeof(hash));
     memset(vis,,sizeof(vis)); //初始化为0表示都没有访问过
       for(int i=;i<n;i++)
     {
       scanf("%d %d",&ss[i].st,&ss[i].en);
         lis[k++]=ss[i].st;
         lis[k++]=ss[i].en;
     }
     sort(lis,lis+k);  //升序
     int j=;
     for(int i=;i<k;i++)
     {
        if(hash[lis[i]]==)
           hash[lis[i]]=++j; //编号从1起
     }
      build_seg(,j,);
     for(int i=;i<n;i++){
        Update(hash[ss[i].st],hash[ss[i].en],,i+);
     }
     ans=;
     query(,j,);
     printf("%d\n",ans);
   }
   return ;
 }