UVA11149 Power of Matrix —— 矩阵倍增、矩阵快速幂

题目链接：https://vjudge.net/problem/UVA-11149

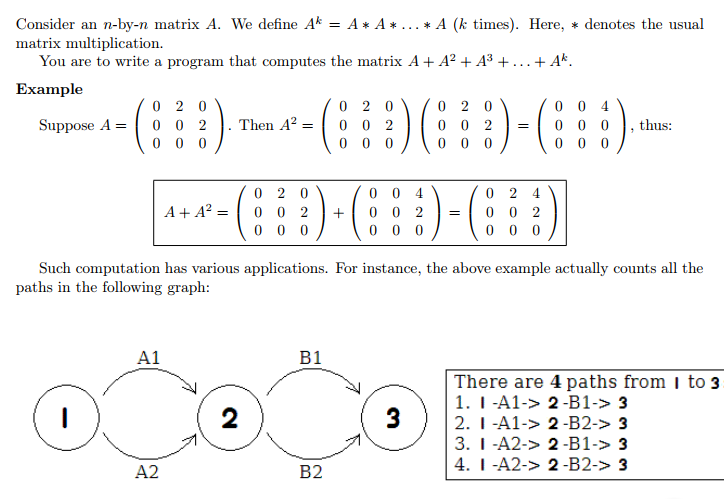
题意：

给出矩阵A，求出A^1 + A^2 …… + A^k 。

题解：

1.可知：A^1 + A^2 …… + A^k = （1+A^k/2）*（A^1 + A^2 …… + A^k/2）+ （k%2?A^k：0）。

2.根据上述式子，可二分对其求解。

代码如下：

 #include <iostream>
 #include <cstdio>
 #include <cstring>
 #include <algorithm>
 #include <vector>
 #include <cmath>
 #include <queue>
 #include <stack>
 #include <map>
 #include <string>
 #include <set>
 using namespace std;
 typedef long long LL;
 const int INF = 2e9;
 const LL LNF = 9e18;
 //const int MOD = 1e9+7;
 const int MAXN = 1e6+;
 
 const int MOD = ;
 int Size;
 struct MA
 {
     LL mat[][];
     void init()
     {
         for(int i = ; i<Size; i++)
         for(int j = ; j<Size; j++)
             mat[i][j] = (i==j);
     }
 };
 
 MA mul(MA x, MA y)
 {
     MA ret;
     memset(ret.mat, , sizeof(ret.mat));
     for(int i = ; i<Size; i++)
     for(int j = ; j<Size; j++)
     for(int k = ; k<Size; k++)
         ret.mat[i][j] += 1LL*x.mat[i][k]*y.mat[k][j]%MOD, ret.mat[i][j] %= MOD;;
     return ret;
 }
 
 MA add(MA x, MA y)
 {
     MA ret;
     memset(ret.mat, , sizeof(ret.mat));
     for(int i = ; i<Size; i++)
     for(int j = ; j<Size; j++)
         ret.mat[i][j] = x.mat[i][j]+y.mat[i][j], ret.mat[i][j] %= MOD;
     return ret;
 }
 
 MA qpow(MA x, LL y)
 {
     MA s;
     s.init();
     while(y)
     {
         if(y&) s = mul(s, x);
         x = mul(x, x);
         y >>= ;
     }
     return s;
 }
 
 MA solve(MA x, int k)
 {
     if(k==) return x;
     MA s;
     s.init();
     s = mul(add(s, qpow(x, k/)), solve(x, k/));
     if(k%) s = add(s, qpow(x, k));
     return s;
 }
 
 int main()
 {
     int n, k;
     while(scanf("%d%d", &n,&k)&&n)
     {
         MA s;
         Size = n;
         memset(s.mat, , sizeof(s.mat));
         for(int i = ; i<n; i++)
         for(int j = ; j<n; j++)
         {
             scanf("%lld", &s.mat[i][j]);
             s.mat[i][j] %= MOD;
         }
 
         s = solve(s, k);
         for(int i = ; i<n; i++)
         {
             for(int j = ; j<n; j++)
                 printf("%lld%s", s.mat[i][j], j==n-?"\n":" ");
         }
         printf("\n");
     }
 }