[LeetCode] Unique Binary Search Trees 独一无二的二叉搜索树

Given n, how many structurally unique BST's (binary search trees) that store values 1 ... n?

Example:

Input: 3
Output: 5
Explanation:
Given n = 3, there are a total of 5 unique BST's:

   1         3     3      2      1
    \       /     /      / \      \
     3     2     1      1   3      2
    /     /       \                 \
   2     1         2                 3

这道题实际上是 卡塔兰数 Catalan Numbe 的一个例子，如果对卡塔兰数不熟悉的童鞋可能真不太好做。话说其实我也是今天才知道的好嘛 -.-|||，为啥我以前都不知道捏？！为啥卡塔兰数不像斐波那契数那样人尽皆知呢，是我太孤陋寡闻么？！不过今天知道也不晚，不断的学习新的东西，这才是刷题的意义所在嘛! 好了，废话不多说了，赶紧回到题目上来吧。我们先来看当 n = 1 的情况，只能形成唯一的一棵二叉搜索树，n分别为 1,2,3 的情况如下所示：

                                            n = 

                                           n =
               /          \

                                        n =
    \       /     /      / \      \

    /     /       \                 \
                                  

就跟斐波那契数列一样，我们把 n = 0 时赋为1，因为空树也算一种二叉搜索树，那么 n = 1 时的情况可以看做是其左子树个数乘以右子树的个数，左右子树都是空树，所以1乘1还是1。那么 n = 2 时，由于1和2都可以为根，分别算出来，再把它们加起来即可。n = 2 的情况可由下面式子算出（这里的 dp[i] 表示当有i个数字能组成的 BST 的个数）：

dp[2] =  dp[0] * dp[1]　　　(1为根的情况，则左子树一定不存在，右子树可以有一个数字)

　　　　+ dp[1] * dp[0]　　  (2为根的情况，则左子树可以有一个数字，右子树一定不存在)

同理可写出 n = 3 的计算方法：

dp[3] =  dp[0] * dp[2]　　　(1为根的情况，则左子树一定不存在，右子树可以有两个数字)

　　　　+ dp[1] * dp[1]　　  (2为根的情况，则左右子树都可以各有一个数字)

　　　  + dp[2] * dp[0]　　  (3为根的情况，则左子树可以有两个数字，右子树一定不存在)

由此可以得出卡塔兰数列的递推式为：

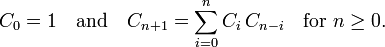
我们根据以上的分析，可以写出代码如下：

解法一：

class Solution {
public:
    int numTrees(int n) {
        vector<int> dp(n + );
        dp[] = dp[] = ;
        for (int i = ; i <= n; ++i) {
            for (int j = ; j < i; ++j) {
                dp[i] += dp[j] * dp[i - j - ];
            }
        }
        return dp[n];
    }
};

由卡特兰数的递推式还可以推导出其通项公式，即 C(2n,n)/(n+1)，表示在 2n 个数字中任取n个数的方法再除以 n+1，只要你还没有忘记高中的排列组合的知识，就不难写出下面的代码，注意在相乘的时候为了防止整型数溢出，要将结果 res 定义为长整型，参见代码如下：

解法二：

class Solution {
public:
    int numTrees(int n) {
        long res = ;
        for (int i = n + ; i <=  * n; ++i) {
            res = res * i / (i - n);
        }
        return res / (n + );
    }
};

类似题目：

Unique Binary Search Trees II

Different Ways to Add Parentheses

参考资料：

https://leetcode.com/problems/unique-binary-search-trees/

https://leetcode.com/problems/unique-binary-search-trees/discuss/31666/DP-Solution-in-6-lines-with-explanation.-F(i-n)-G(i-1)-*-G(n-i)

https://leetcode.com/problems/unique-binary-search-trees/discuss/31671/A-very-simple-and-straight-ans-based-on-MathCatalan-Number-O(N)-timesO(1)space

LeetCode All in One 题目讲解汇总(持续更新中...)