POJ1195 Mobile phones 【二维线段树】

Mobile phones

Time Limit: 5000MS		Memory Limit: 65536K
Total Submissions: 14291		Accepted: 6644

Description

Suppose that the fourth generation mobile phone base stations in the Tampere area operate as follows. The area is divided into squares. The squares form an S * S matrix with the rows and columns numbered from 0 to S-1. Each square contains a base station. The
number of active mobile phones inside a square can change because a phone is moved from a square to another or a phone is switched on or off. At times, each base station reports the change in the number of active phones to the main base station along with
the row and the column of the matrix. 



Write a program, which receives these reports and answers queries about the current total number of active mobile phones in any rectangle-shaped area. 

Input

The input is read from standard input as integers and the answers to the queries are written to standard output as integers. The input is encoded as follows. Each input comes on a separate line, and consists of one instruction integer and a number of parameter
integers according to the following table. 




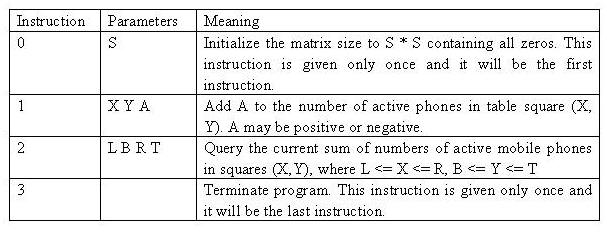
The values will always be in range, so there is no need to check them. In particular, if A is negative, it can be assumed that it will not reduce the square value below zero. The indexing starts at 0, e.g. for a table of size 4 * 4, we have 0 <= X <= 3 and
0 <= Y <= 3. 



Table size: 1 * 1 <= S * S <= 1024 * 1024 

Cell value V at any time: 0 <= V <= 32767 

Update amount: -32768 <= A <= 32767 

No of instructions in input: 3 <= U <= 60002 

Maximum number of phones in the whole table: M= 2^30 

Output

Your program should not answer anything to lines with an instruction other than 2. If the instruction is 2, then your program is expected to answer the query by writing the answer as a single line containing a single integer to standard output.

Sample Input

0 4
1 1 2 3
2 0 0 2 2
1 1 1 2
1 1 2 -1
2 1 1 2 3
3

Sample Output

3
4

附二维树状数组解法：http://blog.csdn.net/chang_mu/article/details/37739053

题意：点更新。区域查询。

题解：主要的二维线段树。（今天看了非常久才看懂...线段树扩展到二维果然没有树状数组方便，可是一旦想通，会发现思路事实上并不复杂）。

#include <stdio.h>
#define maxn 1026
#define lson l, mid, rt << 1
#define rson mid + 1, r, rt << 1 | 1

int tree[maxn * 3][maxn * 3], size;

void getSize(){ scanf("%d", &size); }

void updateY(int rootX, int pos, int val, int l, int r, int rt)
{
	tree[rootX][rt] += val;
	if(l == r) return;

	int mid = (l + r) >> 1;
	if(pos <= mid) updateY(rootX, pos, val, lson);
	else updateY(rootX, pos, val, rson);
}

void updateX(int x, int y, int val, int l, int r, int rt)
{
	//更新的任务都交给updateY
	updateY(rt, y, val, 0, size - 1, 1);
	if(l == r) return;

	int mid = (l + r) >> 1;
	if(x <= mid) updateX(x, y, val, lson);
	else updateX(x, y, val, rson);
}

void update()
{
	int x, y, val;
	scanf("%d%d%d", &x, &y, &val);
	updateX(x, y, val, 0, size - 1, 1);
}

int queryY(int rootX, int y1, int y2, int l, int r, int rt)
{
	if(l == y1 && r == y2) return tree[rootX][rt];

	int mid = (l + r) >> 1;
	if(y2 <= mid) return queryY(rootX, y1, y2, lson);
	else if(y1 > mid) return queryY(rootX, y1, y2, rson);
	else{
		return queryY(rootX, y1, mid, lson) + queryY(rootX, mid + 1, y2, rson);
	}
}

int queryX(int x1, int x2, int y1, int y2, int l, int r, int rt)
{
	if(x1 == l && x2 == r) return queryY(rt, y1, y2, 0, size - 1, 1);

	int mid = (l + r) >> 1;
	if(x2 <= mid) return queryX(x1, x2, y1, y2, lson);
	else if(x1 > mid) queryX(x1, x2, y1, y2, rson);
	else{
		return queryX(x1, mid, y1, y2, lson) + queryX(mid + 1, x2, y1, y2, rson);
	}
}

void query()
{
	int x1, y1, x2, y2;
	scanf("%d%d%d%d", &x1, &y1, &x2, &y2);

	printf("%d\n", queryX(x1, x2, y1, y2, 0, size - 1, 1));
}

void (*funArr[])() = {
	getSize, update, query
};

int main()
{
	int num;
	while(scanf("%d", &num), num != 3)
		(*funArr[num])();
	return 0;
}