codeforces 477A A. Dreamoon and Sums(数学)

题目链接：

A. Dreamoon and Sums

time limit per test

1.5 seconds

memory limit per test

256 megabytes

input

standard input

output

standard output

Dreamoon loves summing up something for no reason. One day he obtains two integers a and b occasionally. He wants to calculate the sum of all nice integers. Positive integer x is called nice if 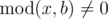 and 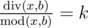, where k is some integer number in range[1, a].

By 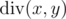 we denote the quotient of integer division of x and y. By  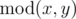we denote the remainder of integer division of x andy. You can read more about these operations here: http://goo.gl/AcsXhT.

The answer may be large, so please print its remainder modulo 1 000 000 007 (109 + 7). Can you compute it faster than Dreamoon?

Input

The single line of the input contains two integers a, b (1 ≤ a, b ≤ 107).

Output

Print a single integer representing the answer modulo 1 000 000 007 (109 + 7).

Examples

input

1 1

output

0

input

2 2

output

8

题意：

计算满足上述要求的数的和,满足要求的数x/b是x%b的k倍,k属于[1,a];

思路：

一个推公式的题,x=b*div+mod;其中div=k*mod;所以x=(b*k+1)*mod;而1<=k<=a;1<=mod<=b-1;
得到公式∑∑(b*k+1)*mod=(∑(b*k+1))*(∑mod)=b*(b-1)/2*(a+a*(a+1)/2*b);
就变成水题了;

AC代码：

#include <iostream>
#include <cstdio>
#include <cstring>
#include <algorithm>
#include <cmath>
#include <bits/stdc++.h>
#include <stack>
#include <map>

using namespace std;

#define For(i,j,n) for(int i=j;i<=n;i++)
#define mst(ss,b) memset(ss,b,sizeof(ss));

typedef  long long LL;

template<class T> void read(T&num) {
    char CH; bool F=false;
    for(CH=getchar();CH<'0'||CH>'9';F= CH=='-',CH=getchar());
    for(num=0;CH>='0'&&CH<='9';num=num*10+CH-'0',CH=getchar());
    F && (num=-num);
}
int stk[70], tp;
template<class T> inline void print(T p) {
    if(!p) { puts("0"); return; }
    while(p) stk[++ tp] = p%10, p/=10;
    while(tp) putchar(stk[tp--] + '0');
    putchar('\n');
}

const int mod=1e9+7;
const double PI=acos(-1.0);
const int inf=1e9;
const int N=1e6+20;
const int maxn=1e3+110;
const double eps=1e-12;

int main()
{
    LL a,b;
    read(a);read(b);
    LL ans=b*(b-1)/2%mod;
    ans=ans*(a+a*(a+1)/2%mod*b%mod)%mod;
    cout<<ans<<endl;

    return 0;
}