UVA - 1416 Warfare And Logistics (最短路)

Description

The army of United Nations launched a new wave of air strikes on terroristforces. The objective of the mission is to reduce enemy's logistical mobility. Each airstrike will destroy a path and therefore increase the shipping cost of the shortest pathbetween
two enemy locations. The maximal damage is always desirable.

Let's assume that there are n enemy locations connected by
m bidirectional paths,each with specific shipping cost. Enemy's total shipping cost is given as
c = 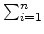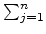path(i,
j). Here path(i, j) is the shortest path between locations
i and j. In case
i and j are not connected,
path(i, j) = L. Each air strike can only destroy one path. The total shipping cost after the strike is noted as
c'. In order to maximizedthe damage to the enemy, UN's air force try to find the maximal
c' - c.

Input

The first line ofeach input case consists ofthree integers:
n, m, and L.
1 < n100,1m1000,
1L108.
Each ofthe following m lines contains three integers:
a,b,
s, indicating length of the path between a and
b.

Output

For each case, output the total shipping cost before the air strike and the maximaltotal shipping cost after the strike. Output them in one line separated by a space.

Sample Input

4  6  1000
1  3  2
1  4  4
2  1  3
2  3  3
3  4  1
4  2  2

Sample Output

28  38

题意：给出一个n节点m条边的无向图，每条边上有一个正权，令c等于每对结点的最短路径之和，要求删除一条边后使得新的c值最大。不连通的两个点距离视为L

思路：每次求单源最短路的同一时候记录下删除边的值，然后计算最小值就是了

#include <iostream>
#include <cstdio>
#include <cstring>
#include <algorithm>
#include <vector>
#include <queue>
using namespace std;
typedef long long ll;
const int MAXN = 1010;
const int INF = 0x3f3f3f3f;

struct Edge {
	int from, to, dist;
};

struct HeapNode {
	int d, u;
	bool operator<(const HeapNode rhs) const {
		return d > rhs.d;
	}
};

struct Dijkstra {
	int n, m;
	vector<Edge> edges;
	vector<int> G[MAXN];
	bool done[MAXN];
	ll d[MAXN];
	int p[MAXN];

	void init(int n) {
		this->n = n;
		for (int i = 0; i < n; i++)
			G[i].clear();
		edges.clear();
	}

	void AddEdge(int from, int to, int dist) {
		edges.push_back((Edge){from, to, dist});
		edges.push_back((Edge){to, from, dist});
		m = edges.size();
		G[from].push_back(m-2);
		G[to].push_back(m-1);
	}

	void dijkstra(int s, int curEdge) {
		priority_queue<HeapNode> Q;
		for (int i = 0; i < n; i++)
			d[i] = INF;
		d[s] = 0;
		memset(done, 0, sizeof(done));
		memset(p, -1, sizeof(p));
		Q.push((HeapNode){0, s});
		while (!Q.empty()) {
			HeapNode x = Q.top();
			Q.pop();
			int u = x.u;
			if (done[u])
				continue;
			done[u] = true;
			for (int i = 0; i < G[u].size(); i++) {
				Edge &e = edges[G[u][i]];
				if (G[u][i] == curEdge/2*2 || G[u][i] == curEdge/2*2+1)
					continue;
				if (d[e.to] > d[u] + e.dist) {
					d[e.to] = d[u] + e.dist;
					p[e.to] = G[u][i];
					Q.push((HeapNode){d[e.to], e.to});
				}
			}
		}
	}
}arr;

int pre[MAXN];
int n, m, L;
ll s[MAXN][MAXN], A[MAXN];

int main() {
	while (scanf("%d%d%d", &n, &m, &L) != EOF) {
		int u, v, w;
		arr.init(n);
		for (int i = 0; i < m; i++) {
			scanf("%d%d%d", &u, &v, &w);
			u--, v--;
			arr.AddEdge(u, v, w);
		}
		ll ans = 0;
		memset(s, -1, sizeof(s));
		for (int i = 0; i < n; i++) {
			arr.dijkstra(i, -100);
			ll sum = 0;
			for (int j = 0; j < n; j++) {
				if (arr.d[j] == INF)
					arr.d[j] = L;
				sum += arr.d[j];
				pre[j] = arr.p[j];
			}
			A[i] = sum;
			ans += sum;
			for (int j = 0; j < n; j++) {
				if (j == i || pre[j] == -1)
					continue;
				ll tmp = 0;
				arr.dijkstra(i, pre[j]);
				for (int k = 0; k < n; k++) {
					if (arr.d[k] == INF)
						arr.d[k] = L;
					tmp += arr.d[k];
				}
				s[pre[j]/2][i] = tmp;
			}
		}
		ll Max = 0;
		for (int i = 0; i < m; i++) {
			ll sum = 0;
			for (int j = 0; j < n; j++)
				if (s[i][j] == -1)
					sum += A[j];
				else sum += s[i][j];
			Max = max(Max, sum);
		}
		printf("%lld %lld\n", ans, Max);
	}
	return 0;
}