Good Bye 2018 C. New Year and the Sphere Transmission

传送门

https://www.cnblogs.com/violet-acmer/p/10201535.html

•题意

　　$n$ 个 $people$，编号 $1,2,3,\cdots ,n$ ，按顺时针方向围城一圈；

　　初始，编号为 $1$ 的 $people$ 抱着一个球，他可以将球顺时针传给他左手边的第 $k$ 个 $people$；

　　接到球的 $people$ 依次将球传给他顺时针方向的第 $k$ 个 $people$；

　　循环进行，直到球再一次落到 $1$ 号 $people$ 手中，结束；

　　定义一个开心值 ：所有接到球的 $people$ 的编号和。

　　求所有的开心值，并按升序排列。

•题解

　　弱弱的我只能通过打表找规律%%%%%%%那些一眼看出规律的大神们　

　　$\begin{aligned} k &= 1\rightarrow 1 \\ k&= 2\rightarrow 1,3 \\ k&= 3\rightarrow 1,6 \\ k&= 4\rightarrow 1,4,10 \\ k&= 5\rightarrow 1,15 \\ k&= 6\rightarrow 1,5,9,21 \\ k&= 7\rightarrow 1,28\end{aligned}$

　　刚开始，发现，有些数的开心值只有两个，然后，把这些只有两个开心值的数列了一下，发现，全是素数。

　　不知为啥，求了一下每个数的因子个数，发现没，开心值的个数与他们的因子个数有关！！！

　　然后，在草纸上列出了前 12 项的答案，找了一下规律，哇，最后10分钟，找到了一个前10个通用的规律。

　　最后结束时刻提交,emmmmm，wa

　　然后，睡觉，哈哈哈。

　　今天，把昨天的错误数据看了一下，重新找了一下规律

　　emmmm，找到了

　　以 $k=15$ 为例：

　　　　$15$ 的因子为 $1,3,5,15$

　　　　开心值为 $1,18,35,120$

　　　　1=1;

　　　　18=1+6+11;　　　　　　　　　　　　　　　　　　　　//d=5,tot=3

　　　　35=1+4+7+10+13;　　　　　　　　　　　　　　　　   //d=3,tot=5

　　　　120=1+2+3+4+5+6+7+8+9+10+11+12+13+14+15;         //d=1,tot=15

　　发现没，开心值就是以 $15$ 的因子为公差的前 $tot$ 项和；

•Code

 #include<iostream>
 #include<cstdio>
 #include<algorithm>
 #include<cstring>
 #include<cmath>
 using namespace std;
 #define ll __int64
 #define mem(a,b) memset(a,b,sizeof(a))
 const int maxn=1e6+;

 ll n;
 ll a[maxn];
 ll res[maxn];

 int factor()//求出n的所有因子
 {
     int x=sqrt(n);
     a[]=;
     int index=;
     for(int i=;i <= x;++i)
     {
         if(n%i == )
         {
             a[++index]=i;
             if(n/i != i)
                 a[++index]=n/i;
         }
     }
     a[++index]=n;
     return index;
 }
 int main()
 {
     scanf("%d",&n);
     int t=factor();
     sort(a+,a+t+);
     for(int i=;i <= t;++i)
     {
         ll d=a[i],tot=n/d;
         ll a1=,an=a1+(tot-)*d;
         res[i]=tot*(a1+an)/;
     }
     for(int i=t;i >= ;--i)
         printf("%I64d ",res[i]);
 }

•打表找规律代码

 #include<iostream>
 #include<cstdio>
 #include<algorithm>
 #include<cstring>
 using namespace std;
 #define ll __int64
 #define mem(a,b) memset(a,b,sizeof(a))
 const ll MOD=;
 const int maxn=1e6+;

 int n;
 int a[maxn];

 int main()
 {
     for(int i=;i <= ;++i)
     {
         i=;
         int tot=;
         for(int k=;k <= i;++k)
         {
             int res=;
             int index=+k;
             printf("****\nk=%d\n",k);
             printf("");
             while(index != )
             {
                 if(index > i)
                     index %= i;
                 if(index == )
                     break;
                 res += index;
                 printf("+%d",index);
                 index += k;
             }
             printf("=%d\n",res);
             a[tot++]=res;
         }

         sort(a,a+tot);
         int t=unique(a,a+tot)-a;
         printf("\n===========\ni=%d\n",i);
         for(int j=;j < t;++j)
             printf("%d ",a[j]);
         break;
     }
 }
 //1 27 105 235 625 1275

---

•分割线2019.6.14

•感悟

　　因打表找规律而AC的题，不能当作正解，赛后补题，要花时间思考正解；

•想法

　　从编号为 $1$ 的 $people$ 开始传球，依次传给其顺时针方向的第 $k$ 个人；

　　可以肯定的是，球一定会回到 $1$ 手中，假设传了 $x$ 次，球重新回到 $1$ 手中；

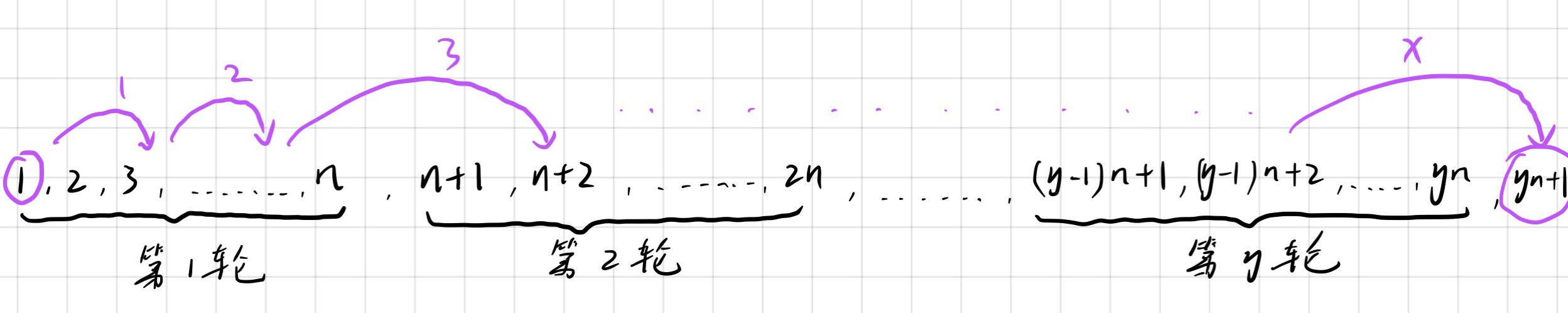
　　并假设这 $x$ 次传球，共传了 $y$ 轮，如下图所示：

　　

第 i 轮对应的序列 $(i-1)\cdot n+1,(i-1)\cdot n+2,\cdots ,i\cdot n$ 对应的 $people$ 编号为 $1,2,\cdots ,n$；

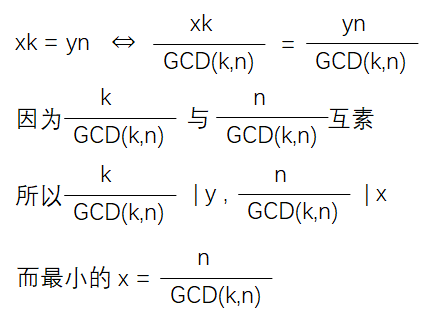
　　也就是 $1+x\cdot k = 1+y\cdot n$，即 $x\cdot k = y\cdot n$；

　　我们来分析一下这个等式可以推出什么神奇的东西：

　　

　　为什么要最小的 $x$ 呢？

　　因为只要传球期间来到 $1$ 就停止，所以需要的是最小的 $x$；

　　如果 $GCD(k,n) = k$，那么传一轮便可以来到 $1$ 处；

　　如果 $GCD(k,n) \neq k$，那么需要传多轮才能来到 $1$ 处；

　　那么下面来讨论 $GCD(k,n) \neq k$  的情况；

　　假设 $GCD(k,n) = f$，这种情况下共传球 $x=\frac{n}{f}$ 次，与 $k=f$ 的传球次数相同；

　　又因为 $f\ |\ k$，所以，这 $x$ 次传球的 $people$ 的编号一定相同；

　　所以，对于任意 $k$，传球次数和编号只与 $GCD(n,k)$ 有关系；

　　也就是只和 $n$ 的因子有关系；

　　当 $GCD(n,k) = f$ 时，接到球的 $people$ 编号为：

　　　　$1\rightarrow (1+f)\rightarrow (1+2f) \rightarrow \cdots \rightarrow (1+xf)$;

　　共传球 $x=\frac{n}{f}$ 次；

　　满足首项 $a_1=1$，末项 $a_{x+1}=n+1$,公差 $d=f$ 的等差数列；

　　前 $x$ 项和即为当前的开心值；

•Code

 #include<bits/stdc++.h>
 using namespace std;
 #define ll long long

 int n;
 vector<int >f;
 vector<ll >ans;

 void Factor(int n)///求解 n 的因子
 {
     f.clear();
     for(int i=;i*i <= n;++i)
     {
         if(n%i != )
             continue;

         f.push_back(i);
         if(n/i != i)
             f.push_back(n/i);
     }
 }
 void Solve()
 {
     Factor(n);

     for(int i=;i < f.size();++i)
     {
         ll d=f[i];
         ll x=n/f[i];
         ll s=x*(++(x-)*d)/;
         ans.push_back(s);
     }
     sort(ans.begin(),ans.end());
     for(int i=;i < ans.size();++i)
         printf("%lld ",ans[i]);
 }
 int main()
 {
     scanf("%d",&n);
     Solve();

     return ;
 }

---

应用

•题目描述

•题解

　　使得所有人都拿过球，类比于上题，也就是说求使得开心值为 $1+2+3+\cdots +n$ 的最大的 $k$；

　　那么，只有当 $GCD(n,k)=1$ 时，球才会传递给 $x=n$ 个人；

　　那么，本题就转化为求解 $GCD(n,k) = 1$ 的，并且满足 $k \le n$ 的最大的 $k$；

　　显然，$k=n-1$ 为满足条件的最大的 $k$；

•变形

　　如果限制 $k \le \frac{n}{2}$ 呢？

•分析

　　如果 $n$ 为奇数，那么 $\lfloor{ \frac{n}{2} }\rfloor$ 一定与 $n$ 互素；

　　如果 $n$ 为偶数，那么，如果 $\frac{n}{2}$ 为奇数，答案为 $\frac{n}{2}-2$；

　　反之，如果 $\frac{n}{2}$ 为偶数，那么答案为 $\frac{n}{2}-1$，因为奇数与偶数一定互素；

　　也就是说，直接判断 $\lfloor{ \frac{n}{2} }\rfloor\ ,\ \lfloor{ \frac{n}{2} }\rfloor-1\ ,\ \lfloor{ \frac{n}{2} }\rfloor-2$ 这三个数哪个与 $n$ 互素即可；