Necklace of Beads(polya计数)

Necklace of Beads

Time Limit: 1000MS		Memory Limit: 10000K
Total Submissions: 7451		Accepted: 3102

Description

Beads of red, blue or green colors are connected together into a circular necklace of n beads ( n < 24 ). If the repetitions that are produced by rotation around the center of the circular necklace or reflection to the axis of symmetry are all neglected, how many different forms of the necklace are there? 

Input

The input has several lines, and each line contains the input data n.  -1 denotes the end of the input file. 

Output

The output should contain the output data: Number of different forms, in each line correspondent to the input data.

Sample Input

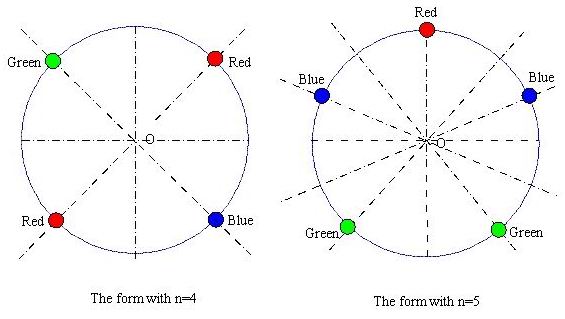
4
5
-1

Sample Output

21
39
题解：给出红，绿，蓝3种颜色 的n个珠子，求能够组成多少个不同的项链。 （旋转 和 翻转后 相同的属于同一个项链）
看了好久大神的代码还是不太理解；暂时的理解是，对于每个循环节里的元素都有k中染色方案，所以是k^c(f);
现在只需要找出所有循环节的种数就好了；当然翻转和旋转循环节是不同的，翻转和旋转均有n种；所有种数加完要除以2n

Polya定理：

(1)设G是p个对象的一个置换群，用k种颜色给这p个对象，若一种染色方案在群G的作用下变为另一种方案，则这两个方案当作是同一种方案，这样的不同染色方案数为

(2)对于N个珠子的项链，共有n种旋转置换和n种翻转置换。

对于旋转置换：每种置换的循环节数c(fi) = gcd(n,i)，(i为一次转过多少个珠子)

对于翻转置换：如果n为奇数，共有n种翻转置换，每种置换的循环节数均为c(f) = n/2 + 1;              如果n为偶数，分两种情况 <1> 从空白处穿对称轴，则轴两边各有n/2个对象，得到c(f) = n/2；

<2> 从两个对象上穿对称轴，则轴两边各有n/2-2个对象，得到c(f) = n/2 + 1。

代码：

#include<cstdio>
#include<iostream>
#include<cmath>
#include<algorithm>
#include<cstring>
#include<queue>
using namespace std;
const int INF=0x3f3f3f3f;
#define mem(x,y) memset(x,y,sizeof(x))
#define SI(x) scanf("%d",&x)
#define PI(x) printf("%d",x)
typedef long long LL;
int gcd(int a,int b){return b==?a:gcd(b,a%b);}
int main(){
    int n;
    while(~SI(n),n!=-){
        LL ans=;
        if(!n){
            puts("");continue;
        }
        for(int i=;i<=n;i++)
            ans+=pow(,gcd(i,n));
        ans+=n*(n&?pow(,n/+):(pow(,n/)/+pow(,n/+)/));
        printf("%lld\n",ans/(*n));
    }
    return ;
}