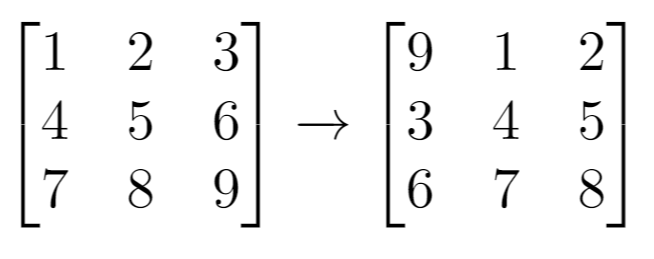【leetcode】1260. Shift 2D Grid
题目如下:
Given a 2D
gridof sizen*mand an integerk. You need to shift thegridktimes.In one shift operation:
- Element at
grid[i][j]becomes atgrid[i][j + 1].- Element at
grid[i][m - 1]becomes atgrid[i + 1][0].- Element at
grid[n - 1][m - 1]becomes atgrid[0][0].Return the 2D grid after applying shift operation
ktimes.Example 1:
Input:grid= [[1,2,3],[4,5,6],[7,8,9]], k = 1
Output: [[9,1,2],[3,4,5],[6,7,8]]Example 2:
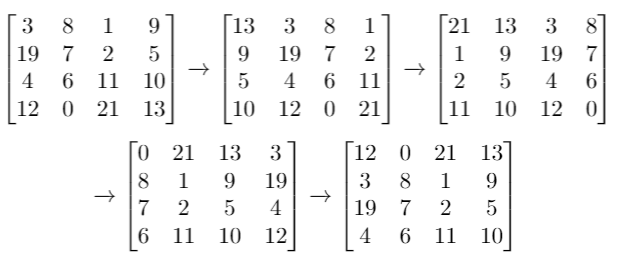
Input:grid= [[3,8,1,9],[19,7,2,5],[4,6,11,10],[12,0,21,13]], k = 4
Output: [[12,0,21,13],[3,8,1,9],[19,7,2,5],[4,6,11,10]]Example 3:
Input:grid= [[1,2,3],[4,5,6],[7,8,9]], k = 9
Output: [[1,2,3],[4,5,6],[7,8,9]]Constraints:
1 <= grid.length <= 501 <= grid[i].length <= 50-1000 <= grid[i][j] <= 10000 <= k <= 100
解题思路:记grid的行数为row,列数为col,显然经过row*col次移动后和不移动效果是一样的,所以可以首先令k = k%(row*col)。剩下的k就是每一个元素需要移动的次数,我的方法是给grid的每个元素编号,从左到右,从上到下,依次为0,1,1....row*col - 1,这样方便计算。
代码如下:
class Solution(object):
def shiftGrid(self, grid, k):
"""
:type grid: List[List[int]]
:type k: int
:rtype: List[List[int]]
"""
row = len(grid)
col = len(grid[0])
k = k%(row * col)
res = [[0] * col for _ in range(row)]
for i in range(row):
for j in range(col):
inx = (i*col + j) + k
if inx >= (row*col):inx -= row*col
newrow = inx/col
newcol = inx%col
res[newrow][newcol] = grid[i][j]
return res
【leetcode】1260. Shift 2D Grid的更多相关文章
- 【leetcode】Search a 2D Matrix
Search a 2D Matrix Write an efficient algorithm that searches for a value in an m x n matrix. This m ...
- 【leetcode】 Search a 2D Matrix (easy)
Write an efficient algorithm that searches for a value in an m x n matrix. This matrix has the follo ...
- 【LeetCode】764. Largest Plus Sign 解题报告(Python)
[LeetCode]764. Largest Plus Sign 解题报告(Python) 作者: 负雪明烛 id: fuxuemingzhu 个人博客: http://fuxuemingzhu.cn ...
- 【leetcode】657. Robot Return to Origin
Algorithm [leetcode]657. Robot Return to Origin https://leetcode.com/problems/robot-return-to-origin ...
- 【LeetCode】Permutations 解题报告
全排列问题.经常使用的排列生成算法有序数法.字典序法.换位法(Johnson(Johnson-Trotter).轮转法以及Shift cursor cursor* (Gao & Wang)法. ...
- 【LeetCode】分治法 divide and conquer (共17题)
链接:https://leetcode.com/tag/divide-and-conquer/ [4]Median of Two Sorted Arrays [23]Merge k Sorted Li ...
- 【LeetCode】1162. 地图分析 As Far from Land as Possible(Python)
作者: 负雪明烛 id: fuxuemingzhu 个人博客:http://fuxuemingzhu.cn/ 目录 题目描述 题目大意 解题方法 这个题想考察什么? 剩下的任务就是套模板! 日期 题目 ...
- 【LeetCode】代码模板,刷题必会
目录 二分查找 排序的写法 BFS的写法 DFS的写法 回溯法 树 递归 迭代 前序遍历 中序遍历 后序遍历 构建完全二叉树 并查集 前缀树 图遍历 Dijkstra算法 Floyd-Warshall ...
- 【LeetCode】Island Perimeter 解题报告
[LeetCode]Island Perimeter 解题报告 [LeetCode] https://leetcode.com/problems/island-perimeter/ Total Acc ...
随机推荐
- [转帖]ORA-00600-[kcratr_nab_less_than_odr]问题小记
ORA-00600-[kcratr_nab_less_than_odr]问题小记 2018年03月12日 20:56:57 我不是VIP 阅读数 1500 https://blog.csdn.ne ...
- SpringBoot自动化配置之四:@Conditional注解详解
前言 之前在分析spring boot 源码时导出可见@ConditionalOnBean 之类的注解,那么它到底是如何使用的以及其工作流程如何,我们这里就围绕以下几点来分析: @Conditiona ...
- C++学习 之 变量和常量的使用(笔记)
一.变量 1.对变量含义的理解: 变量就像是经过工厂加工后有一定容量的容器.在变量定义时,系统充当了工厂的角色,按照类型为变量分配相应的空间.定义完成的变量可以存放相应类型的值,存放的值大于变量所能接 ...
- JavaScript Let 和 Const
来源:菜鸟教程:https://www.runoob.com/js/js-let-const.html JavaScript let 和 const ECMAScript 2015(ECMAScrip ...
- python线程的几种创建方式
Python3 线程中常用的两个模块为: _thread threading(推荐使用) 使用Thread类创建 import threading from time import sleep,cti ...
- Charles学习(三)之使用Map local代理本地静态资源以及配置网页代理在Mac模拟器调试iOS客户端
前言 问题一:我们在App内嵌H5开发的过程中,肯定会遇到一个问题就是我不想在chrome的控制台中调试,我想要在手机上调试,那么如何解决这个问题呢? 问题二:我们期待调试时达到的效果就是和Charl ...
- MySQL两种内核对比
MySQL内核 https://blog.csdn.net/baichoufei90/article/details/83504446 关键字:全文索引 索引外置 两种内核:MyISAM 和InnoD ...
- 使用CSS设置背景图片,图片比较大,完全显示在一个DIV中
做的时候想要边框为比较好看的样式,需要UI切图并且放在div中,看起来会好看点 像这样的,我随便挑选了一个,UI帮我切图出来 需要把这个图片填到相应的div里面,但是很显然碰到一个问题,图片太大,而且 ...
- git配置ssh秘钥(公钥以及私钥)linux
本文默认已经安装git,并有github或者gitlab账号 git在linux下安装参考:https://www.cnblogs.com/lz0925/p/10791147.html 在Linux中 ...
- 你所不知的VIM强大功能
1. 可视化区块(Visual Block) (1)cd ~ 切换到自己的家目录(本范例中为root用户) (2)touch test1 test2 建立两个文件做演示(3)last > tes ...