Algorithm --> 最长公共子序列（LCS）

 

一、什么是最长公共子序列

   

　　什么是最长公共子序列呢？举个简单的例子吧，一个数列S，若分别是两个或多个已知序列的子序列，且是所有符合条件序列中最长的，则S称为已知序列的最长公共子序列。

　　举例如下，如：有两个随机数列，1 2 3 4 5 6 和 3 4 5 8 9，则它们的最长公共子序列便是：3 4 5。

 

     最长公共子串（Longest Common Substirng）和最长公共子序列（Longest Common Subsequence，LCS）的区别为：子串是串的一个连续的部分，子序列则是从不改变序列的顺序，而从序列中去掉任意的元素而获得新的序列；也就是说，子串中字符的位置必须是连续的，子序列则可以不必连续。

 

 

 

二、解决方案

<1> 枚举法

这种方法是最简单，也是最容易想到的，当然时间复杂度也是龟速的，我们可以分析一下，刚才也说过了cnblogs的子序列个数有2⁷个 ，延伸一下：一个长度为N的字符串，其子序列有2^N个，每个子序列要在第二个长度为N的字符串中去匹配，匹配一次需要O(N)的时间，总共也就是O(N*2^N)，可以看出，时间复杂度为指数级，恐怖的令人窒息。

<2> 动态规划

最长公共子序列的结构有如下表示：

设序列X=<x1, x2, …, xm>和Y=<y1, y2, …, yn>的一个最长公共子序列Z=<z1, z2, …, zk>，则：

   1> 若 xm=yn，则 zk=xm=yn，且Zk-1是Xm-1和Yn-1的最长公共子序列； 

   2> 若 xm≠yn且 zk≠xm ，则 Z是 Xm-1和 Y的最长公共子序列；

   3> 若 xm≠yn且 zk≠yn ，则 Z是 X和 Yn-1的最长公共子序列；

 

现有两个序列X={x₁,x₂,x_3，...x_i}，Y={y₁,y₂,y_3，....，y_i}，设一个C[i,j]: 保存X_i与Y_j的LCS的长度，递推方程为：

代码：

    //只能打印一个最长公共子序列
    #include <iostream>
    using namespace std;

     const int X = , Y = ;        //串的最大长度
     char result[X+];                    //用于保存结果
     int count=;                        //用于保存公共最长公共子串的个数

     /*功能：计算最优值
      *参数：
      *        x:字符串x
      *        y:字符串y
      *        b:标志数组
      *        xlen:字符串x的长度
      *        ylen:字符串y的长度
      *返回值：最长公共子序列的长度
      *
      */
     int Lcs_Length(string x, string y, int b[][Y+],int xlen,int ylen)
     {
         int i = ;
         int j = ;

         int c[X+][Y+];
         for (i = ; i<=xlen; i++)
         {
             c[i][]=;
         }
         for (i = ; i <= ylen; i++ )
         {
             c[][i]=;
         }
         for (i = ; i <= xlen; i++)
         {
             for (j = ; j <= ylen; j++)
             {
                 if (x[i - ] == y[j - ])
                 {
                     c[i][j] = c[i-][j-]+;
                     b[i][j] = ;
                 }
                 else if (c[i-][j] > c[i][j-])
                 {
                     c[i][j] = c[i-][j];
                     b[i][j] = ;
                 }
                 else if(c[i-][j] <= c[i][j-])
                 {
                     c[i][j] = c[i][j-];
                     b[i][j] = ;
                 }
             }
         }
　　　　  return c[xlen][ylen];
     }

    void Display_Lcs(int i, int j, string x, int b[][Y+],int current_Len)
     {
         if (i == || j==)
         {
             return;
         }
         if(b[i][j]== )
         {
             current_Len--;
             result[current_Len]=x[i- ];
             Display_Lcs(i-, j-, x, b, current_Len);
         }
         else if(b[i][j] == )
         {
             Display_Lcs(i-, j, x, b, current_Len);
         }
         else if(b[i][j]==)
         {
              Display_Lcs(i, j-, x, b, current_Len);
         }
         else
         {
              Display_Lcs(i-,j,x,b, current_Len);
         }
     }

     int main(int argc, char* argv[])
     {
         string x = "ABCBDAB";
         string y = "BDCABA";
         int xlen = x.length();
         int ylen = y.length();

         int b[X + ][Y + ];

         int lcs_max_len = Lcs_Length( x, y, b, xlen,ylen );
         cout << lcs_max_len << endl;

         Display_Lcs( xlen, ylen, x, b, lcs_max_len );

         //打印结果如下所示
        for(int i = ; i < lcs_max_len; i++)
        {
            cout << result[i];
        }
        cout << endl;
        return ;
     }

运行结果如下：

BDAB

由于有时并不是只有一个最长公共子序列，所以，对上面的代码进行改进，增加一个数组保存结果等....代码如下所示。

//求取所有的最长公共子序列
    #include <iostream>
    using namespace std;

     const int X = , Y = ;        //串的最大长度
     char result[X+];                    //用于保存结果
     int cnt = ;                        //用于保存公共最长公共子串的个数

     /*功能：计算最优值
      *参数：
      *        x:字符串x
      *        y:字符串y
      *        b:标志数组
      *        xlen:字符串x的长度
      *        ylen:字符串y的长度
      *返回值：最长公共子序列的长度
      *
      */
     int Lcs_Length(string x, string y, int b[][Y+],int xlen,int ylen)
     {
         int i = ;
         int j = ;

         int c[X+][Y+];
         for (i = ; i<=xlen; i++)
         {
             c[i][]=;
         }
         for (i = ; i <= ylen; i++ )
         {
             c[][i]=;
         }
         for (i = ; i <= xlen; i++)
         {

             for (j = ; j <= ylen; j++)
             {
                 if (x[i - ] == y[j - ])
                 {
                     c[i][j] = c[i-][j-]+;
                     b[i][j] = ;
                 }
                 else if (c[i-][j] > c[i][j-])
                 {
                     c[i][j] = c[i-][j];
                     b[i][j] = ;
                 }
                 else if(c[i-][j] < c[i][j-])
                 {
                     c[i][j] = c[i][j-];
                     b[i][j] = ;
                 }
                 else
                 {
                     c[i][j] = c[i][j-]; //或者c[i][j]=c[i-1][j];
                     b[i][j] = ;
                 }
             }
         }
   　　　　return c[xlen][ylen];
     }

     /*功能：计算最长公共子序列
      *参数：
      *        xlen:字符串x的长度
      *        ylen:字符串y的长度
      *        x    :字符串x
      *        b:标志数组
      *        current_len:当前长度
      *        lcs_max_len:最长公共子序列长度
      *
      */
     void Display_Lcs(int i, int j, string x, int b[][Y+],int current_len,int lcs_max_len)
     {
         if (i == || j==)
         {
             for(int s=; s < lcs_max_len; s++)
             {
                 cout << result[s];
             }
             cout<<endl;
             cnt++;
             return;
         }
         if(b[i][j]== )
         {
             current_len--;
             result[current_len]=x[i- ];
             Display_Lcs(i-, j-, x, b,current_len,lcs_max_len);
         }
         else if(b[i][j] == )
         {
             Display_Lcs(i-, j, x, b,current_len,lcs_max_len);
         }
         else if(b[i][j]==)
         {
             Display_Lcs(i, j-, x, b,current_len,lcs_max_len);
         }
         else
         {
             Display_Lcs(i,j-,x,b,current_len,lcs_max_len);
             Display_Lcs(i-,j,x,b,current_len,lcs_max_len);
         }
     }

     int main(int argc, char* argv[])
     {
         string x = "ABCBDAB";
         string y = "BDCABA";
         int xlen = x.length();
         int ylen = y.length();

         int b[X + ][Y + ];

         int lcs_max_len = Lcs_Length( x, y, b, xlen,ylen );
         cout << lcs_max_len << endl;

         Display_Lcs( xlen, ylen, x, b, lcs_max_len, lcs_max_len );
         cout << "共有：" << cnt << "种";

         return ;
     }

运行结果如下：

BDAB
BCAB
BCBA
共有：  种

/****************************************

　　　　update

****************************************/

示例：

#include <iostream>
using namespace std;
#define X_LEN  7
#define Y_LEN  6
#define EQUAL  0
#define UP    1
#define LEVEL  2
void lcs_length(char* X,char* Y,int c[X_LEN+][Y_LEN+],int b[X_LEN+][Y_LEN+]);
void print_lcs(int b[X_LEN+][Y_LEN+],char *X,int i,int j);

int main()
{
    char X[X_LEN+] = {' ','A','B','C','B','D','A','B'};
    char Y[Y_LEN+] = {' ','B','D','C','A','B','A'};
    int c[X_LEN+][Y_LEN+]={};
    int b[X_LEN+][Y_LEN+] = {};
    int i,j;
    lcs_length(X,Y,c,b);
    for(i=;i<=X_LEN;i++)
    {
         for(j=;j<=Y_LEN;j++)
            cout<<c[i][j]<<" ";
        cout<<endl;
    }
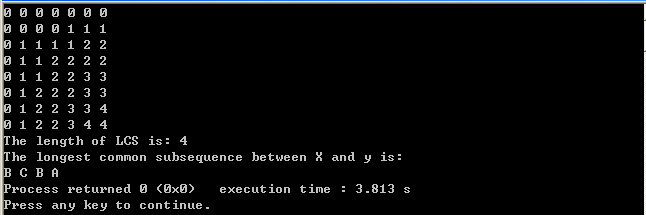
    cout<<"The length of LCS is: "<<c[X_LEN][Y_LEN]<<endl;
    cout<<"The longest common subsequence between X and y is: "<<endl;
    print_lcs(b,X,X_LEN,Y_LEN);
    return ;
}
//采用动态规划方法自底向上的进行计算，寻找最优解
void lcs_length(char* X,char* Y,int c[X_LEN+][Y_LEN+],int b[X_LEN+][Y_LEN+])
{
    int i,j;
    //设置边界条件，即i=0或者j=0
    for(i=;i<X_LEN;i++)
        c[i][] = ;
    for(j=;j<Y_LEN;j++)
        c[][j] = ;
    for(i=;i<=X_LEN;i++)
        for(j=;j<=Y_LEN;j++)
        {
            if(X[i] == Y[j])   //满足递归公式第二条
            {
                c[i][j] = c[i-][j-]+;
                b[i][j] = EQUAL ;
            }
            else if(c[i-][j] >= c[i][j-])  //递归公式第三条
            {
                c[i][j] = c[i-][j];
                b[i][j] = UP;
            }
            else
            {
                c[i][j] = c[i][j-];
                b[i][j] = LEVEL;
            }
        }
}
void print_lcs(int b[X_LEN+][Y_LEN+],char *X,int i,int j)
{
    if(i== || j==)
        return;
    if(b[i][j] == EQUAL)
    {
        print_lcs(b,X,i-,j-);
        cout<<X[i]<<" ";
    }
    else
        if(b[i][j] == UP)
            print_lcs(b,X,i-,j);
    else
        print_lcs(b,X,i,j-);
}

结果：