数据结构之B树、B+树（二）---代码实现
B-Tree | Set 1 （construct）
Following is an example B-Tree of minimum degree 3. Note that in practical B-Trees, the value of minimum degree is much more than 3.
定义B-Tree
// C++ implemntation of search() and traverse() methods
#include<iostream>
using namespace std;
// A BTree node
class BTreeNode
{
int *keys; // An array of keys
int t; // Minimum degree (defines the range for number of keys)
BTreeNode **C; // An array of child pointers
int n; // Current number of keys
bool leaf; // Is true when node is leaf. Otherwise false
public:
BTreeNode(int _t, bool _leaf); // Constructor
// A function to traverse all nodes in a subtree rooted with this node
void traverse();
// A function to search a key in subtree rooted with this node.
BTreeNode *search(int k); // returns NULL if k is not present.
// Make BTree friend of this so that we can access private members of this
// class in BTree functions
friend class BTree;
};
// A BTree
class BTree
{
BTreeNode *root; // Pointer to root node
int t; // Minimum degree
public:
// Constructor (Initializes tree as empty)
BTree(int _t)
{ root = NULL; t = _t; }
// function to traverse the tree
void traverse()
{ if (root != NULL) root->traverse(); }
// function to search a key in this tree
BTreeNode* search(int k)
{ return (root == NULL)? NULL : root->search(k); }
};
// Constructor for BTreeNode class
BTreeNode::BTreeNode(int _t, bool _leaf)
{
// Copy the given minimum degree and leaf property
t = _t;
leaf = _leaf;
// Allocate memory for maximum number of possible keys
// and child pointers
keys = new int[*t-];
C = new BTreeNode *[*t];
// Initialize the number of keys as 0
n = ;
}
Traversal
Traversal is also similar to Inorder traversal of Binary Tree. We start from the leftmost child, recursively print the leftmost child, then repeat the same process for remaining children and keys. In the end, recursively print the rightmost child.
// Function to traverse all nodes in a subtree rooted with this node
void BTreeNode::traverse()
{
// There are n keys and n+1 children, travers through n keys
// and first n children
int i;
for (i = ; i < n; i++)
{
// If this is not leaf, then before printing key[i],
// traverse the subtree rooted with child C[i].
if (leaf == false)
C[i]->traverse();
cout << " " << keys[i];
}
// Print the subtree rooted with last child
if (leaf == false)
C[i]->traverse();
}
Search
Search is similar to search in Binary Search Tree. Let the key to be searched be k. We start from root and recursively traverse down. For every visited non-leaf node, if the node has key, we simply return the node. Otherwise we recur down to the appropriate child (The child which is just before the first greater key) of the node. If we reach a leaf node and don’t find k in the leaf node, we return NULL.
// Function to search key k in subtree rooted with this node
BTreeNode *BTreeNode::search(int k)
{
// Find the first key greater than or equal to k
int i = ;
while (i < n && k > keys[i])
i++;
// If the found key is equal to k, return this node
if (keys[i] == k)
return this;
// If key is not found here and this is a leaf node
if (leaf == true)
return NULL;
// Go to the appropriate child
return C[i]->search(k);
}
B-Tree | Set 2 (Insert)
Insertion
1) Initialize x as root.
2) While x is not leaf, do following
......a) Find the child of x that is going to to be traversed next. Let the child be y.
......b) If y is not full, change x to point to y.
......c) If y is full, split it and change x to point to one of the two parts of y. If k is smaller than mid key in y, then set x as first part of y. Else second part of y. When we split y, we move a key from y to its parent x.
3) The loop in step 2 stops when x is leaf. x must have space for 1 extra key as we have been splitting all nodes in advance. So simply insert k to x.
-------------------------------------------------------------------------------------------------------------------------------------------
Initially root is NULL. Let us first insert 10.
Let us now insert 20, 30, 40 and 50. They all will be inserted in root because maximum number of keys a node can accommodate is 2*t – 1 which is 5.
Let us now insert 60. Since root node is full, it will first split into two, then 60 will be inserted into the appropriate child.
Let us now insert 70 and 80. These new keys will be inserted into the appropriate leaf without any split.
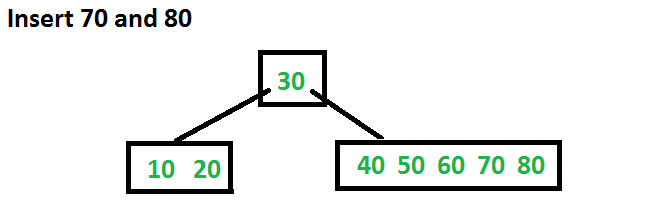
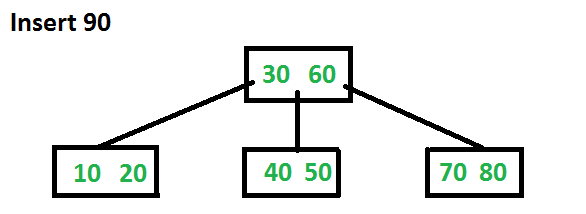
Let us now insert 90. This insertion will cause a split. The middle key will go up to the parent.
// C++ program for B-Tree insertion
#include<iostream>
using namespace std;
// A BTree node
class BTreeNode
{
int *keys; // An array of keys
int t; // Minimum degree (defines the range for number of keys)
BTreeNode **C; // An array of child pointers
int n; // Current number of keys
bool leaf; // Is true when node is leaf. Otherwise false
public:
BTreeNode(int _t, bool _leaf); // Constructor
// A utility function to insert a new key in the subtree rooted with
// this node. The assumption is, the node must be non-full when this
// function is called
void insertNonFull(int k);
// A utility function to split the child y of this node. i is index of y in
// child array C[]. The Child y must be full when this function is called
void splitChild(int i, BTreeNode *y);
// A function to traverse all nodes in a subtree rooted with this node
void traverse();
// A function to search a key in subtree rooted with this node.
BTreeNode *search(int k); // returns NULL if k is not present.
// Make BTree friend of this so that we can access private members of this
// class in BTree functions
friend class BTree;
};
// A BTree
class BTree
{
BTreeNode *root; // Pointer to root node
int t; // Minimum degree
public:
// Constructor (Initializes tree as empty)
BTree(int _t)
{ root = NULL; t = _t; }
// function to traverse the tree
void traverse()
{ if (root != NULL) root->traverse(); }
// function to search a key in this tree
BTreeNode* search(int k)
{ return (root == NULL)? NULL : root->search(k); }
// The main function that inserts a new key in this B-Tree
void insert(int k);
};
// Constructor for BTreeNode class
BTreeNode::BTreeNode(int t1, bool leaf1)
{
// Copy the given minimum degree and leaf property
t = t1;
leaf = leaf1;
// Allocate memory for maximum number of possible keys
// and child pointers
keys = new int[*t-];
C = new BTreeNode *[*t];
// Initialize the number of keys as 0
n = ;
}
// Function to traverse all nodes in a subtree rooted with this node
void BTreeNode::traverse()
{
// There are n keys and n+1 children, travers through n keys
// and first n children
int i;
for (i = ; i < n; i++)
{
// If this is not leaf, then before printing key[i],
// traverse the subtree rooted with child C[i].
if (leaf == false)
C[i]->traverse();
cout << " " << keys[i];
}
// Print the subtree rooted with last child
if (leaf == false)
C[i]->traverse();
}
// Function to search key k in subtree rooted with this node
BTreeNode *BTreeNode::search(int k)
{
// Find the first key greater than or equal to k
int i = ;
while (i < n && k > keys[i])
i++;
// If the found key is equal to k, return this node
if (keys[i] == k)
return this;
// If key is not found here and this is a leaf node
if (leaf == true)
return NULL;
// Go to the appropriate child
return C[i]->search(k);
}
// The main function that inserts a new key in this B-Tree
void BTree::insert(int k)
{
// If tree is empty
if (root == NULL)
{
// Allocate memory for root
root = new BTreeNode(t, true);
root->keys[] = k; // Insert key
root->n = ; // Update number of keys in root
}
else // If tree is not empty
{
// If root is full, then tree grows in height
if (root->n == *t-)
{
// Allocate memory for new root
BTreeNode *s = new BTreeNode(t, false);
// Make old root as child of new root
s->C[] = root;
// Split the old root and move 1 key to the new root
s->splitChild(, root);
// New root has two children now. Decide which of the
// two children is going to have new key
int i = ;
if (s->keys[] < k)
i++;
s->C[i]->insertNonFull(k);
// Change root
root = s;
}
else // If root is not full, call insertNonFull for root
root->insertNonFull(k);
}
}
// A utility function to insert a new key in this node
// The assumption is, the node must be non-full when this
// function is called
void BTreeNode::insertNonFull(int k)
{
// Initialize index as index of rightmost element
int i = n-;
// If this is a leaf node
if (leaf == true)
{
// The following loop does two things
// a) Finds the location of new key to be inserted
// b) Moves all greater keys to one place ahead
while (i >= && keys[i] > k)
{
keys[i+] = keys[i];
i--;
}
// Insert the new key at found location
keys[i+] = k;
n = n+;
}
else // If this node is not leaf
{
// Find the child which is going to have the new key
while (i >= && keys[i] > k)
i--;
// See if the found child is full
if (C[i+]->n == *t-)
{
// If the child is full, then split it
splitChild(i+, C[i+]);
// After split, the middle key of C[i] goes up and
// C[i] is splitted into two. See which of the two
// is going to have the new key
if (keys[i+] < k)
i++;
}
C[i+]->insertNonFull(k);
}
}
// A utility function to split the child y of this node
// Note that y must be full when this function is called
void BTreeNode::splitChild(int i, BTreeNode *y)
{
// Create a new node which is going to store (t-1) keys
// of y
BTreeNode *z = new BTreeNode(y->t, y->leaf);
z->n = t - ;
// Copy the last (t-1) keys of y to z
for (int j = ; j < t-; j++)
z->keys[j] = y->keys[j+t];
// Copy the last t children of y to z
if (y->leaf == false)
{
for (int j = ; j < t; j++)
z->C[j] = y->C[j+t];
}
// Reduce the number of keys in y
y->n = t - ;
// Since this node is going to have a new child,
// create space of new child
for (int j = n; j >= i+; j--)
C[j+] = C[j];
// Link the new child to this node
C[i+] = z;
// A key of y will move to this node. Find location of
// new key and move all greater keys one space ahead
for (int j = n-; j >= i; j--)
keys[j+] = keys[j];
// Copy the middle key of y to this node
keys[i] = y->keys[t-];
// Increment count of keys in this node
n = n + ;
}
// Driver program to test above functions
int main()
{
BTree t(); // A B-Tree with minium degree 3
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
cout << "Traversal of the constucted tree is ";
t.traverse();
int k = ;
(t.search(k) != NULL)? cout << "\nPresent" : cout << "\nNot Present";
k = ;
(t.search(k) != NULL)? cout << "\nPresent" : cout << "\nNot Present";
return ;
}
Output:
Traversal of the constucted tree is
Present
Not Present
B-Tree | Set 3 (Delete)
Delete
We sketch how deletion works with various cases of deleting keys from a B-tree.
1. If the key k is in node x and x is a leaf, delete the key k from x.
2. If the key k is in node x and x is an internal node, do the following.
a) If the child y that precedes k in node x has at least t keys, then find the predecessor k0 of k in the sub-tree rooted at y. Recursively delete k0, and replace k by k0 in x. (We can find k0 and delete it in a single downward pass.)
b) If y has fewer than t keys, then, symmetrically, examine the child z that follows k in node x. If z has at least t keys, then find the successor k0 of k in the subtree rooted at z. Recursively delete k0, and replace k by k0 in x. (We can find k0 and delete it in a single downward pass.)
c) Otherwise, if both y and z have only t-1 keys, merge k and all of z into y, so that x loses both k and the pointer to z, and y now contains 2t-1 keys. Then free z and recursively delete k from y.
3. If the key k is not present in internal node x, determine the root x.c(i) of the appropriate subtree that must contain k, if k is in the tree at all. If x.c(i) has only t-1 keys, execute step 3a or 3b as necessary to guarantee that we descend to a node containing at least t keys. Then finish by recursing on the appropriate child of x.
a) If x.c(i) has only t-1 keys but has an immediate sibling with at least t keys, give x.c(i) an extra key by moving a key from x down into x.c(i), moving a key from x.c(i) ’s immediate left or right sibling up into x, and moving the appropriate child pointer from the sibling into x.c(i).
b) If x.c(i) and both of x.c(i)’s immediate siblings have t-1 keys, merge x.c(i) with one sibling, which involves moving a key from x down into the new merged node to become the median key for that node.
Since most of the keys in a B-tree are in the leaves, deletion operations are most often used to delete keys from leaves. The recursive delete procedure then acts in one downward pass through the tree, without having to back up. When deleting a key in an internal node, however, the procedure makes a downward pass through the tree but may have to return to the node from which the key was deleted to replace the key with its predecessor or successor (cases 2a and 2b).
The following figures from CLRS book explain the deletion porcess.
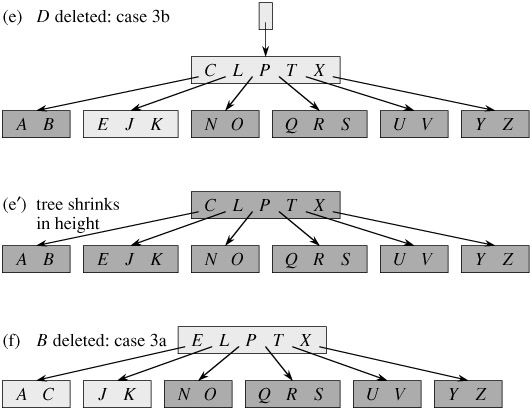
Implementation:
/* The following program performs deletion on a B-Tree. It contains functions
specific for deletion along with all the other functions provided in the
previous articles on B-Trees. See http://www.geeksforgeeks.org/b-tree-set-1-introduction-2/
for previous article.
The deletion function has been compartmentalized into 8 functions for ease
of understanding and clarity
The following functions are exclusive for deletion
In class BTreeNode:
1) remove
2) removeFromLeaf
3) removeFromNonLeaf
4) getPred
5) getSucc
6) borrowFromPrev
7) borrowFromNext
8) merge
9) findKey
In class BTree:
1) remove
The removal of a key from a B-Tree is a fairly complicated process. The program handles
all the 6 different cases that might arise while removing a key.
Testing: The code has been tested using the B-Tree provided in the CLRS book( included
in the main function ) along with other cases.
Reference: CLRS3 - Chapter 18 - (499-502)
It is advised to read the material in CLRS before taking a look at the code. */
#include<iostream>
using namespace std;
// A BTree node
class BTreeNode
{
int *keys; // An array of keys
int t; // Minimum degree (defines the range for number of keys)
BTreeNode **C; // An array of child pointers
int n; // Current number of keys
bool leaf; // Is true when node is leaf. Otherwise false
public:
BTreeNode(int _t, bool _leaf); // Constructor
// A function to traverse all nodes in a subtree rooted with this node
void traverse();
// A function to search a key in subtree rooted with this node.
BTreeNode *search(int k); // returns NULL if k is not present.
// A function that returns the index of the first key that is greater
// or equal to k
int findKey(int k);
// A utility function to insert a new key in the subtree rooted with
// this node. The assumption is, the node must be non-full when this
// function is called
void insertNonFull(int k);
// A utility function to split the child y of this node. i is index
// of y in child array C[]. The Child y must be full when this
// function is called
void splitChild(int i, BTreeNode *y);
// A wrapper function to remove the key k in subtree rooted with
// this node.
void remove(int k);
// A function to remove the key present in idx-th position in
// this node which is a leaf
void removeFromLeaf(int idx);
// A function to remove the key present in idx-th position in
// this node which is a non-leaf node
void removeFromNonLeaf(int idx);
// A function to get the predecessor of the key- where the key
// is present in the idx-th position in the node
int getPred(int idx);
// A function to get the successor of the key- where the key
// is present in the idx-th position in the node
int getSucc(int idx);
// A function to fill up the child node present in the idx-th
// position in the C[] array if that child has less than t-1 keys
void fill(int idx);
// A function to borrow a key from the C[idx-1]-th node and place
// it in C[idx]th node
void borrowFromPrev(int idx);
// A function to borrow a key from the C[idx+1]-th node and place it
// in C[idx]th node
void borrowFromNext(int idx);
// A function to merge idx-th child of the node with (idx+1)th child of
// the node
void merge(int idx);
// Make BTree friend of this so that we can access private members of
// this class in BTree functions
friend class BTree;
};
class BTree
{
BTreeNode *root; // Pointer to root node
int t; // Minimum degree
public:
// Constructor (Initializes tree as empty)
BTree(int _t)
{
root = NULL;
t = _t;
}
void traverse()
{
if (root != NULL) root->traverse();
}
// function to search a key in this tree
BTreeNode* search(int k)
{
return (root == NULL)? NULL : root->search(k);
}
// The main function that inserts a new key in this B-Tree
void insert(int k);
// The main function that removes a new key in thie B-Tree
void remove(int k);
};
BTreeNode::BTreeNode(int t1, bool leaf1)
{
// Copy the given minimum degree and leaf property
t = t1;
leaf = leaf1;
// Allocate memory for maximum number of possible keys
// and child pointers
keys = new int[*t-];
C = new BTreeNode *[*t];
// Initialize the number of keys as 0
n = ;
}
// A utility function that returns the index of the first key that is
// greater than or equal to k
int BTreeNode::findKey(int k)
{
int idx=;
while (idx<n && keys[idx] < k)
++idx;
return idx;
}
// A function to remove the key k from the sub-tree rooted with this node
void BTreeNode::remove(int k)
{
int idx = findKey(k);
// The key to be removed is present in this node
if (idx < n && keys[idx] == k)
{
// If the node is a leaf node - removeFromLeaf is called
// Otherwise, removeFromNonLeaf function is called
if (leaf)
removeFromLeaf(idx);
else
removeFromNonLeaf(idx);
}
else
{
// If this node is a leaf node, then the key is not present in tree
if (leaf)
{
cout << "The key "<< k <<" is does not exist in the tree\n";
return;
}
// The key to be removed is present in the sub-tree rooted with this node
// The flag indicates whether the key is present in the sub-tree rooted
// with the last child of this node
bool flag = ( (idx==n)? true : false );
// If the child where the key is supposed to exist has less that t keys,
// we fill that child
if (C[idx]->n < t)
fill(idx);
// If the last child has been merged, it must have merged with the previous
// child and so we recurse on the (idx-1)th child. Else, we recurse on the
// (idx)th child which now has atleast t keys
if (flag && idx > n)
C[idx-]->remove(k);
else
C[idx]->remove(k);
}
return;
}
// A function to remove the idx-th key from this node - which is a leaf node
void BTreeNode::removeFromLeaf (int idx)
{
// Move all the keys after the idx-th pos one place backward
for (int i=idx+; i<n; ++i)
keys[i-] = keys[i];
// Reduce the count of keys
n--;
return;
}
// A function to remove the idx-th key from this node - which is a non-leaf node
void BTreeNode::removeFromNonLeaf(int idx)
{
int k = keys[idx];
// If the child that precedes k (C[idx]) has atleast t keys,
// find the predecessor 'pred' of k in the subtree rooted at
// C[idx]. Replace k by pred. Recursively delete pred
// in C[idx]
if (C[idx]->n >= t)
{
int pred = getPred(idx);
keys[idx] = pred;
C[idx]->remove(pred);
}
// If the child C[idx] has less that t keys, examine C[idx+1].
// If C[idx+1] has atleast t keys, find the successor 'succ' of k in
// the subtree rooted at C[idx+1]
// Replace k by succ
// Recursively delete succ in C[idx+1]
else if (C[idx+]->n >= t)
{
int succ = getSucc(idx);
keys[idx] = succ;
C[idx+]->remove(succ);
}
// If both C[idx] and C[idx+1] has less that t keys,merge k and all of C[idx+1]
// into C[idx]
// Now C[idx] contains 2t-1 keys
// Free C[idx+1] and recursively delete k from C[idx]
else
{
merge(idx);
C[idx]->remove(k);
}
return;
}
// A function to get predecessor of keys[idx]
int BTreeNode::getPred(int idx)
{
// Keep moving to the right most node until we reach a leaf
BTreeNode *cur=C[idx];
while (!cur->leaf)
cur = cur->C[cur->n];
// Return the last key of the leaf
return cur->keys[cur->n-];
}
int BTreeNode::getSucc(int idx)
{
// Keep moving the left most node starting from C[idx+1] until we reach a leaf
BTreeNode *cur = C[idx+];
while (!cur->leaf)
cur = cur->C[];
// Return the first key of the leaf
return cur->keys[];
}
// A function to fill child C[idx] which has less than t-1 keys
void BTreeNode::fill(int idx)
{
// If the previous child(C[idx-1]) has more than t-1 keys, borrow a key
// from that child
if (idx!= && C[idx-]->n>=t)
borrowFromPrev(idx);
// If the next child(C[idx+1]) has more than t-1 keys, borrow a key
// from that child
else if (idx!=n && C[idx+]->n>=t)
borrowFromNext(idx);
// Merge C[idx] with its sibling
// If C[idx] is the last child, merge it with with its previous sibling
// Otherwise merge it with its next sibling
else
{
if (idx != n)
merge(idx);
else
merge(idx-);
}
return;
}
// A function to borrow a key from C[idx-1] and insert it
// into C[idx]
void BTreeNode::borrowFromPrev(int idx)
{
BTreeNode *child=C[idx];
BTreeNode *sibling=C[idx-];
// The last key from C[idx-1] goes up to the parent and key[idx-1]
// from parent is inserted as the first key in C[idx]. Thus, the loses
// sibling one key and child gains one key
// Moving all key in C[idx] one step ahead
for (int i=child->n-; i>=; --i)
child->keys[i+] = child->keys[i];
// If C[idx] is not a leaf, move all its child pointers one step ahead
if (!child->leaf)
{
for(int i=child->n; i>=; --i)
child->C[i+] = child->C[i];
}
// Setting child's first key equal to keys[idx-1] from the current node
child->keys[] = keys[idx-];
// Moving sibling's last child as C[idx]'s first child
if (!leaf)
child->C[] = sibling->C[sibling->n];
// Moving the key from the sibling to the parent
// This reduces the number of keys in the sibling
keys[idx-] = sibling->keys[sibling->n-];
child->n += ;
sibling->n -= ;
return;
}
// A function to borrow a key from the C[idx+1] and place
// it in C[idx]
void BTreeNode::borrowFromNext(int idx)
{
BTreeNode *child=C[idx];
BTreeNode *sibling=C[idx+];
// keys[idx] is inserted as the last key in C[idx]
child->keys[(child->n)] = keys[idx];
// Sibling's first child is inserted as the last child
// into C[idx]
if (!(child->leaf))
child->C[(child->n)+] = sibling->C[];
//The first key from sibling is inserted into keys[idx]
keys[idx] = sibling->keys[];
// Moving all keys in sibling one step behind
for (int i=; i<sibling->n; ++i)
sibling->keys[i-] = sibling->keys[i];
// Moving the child pointers one step behind
if (!sibling->leaf)
{
for(int i=; i<=sibling->n; ++i)
sibling->C[i-] = sibling->C[i];
}
// Increasing and decreasing the key count of C[idx] and C[idx+1]
// respectively
child->n += ;
sibling->n -= ;
return;
}
// A function to merge C[idx] with C[idx+1]
// C[idx+1] is freed after merging
void BTreeNode::merge(int idx)
{
BTreeNode *child = C[idx];
BTreeNode *sibling = C[idx+];
// Pulling a key from the current node and inserting it into (t-1)th
// position of C[idx]
child->keys[t-] = keys[idx];
// Copying the keys from C[idx+1] to C[idx] at the end
for (int i=; i<sibling->n; ++i)
child->keys[i+t] = sibling->keys[i];
// Copying the child pointers from C[idx+1] to C[idx]
if (!child->leaf)
{
for(int i=; i<=sibling->n; ++i)
child->C[i+t] = sibling->C[i];
}
// Moving all keys after idx in the current node one step before -
// to fill the gap created by moving keys[idx] to C[idx]
for (int i=idx+; i<n; ++i)
keys[i-] = keys[i];
// Moving the child pointers after (idx+1) in the current node one
// step before
for (int i=idx+; i<=n; ++i)
C[i-] = C[i];
// Updating the key count of child and the current node
child->n += sibling->n+;
n--;
// Freeing the memory occupied by sibling
delete(sibling);
return;
}
// The main function that inserts a new key in this B-Tree
void BTree::insert(int k)
{
// If tree is empty
if (root == NULL)
{
// Allocate memory for root
root = new BTreeNode(t, true);
root->keys[] = k; // Insert key
root->n = ; // Update number of keys in root
}
else // If tree is not empty
{
// If root is full, then tree grows in height
if (root->n == *t-)
{
// Allocate memory for new root
BTreeNode *s = new BTreeNode(t, false);
// Make old root as child of new root
s->C[] = root;
// Split the old root and move 1 key to the new root
s->splitChild(, root);
// New root has two children now. Decide which of the
// two children is going to have new key
int i = ;
if (s->keys[] < k)
i++;
s->C[i]->insertNonFull(k);
// Change root
root = s;
}
else // If root is not full, call insertNonFull for root
root->insertNonFull(k);
}
}
// A utility function to insert a new key in this node
// The assumption is, the node must be non-full when this
// function is called
void BTreeNode::insertNonFull(int k)
{
// Initialize index as index of rightmost element
int i = n-;
// If this is a leaf node
if (leaf == true)
{
// The following loop does two things
// a) Finds the location of new key to be inserted
// b) Moves all greater keys to one place ahead
while (i >= && keys[i] > k)
{
keys[i+] = keys[i];
i--;
}
// Insert the new key at found location
keys[i+] = k;
n = n+;
}
else // If this node is not leaf
{
// Find the child which is going to have the new key
while (i >= && keys[i] > k)
i--;
// See if the found child is full
if (C[i+]->n == *t-)
{
// If the child is full, then split it
splitChild(i+, C[i+]);
// After split, the middle key of C[i] goes up and
// C[i] is splitted into two. See which of the two
// is going to have the new key
if (keys[i+] < k)
i++;
}
C[i+]->insertNonFull(k);
}
}
// A utility function to split the child y of this node
// Note that y must be full when this function is called
void BTreeNode::splitChild(int i, BTreeNode *y)
{
// Create a new node which is going to store (t-1) keys
// of y
BTreeNode *z = new BTreeNode(y->t, y->leaf);
z->n = t - ;
// Copy the last (t-1) keys of y to z
for (int j = ; j < t-; j++)
z->keys[j] = y->keys[j+t];
// Copy the last t children of y to z
if (y->leaf == false)
{
for (int j = ; j < t; j++)
z->C[j] = y->C[j+t];
}
// Reduce the number of keys in y
y->n = t - ;
// Since this node is going to have a new child,
// create space of new child
for (int j = n; j >= i+; j--)
C[j+] = C[j];
// Link the new child to this node
C[i+] = z;
// A key of y will move to this node. Find location of
// new key and move all greater keys one space ahead
for (int j = n-; j >= i; j--)
keys[j+] = keys[j];
// Copy the middle key of y to this node
keys[i] = y->keys[t-];
// Increment count of keys in this node
n = n + ;
}
// Function to traverse all nodes in a subtree rooted with this node
void BTreeNode::traverse()
{
// There are n keys and n+1 children, travers through n keys
// and first n children
int i;
for (i = ; i < n; i++)
{
// If this is not leaf, then before printing key[i],
// traverse the subtree rooted with child C[i].
if (leaf == false)
C[i]->traverse();
cout << " " << keys[i];
}
// Print the subtree rooted with last child
if (leaf == false)
C[i]->traverse();
}
// Function to search key k in subtree rooted with this node
BTreeNode *BTreeNode::search(int k)
{
// Find the first key greater than or equal to k
int i = ;
while (i < n && k > keys[i])
i++;
// If the found key is equal to k, return this node
if (keys[i] == k)
return this;
// If key is not found here and this is a leaf node
if (leaf == true)
return NULL;
// Go to the appropriate child
return C[i]->search(k);
}
void BTree::remove(int k)
{
if (!root)
{
cout << "The tree is empty\n";
return;
}
// Call the remove function for root
root->remove(k);
// If the root node has 0 keys, make its first child as the new root
// if it has a child, otherwise set root as NULL
if (root->n==)
{
BTreeNode *tmp = root;
if (root->leaf)
root = NULL;
else
root = root->C[];
// Free the old root
delete tmp;
}
return;
}
// Driver program to test above functions
int main()
{
BTree t(); // A B-Tree with minium degree 3
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
t.insert();
cout << "Traversal of tree constructed is\n";
t.traverse();
cout << endl;
t.remove();
cout << "Traversal of tree after removing 6\n";
t.traverse();
cout << endl;
t.remove();
cout << "Traversal of tree after removing 13\n";
t.traverse();
cout << endl;
t.remove();
cout << "Traversal of tree after removing 7\n";
t.traverse();
cout << endl;
t.remove();
cout << "Traversal of tree after removing 4\n";
t.traverse();
cout << endl;
t.remove();
cout << "Traversal of tree after removing 2\n";
t.traverse();
cout << endl;
t.remove();
cout << "Traversal of tree after removing 16\n";
t.traverse();
cout << endl;
return ;
}
Output:
Traversal of tree constructed is
Traversal of tree after removing
Traversal of tree after removing
Traversal of tree after removing
Traversal of tree after removing
Traversal of tree after removing
Traversal of tree after removing
数据结构之B树、B+树（二）---代码实现的更多相关文章
- ****** 二 ******、软设笔记【数据结构】-KMP算法、树、二叉树
五.KMP算法: *KMP算法是一种改进的字符串匹配算法. *KMP算法的关键是利用匹配失败后的信息,尽量减少模式串与主串的匹配次数以达到快速匹配的目的.具体实现就是实现一个next()函 ...
- 高级数据结构---赫(哈)夫曼树及java代码实现
我们经常会用到文件压缩,压缩之后文件会变小,便于传输,使用的时候又将其解压出来.为什么压缩之后会变小,而且压缩和解压也不会出错.赫夫曼编码和赫夫曼树了解一下. 赫夫曼树: 它是一种的叶子结点带有权重的 ...
- 数据结构图文解析之：树的简介及二叉排序树C++模板实现.
0. 数据结构图文解析系列 数据结构系列文章 数据结构图文解析之:数组.单链表.双链表介绍及C++模板实现 数据结构图文解析之:栈的简介及C++模板实现 数据结构图文解析之:队列详解与C++模板实现 ...
- 【Todo】字符串相关的各种算法，以及用到的各种数据结构，包括前缀树后缀树等各种树
另开一文分析字符串相关的各种算法,以及用到的各种数据结构,包括前缀树后缀树等各种树. 先来一个汇总, 算法: 本文中提到的字符串匹配算法有:KMP, BM, Horspool, Sunday, BF, ...
- 数据结构与算法->树->2-3-4树的查找,添加,删除(Java)
代码: 兵马未动,粮草先行 作者: 传说中的汽水枪 如有错误,请留言指正,欢迎一起探讨. 转载请注明出处. 目录 一. 2-3-4树的定义 二. 2-3-4树数据结构定义 三. 2-3-4树的可以得到 ...
- 洛谷 P3380 【【模板】二逼平衡树（树套树）】
其实比想象中的好理解啊 所谓树套树,就是在一棵树的基础上,每一个节点再维护一棵树 说白了,就是为了实现自己想要的操作和优秀的时间复杂度,来人为的增加一些毒瘤数据结构来维护一些什么东西 比如说这道题 如 ...
- bzoj3196: Tyvj 1730 二逼平衡树 树套树
地址:http://www.lydsy.com/JudgeOnline/problem.php?id=3196 题目: 3196: Tyvj 1730 二逼平衡树 Time Limit: 10 Sec ...
- 洛谷 P3380 bzoj3196 Tyvj1730 【模板】二逼平衡树（树套树）
[模板]二逼平衡树(树套树) 题目描述 您需要写一种数据结构(可参考题目标题),来维护一个有序数列,其中需要提供以下操作: 查询k在区间内的排名 查询区间内排名为k的值 修改某一位值上的数值 查询k在 ...
- [bzoj3196][Tyvj1730]二逼平衡树_树套树_位置线段树套非旋转Treap/树状数组套主席树/权值线段树套位置线段树
二逼平衡树 bzoj-3196 Tyvj-1730 题目大意:请写出一个维护序列的数据结构支持:查询给定权值排名:查询区间k小值:单点修改:查询区间内定值前驱:查询区间内定值后继. 注释:$1\le ...
- C# 表达式树遍历（二）
一.前言 上一篇我们对表达式树有了初步的认识,这里我们将对表达式树进行遍历,只有弄清楚了他的运行原理,我们才可以对他进行定制化修改. 表达式系列目录 C# 表达式树讲解(一) C# 表达式树遍历(二) ...
随机推荐
- C# if判断语句执行顺序
DataTable dt = null; )//不报错,因为先执行dt != null 成立时才执行dt.Rows.Count > 0 { } && dt != null)//报 ...
- JAVA_SE基础——29.构造函数
黑马程序员入学Blog... jvm创建Java对象时候需要调用构造器,默认是不带参数的.在构造器中,你可以让jvm帮你初始化一些参数或者执行一系列的动作. 它是对象创建中执行的函数,及第一个被执行的 ...
- [Oracle]undo表空间使用量为100%
在Toad中发现undo表空间undotbs1使用量已经达到100%,但是奇怪的是数据库并没有hang住,依然可以正常运转 通过Oracle提供的EM查看undotbs1表空间的使用,也达到了78.8 ...
- maven多环境部署
1.首先在maven的pom.xml文件中添加profiles标签,然后分别添加3个不同环境的profile, 本例中添加了dev.test.product三个环境,这个可以根据自己的需要添加和减少. ...
- SpringBoot+Angular2 开发环境搭建
https://segmentfault.com/a/1190000007921675
- android studio 何如修改报名
1. 重命名办法,网上很多见 2. 对于需要重新修改包名的级别的 a. 修改package 和 gradle 的包名,对应一致. b. 修改R 所在包名,使用crtl+n修改R文件的路径 c. 手动首 ...
- Django小范围傻瓜总结
1.母版: layout.html {% block x %}{% endblock %} 2.子版: {% extends 'layout' %} {% block x %}.......{% en ...
- windows10无法启动承载网络
每个都试一下
- Linux：sheel脚本for的用法，及日期参数+1day用法
记录下shell的for的用法,及参数是日期的情况下,该日期+1day的用法: #!/usr/bin/env bash source /app/catt/login.sh p_days="2 ...
- vue-router动态路由 刷新页面 静态资源没有加载的原因
在做项目的时候,发现刷新页面的时候,静态路由没有加载,度娘了一圈,终于解决了. vue-router使用history模式+使用嵌套路由: 访问路由和嵌套路由页面,显示正常,但是刷新页面的时候,嵌套路 ...