Codeforces Round #496 (Div. 3) E1. Median on Segments (Permutations Edition) (中位数,思维)

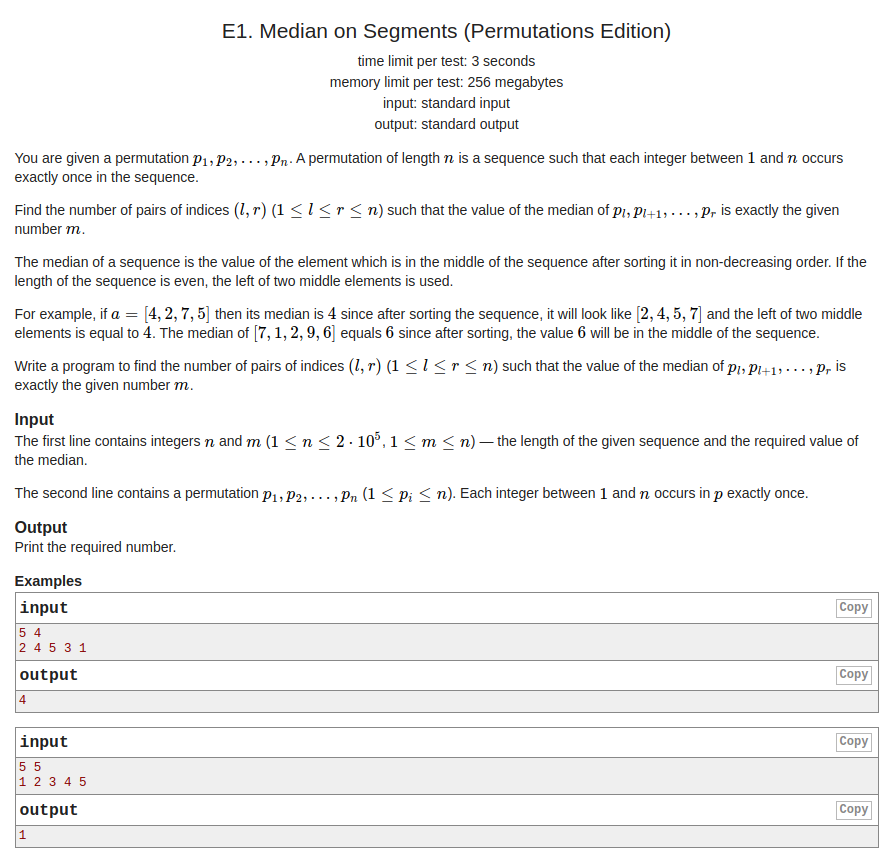
• 题意:给你一个数组,求有多少子数组的中位数等于\(m\).(若元素个数为偶数,取中间靠左的为中位数).

• 题解:由中位数的定义我们知道:若数组中\(<m\)的数有\(x\)个,\(>m\)的数有\(y\)个,只有\(x=y\)或\(y-x\)=1时,中位数才能取到\(m\),记\(m\)在原数组的位置为\(pos\).

  ​ 于是,我们先遍历\([pos,n]\),记录区间\([pos,i]\)中大于\(m\)的数和小于\(m\)的数个数差,用桶记录差值的个数.

  然后我们反着遍历\([1,pos]\),在段区间中,比\(m\)小的数可以和右边比\(m\)大的数抵消,比\(m\)大的数可以和右边比\(m\)小的数抵消,所以我们记录这些个数,然后每次更新一下答案即可(要考虑元素个数为偶数的情况且这题爆\(long\ long\)).

  ​ 其实可能有点难理解,我个人认为可以这么想,假如我们不看左边的部分,那么对于右边的部分,只有当差值为\(0\)或\(1\)的情况才满足条件,而差值是\(0\)和\(1\)的所有情况当我第一次遍历左边的时候(\(m\)本身)就全部加到答案中了,然后再不断向左遍历,和右边相抵消.(比如说,我左边有\(3\)个连续比\(m\)小的数,那么此时\(cnt=3\),而对于右边而言,假如右边的差值为\(3\),也就是说相对比\(m\)大的数有\(3\)个,而此时我左边有\(3\)个比\(m\)小的数,那么他们就抵消了,这种情况自然也就成立,显然,当右边为\(4\)的时候,左边为\(3\)也是成立的).

• 代码:

  #include <iostream>
  #include <cstdio>
  #include <cstring>
  #include <cmath>
  #include <algorithm>
  #include <stack>
  #include <queue>
  #include <vector>
  #include <map>
  #include <set>
  #include <unordered_set>
  #include <unordered_map>
  #define ll long long
  #define fi first
  #define se second
  #define pb push_back
  #define me memset
  const int N = 1e6 + 10;
  const int mod = 1e9 + 7;
  const int INF = 0x3f3f3f3f;
  using namespace std;
  typedef pair<int,int> PII;
  typedef pair<ll,ll> PLL;
  
  int n,m;
  ll a[N];
  int pos,cnt;
  map<int,ll> mp;
  int main() {
      ios::sync_with_stdio(false);cin.tie(0);
  	cin>>n>>m;
  	 for(int i=1;i<=n;++i){
  	 	cin>>a[i];
  	 	if(a[i]==m) pos=i;
  	 }
  	 for(int i=pos;i<=n;++i){
  	 	if(a[i]>m) cnt++;
  	 	else if(a[i]<m) cnt--;
  	    mp[cnt]++;
  	 }
  	 cnt=0;
  	 ll res=0;
  	 for(int i=pos;i>=1;--i){
  	 	if(a[i]<m) cnt++;
  	 	else if(a[i]>m) cnt--;
  	 	res+=mp[cnt]+mp[cnt+1];
  	 }
  	 printf("%lld\n",res);
  
      return 0;
  }