leetCode(49):Count Primes

Description:

Count the number of prime numbers less than a non-negative number, n.

推断一个数是否是质数主要有下面几种方法：

1）直接用该数除于全部小于它的数（非0。1），假设均不能被它整除，则其是质数。

2）除以小于它一半的数。由于大于其一半必然是无法整除。假设均不能被它整除。则其是质数；

3）除以小于sqrt(a)的数，原因例如以下：

除了sqrt(a)之外,其它的两数乘积为a的,一定是比个比sqrt(a)小,一个比sqrt(a)大。所以推断到sqrt(a)就能够了,由于还有一半就是刚才做除法的商的那部份。

4）除以小于sqrt(a)的质数就可以。

  由于质数不能被除自身和1外的全部数整除。非质数则不然，且其必定能被某一质数整除。假设一个数能被某一非质数整除。则其必定能被组成这一非质数的最小质数整数。

从上能够看出，推断一个数是否是质数。其计算量取决于整除的个数。上述四种方法，除数逐渐变少。

通过以上分析，这是本人自己的程序：

class Solution {
public:
    int countPrimes(int n) {
        if (n == 0 || n == 1 || n==2)
    		return 0;

    	vector<int> vec;//质数容器

    	vec.push_back(2);
    	for (int i = 3; i < n; ++i)
    	{
    		int j = 0;
    		int length=vec.size();
    		for (; j<length && vec[j]*vec[j]<i; ++j)//除数是小于sqrt(i)的全部质数
    		{
    			if (i%vec[j] == 0)
    				break;
    		}
    		if (vec[j]*vec[j]>i)
    			vec.push_back(i);
    	}
    	return vec.size();
    }
};

以下是在网上找到一个程序，非常具体：

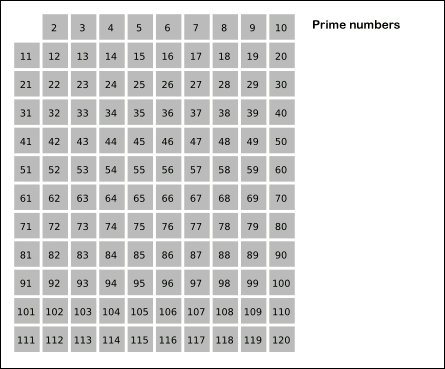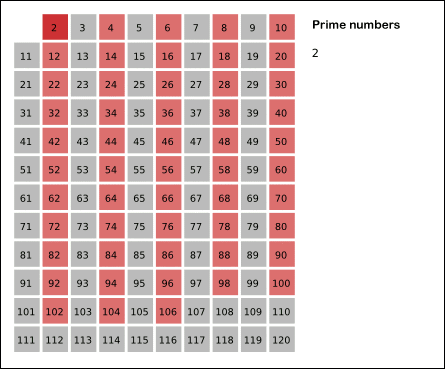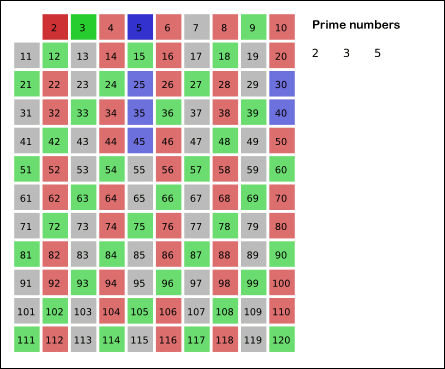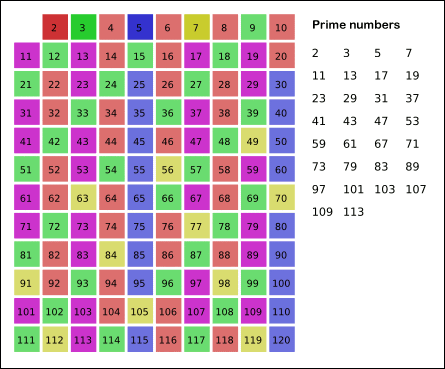
这道题给定一个非负数n，让我们求小于n的质数的个数，题目中给了充足的提示。解题方法就在第二个提示埃拉托斯特尼筛法Sieve
of Eratosthenes中。这个算法的步骤例如以下图所看到的，我们从2開始遍历到根号n，先找到第一个质数2，然后将其全部的倍数全部标记出来。然后到下一个质数3。标记其全部倍数。一次类推，直到根号n，此时数组中未被标记的数字就是质数。我们须要一个n-1长度的bool型数组来记录每一个数字是否被标记，长度为n-1的原因是题目说是小于n的质数个数。并不包含n。

然后我们用两个for循环来实现埃拉托斯特尼筛法，难度并非非常大。代码例如以下所看到的：

class Solution {
public:
    int countPrimes(int n) {
        vector<bool> num(n - 1, true);
        num[0] = false;
        int res = 0, limit = sqrt(n);
        for (int i = 2; i <= limit; ++i) {
            if (num[i - 1]) {
                for (int j = i * i; j < n; j += i) {//为什么要从i*i開始，由于小于i*i的数可能已经被小于i的数整除了。假设没有，那它就是质数
                    num[j - 1] = false;
                }
            }
        }
        for (int j = 0; j < n - 1; ++j) {
            if (num[j]) ++res;
        }
        return res;
    }
};