hdu6074[并查集+LCA+思维] 2017多校4

看了标答感觉思路清晰了许多，用并查集来维护全联通块的点数和边权和。

用另一个up[]数组（也是并查集）来保证每条边不会被重复附权值，这样我们只要将询问按权值从小到大排序，一定能的到最小的边权和与联通块中的点数。

下面是过程分析。过程中一共运用了两个并查集。

[数据]
1
10 3
1 2
1 3
2 4
2 5
3 6
3 7
4 8
5 9
5 10
9 6 3 1 50
1 4 2 5 20
9 10 8 10 40
[分析]
首先我们将图构建出来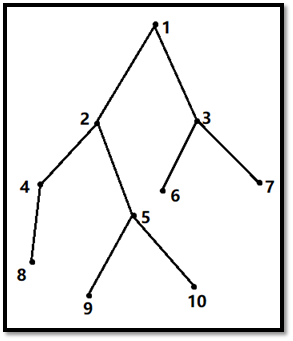

我们将m次询问按权值从小到大排序后：

1 4 2 5 20
9 10 8 10 40
9 6 3 1 50

对于(1,4) (2,5) 20 这一组询问:

　　我们做如下操作, 首先查找1,4的lca(此例中lca即为1)，先合并4与lca之间的边：利用up[] 往上合并直到超过或者到达lca。在往上合并的过程中，可以把下面的边权合并至父节点。利用up[]的路径压缩性质,下次再访问up[4]，up[2]时会直接跳至1。此时cnt[1]已经加上了了从4到1路径中点的个数，cost[1]里面加上了了从4到1所有边权的和(利用一个并查集维护)。经过上述操作 parent[4] = parent[2] = 1， up[4] = up[2] = 1。同理我们继续合并1与lca(lca=1);

　　合并完之后，我们处理2，5这一对点，lca(2,5)=2; 首先我们合并5与lca之间的边，利用up[5]=5，我们在并查集中合并5与anc[5][0](是倍增lca，所以即为5的2^0级父节点) 我们知道anc[5][0]=2;所以在merge(5,anc[5][0],cost)的过程中，实际上是把边权和点数附加到了anc[5][0]的父节点上，即1(详见上一段操作中，2已经被合并至1上，即parent[2] = 1)，也就是说cnt[1]记录了5到lca路径中点的个数，cost[1]里面加上了5到lca所有边权的和，且经过这一段操作，up[5] = 1， parent[5] = 1。同理，我们继续合并2与lca(lca=2)。

对于9 10 8 10 40 这一组询问，

　　lca[9,10]=5,照上面的过程合并中将{1，2，4，5，9 ，10}都加进了父节点中，在处理8，10(lca(8,10)=2)中我们发现，在8，10路径之中有已经被赋值的边，且肯定比当前要赋的值(因为询问是按权值从小到大来的)小，这个时候 up数组 就要发挥作用了。第一次询问up[8] = 8,合并[8，anc[8][0]=4]，下一次询问up[8] = 4,合并[4 , anc[4][0]=2]，这个时候就，因为parent[2] = 1，所以8被记录进了cnt[1]，且边权也被加到了cost[1],再之后up[4] = 1，直接跳到了lca=2节点的上面，提示我们不需要再继续合并了(deep[1]<deep[2])。之后都进行相似的操作就可以得出答案 [9, 280]。

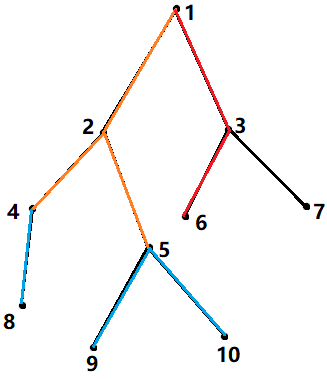就像这样 ， 9 10 8 10 50这组询问实际上只将3条蓝色边赋值成了40，橙色的边早已赋值成了更优的20。

　　实际上，up数组保存的是与自己不在一个联通块(另一个并查集维护联通快性质)的且最接近自己的父节点，比如此例子中up[4]=1，up[8]=1，（此例子中较特殊，已经不存在深度更小的顶点了，假如第一组询问换成2 4 2 5 20，那么up[2] = up[4] = 1）直接跳到了最高点，提示我们不需要继续合并了，之后的边肯定都已经赋过值了，且值更优。

/*hdu6074[并查集+LCA+思维] 2017多校4*/
#include <bits/stdc++.h>
using namespace std;
typedef long long LL;
struct node {
    int a, b, c, d;
    LL w;
    bool operator < (node& x) {
        return w < x.w;
    }
} q[];
vector<int> G[];
int T, m, n;
int up[], p[], deep[], anc[][];
LL cnt[], cost[];
void init() {
    for (int i = ; i <= n; i++) {
        G[i].clear();
    }
    memset(deep, , sizeof(deep));
    for (int i = ; i <= n; i++)
        cost[i]=,cnt[i]=,p[i] = up[i] = i;
}
int getfa(int x) {
    return p[x] == x ? x : p[x] = getfa(p[x]);
}
int getup(int x) {
    return up[x] == x ? x : up[x] = getup(up[x]);
}
void dfs(int u, int fa) {
    anc[u][] = fa;
    for (int i = ; i < ; i++) {
        anc[u][i] = anc[anc[u][i - ]][i - ];
    }
    for (int i = ; i < (int)G[u].size(); i++) {
        int v = G[u][i];
        if (v == fa) continue;
        deep[v] = deep[u] + ;
        dfs(v, u);
    }
}
int lca(int u, int v) {
    if (deep[u] < deep[v]) swap(u, v);
    for (int i = ; ~i; i--) {
        if (deep[v] <= deep[anc[u][i]]) {
            u = anc[u][i];
        }
    }
    if (u == v) return u;
    for (int i = ; ~i; i--) {
        if (anc[u][i] != anc[v][i]) {
            u = anc[u][i];
            v = anc[v][i];
        }
    }
    return anc[u][];
}
void merge(int u, int v, LL w) {
    int x = getfa(u);
    int y = getfa(v);
    if (x == y) return;
    p[x] = y;
    cnt[y] += cnt[x];
    cost[y] += cost[x] + w;
}
void jump(int u, int v, LL w) {
    for (;;) {
        u = getup(u);
        if (deep[u] <= deep[v]) return;
        merge(u, anc[u][], w);
        up[u] = anc[u][];
    }
}
void solve() {
    dfs(, );
    sort(q + , q + m + );
    for (int i = ; i <= m; i++) {
        int k = lca(q[i].a, q[i].b);
        //cout << q[i].w << endl;
        jump(q[i].a, k, q[i].w);
        jump(q[i].b, k, q[i].w);
        k = lca(q[i].c, q[i].d);
        jump(q[i].c, k, q[i].w);
        jump(q[i].d, k, q[i].w);
        merge(q[i].a, q[i].c, q[i].w);
    }
    printf("%lld %lld\n",cnt[getfa()],cost[getfa()]);
}
int main() {
    scanf("%d", &T);
    while (T--) {
        int u, v;
        scanf("%d%d", &n, &m);
        init();
        for (int i = ; i < n; i++) {
            scanf("%d%d", &u, &v);
            G[u].push_back(v);
            G[v].push_back(u);
        }
        for (int i = ; i <= m; i++) {
            scanf("%d%d%d%d%lld", &q[i].a, &q[i].b, &q[i].c, &q[i].d, &q[i].w);
        }
        solve();
    }
    return ;
}
/*
2
10 3
1 2
1 3
2 4
2 5
3 6
3 7
4 8
5 9
5 10
1 4 2 5 20
9 10 8 10 40
9 6 3 1 50
10 1
1 2
1 3
2 4
2 5
3 6
3 7
4 8
5 9
5 10
1 7 9 10 20
*/

顺便附上st表lca的版本.

#include <bits/stdc++.h>
using namespace std;
const int MAX_N = ;
typedef long long LL;

struct node {
    int a, b, c, d;
    LL w;
    bool operator < (node& x) {
        return w < x.w;
    }
} q[];
int pos[MAX_N], dep[MAX_N << ], h[MAX_N << ], up[MAX_N], p[MAX_N], s[MAX_N];
int st[MAX_N << ][], len[MAX_N << ], tot = , deep[MAX_N];
vector<int> G[MAX_N];
int T, m, n;
LL cnt[MAX_N], cost[MAX_N];
void init() {
    tot = ;
    for (int i = ; i <= n; i++) {
        G[i].clear();
    }
    for (int i = ; i <= n; i++)
        cost[i] = , cnt[i] = , p[i] = up[i] = i;
}
int getfa(int x) {
    return p[x] == x ? x : p[x] = getfa(p[x]);
}
int getup(int x) {
    return up[x] == x ? x : up[x] = getup(up[x]);
}
void dfs(int u, int fa, int dps) {
    s[u] = fa;
    deep[u]=dps;
    h[++tot] = u, pos[u] = tot, dep[tot] = dps;
    for (int i = ; i < (int)G[u].size(); i++) {
        int v = G[u][i];
        if (v == fa) continue;
        dfs(v, u, dps + );
        h[++tot] = u;
        dep[tot] = dps;
    }
}
void st_init() {
    len[] = -;
    for (int i = ; i <= tot; i++) {
        st[i][] = i;
        len[i] = ((i & (i - )) == ) ? len[i - ] +  : len[i - ];
    }
    for (int j = ; j <= len[tot]; j++) {
        for (int i = ; i + ( << j) -  < tot; i++) {
            int a = st[i][j - ], b = st[i + ( << (j - ))][j - ];
            st[i][j] = dep[a] < dep[b] ? a : b;
        }
    }
}
int query(int l, int r) {
    int k = len[r - l + ];
    int a = st[l][k], b = st[r - ( << k) + ][k];
    return dep[a] < dep[b] ? a : b;
}
int lca(int u, int v) {
    int l = pos[u], r = pos[v];
    if (l > r) swap(l, r);
    return h[query(l, r)];
}
void merge(int u, int v, LL w) {
    int x = getfa(u);
    int y = getfa(v);
    if (x == y) return;
    p[x] = y;
    cnt[y] += cnt[x];
    cost[y] += cost[x] + w;
}
void jump(int u, int v, LL w) {
    for (;;) {
        u = getup(u);
        if (deep[u] <= deep[v]) return;
        merge(u, s[u], w);
        up[u] = s[u];
        //cout << u << ' '  << s[u] << endl;
    }
}
void solve() {
    dfs(, , );
    st_init();
    sort(q + , q + m + );
    for (int i = ; i <= m; i++) {
        int k = lca(q[i].a, q[i].b);
        //cout << k << endl;
        jump(q[i].a, k, q[i].w);
        jump(q[i].b, k, q[i].w);
        k = lca(q[i].c, q[i].d);
        jump(q[i].c, k, q[i].w);
        jump(q[i].d, k, q[i].w);
        merge(q[i].a, q[i].c, q[i].w);
    }
    printf("%lld %lld\n", cnt[getfa()], cost[getfa()]);
}
int main() {
    scanf("%d", &T);
    while (T--) {
        int u, v;
        scanf("%d%d", &n, &m);
        init();
        for (int i = ; i < n; i++) {
            scanf("%d%d", &u, &v);
            G[u].push_back(v);
            G[v].push_back(u);
        }
        for (int i = ; i <= m; i++) {
            scanf("%d%d%d%d%lld", &q[i].a, &q[i].b, &q[i].c, &q[i].d, &q[i].w);
        }
        solve();
    }
    return ;
}