T分布(T-Distribution)

1、What is the T Distribution?

T分布(也叫Student 's T分布)是一组与正态分布曲线几乎相同的分布，只是更短更胖一点。当有小样本时，使用t分布而不是正态分布。样本容量越大，t分布越接近正态分布。事实上，对于大于20的样本容量(如自由度)，其分布与正态分布几乎完全相同。

The T distribution (also called Student’s T Distribution) is a family of distributions that look almost identical to the normal distribution curve, only a bit shorter and fatter. The t distribution is used instead of the normal distribution when you have small samples (for more on this, see: t-score vs. z-score). The larger the sample size, the more the t distribution looks like the normal distribution. In fact, for sample sizes larger than 20 (e.g. more degrees of freedom), the distribution is almost exactly like the normal distribution.

2、How to Calculate the Score for a T Distribution

当你看t分布表时，你会发现你需要知道df。这意味着“自由度”，也就是样本容量减去1。

When you look at the t-distribution tables, you’ll see that you need to know the “df.” This means “degrees of freedom” and is just the sample size minus one.

方法：

Step 1: Subtract one from your sample size. This will be your degrees of freedom.
Step 2: Look up the df in the left hand side of the t-distribution table. Locate the column under your alpha level(the alpha level is usually given to you in the question).

3、Uses

T分布(以及相关的T得分)用于假设检验，当您想知道您应该接受或拒绝原假设时

The T Distribution (and the associated t scores), are used in hypothesis testing when you want to figure out if you should accept or reject the null hypothesis.

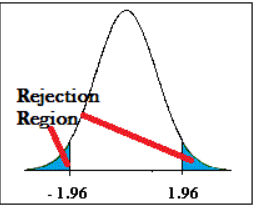
图中的中心区域是接收区域，尾部是拒绝区域。在这个双尾测试的特殊图表中，拒绝区域是蓝色的。尾部区域可以用z分数或t分数来描述。例如，左边的图像显示了5%(每边2.5%)尾部的区域。z分数是1.96(来自z表格)，也就是离 平均值1.96个标准差。如果z小于-1.96或大于1.96，零假设将被拒绝。

The central region on this graph is the acceptance area and the tail is the rejection region, or regions. In this particular graph of a two tailed test, the rejection region is shaded blue. The area in the tail can be described with z-scores or t-scores. For example, the image to the left shows an area in the tails of 5% (2.5% each side). The z-score would be 1.96 (from the z-table), which represents 1.96 standard deviations from the mean. The null hypothesis will be rejected if z is less than -1.96 or greater than 1.96

一般而言，当样本量较小(小于30)或不知道总体标准差时，就会使用该分布。出于实际目的(即在现实世界中)，情况几乎总是如此。不像初级统计课，现实生活中会用到它而不是正态分布。如果样本容量足够大，这两个分布实际上是相同的。

In general, this distribution is used when you have a small sample size (under 30) or you don’t know the population standard deviation. For practical purposes (i.e. in the real world), this is nearly always the case. So, unlike in your elementary statistics class, you’ll likely be using it in real life situations more than the normal distribution. If the size of your sample is large enough, the two distributions are practically the same.

4、参考文献

https://www.statisticshowto.datasciencecentral.com/probability-and-statistics/t-distribution/