POJ 2976 Dropping tests（01分数规划）

　　　　　　　　　　　　　　　　　　　　　　　　　　　　　　Dropping tests

Time Limit: 1000MS		Memory Limit: 65536K
Total Submissions:17069		Accepted: 5925

Description

In a certain course, you take n tests. If you get a_i out of b_i questions correct on test i, your cumulative average is defined to be

.

Given your test scores and a positive integer k, determine how high you can make your cumulative average if you are allowed to drop any k of your test scores.

Suppose you take 3 tests with scores of 5/5, 0/1, and 2/6. Without dropping any tests, your cumulative average is 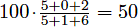. However, if you drop the third test, your cumulative average becomes .

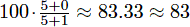Input

The input test file will contain multiple test cases, each containing exactly three lines. The first line contains two integers, 1 ≤ n ≤ 1000 and 0 ≤ k < n. The second line contains n integers indicating a_i for all i. The third line contains n positive integers indicating b_i for all i. It is guaranteed that 0 ≤ a_i ≤ b_i ≤ 1, 000, 000, 000. The end-of-file is marked by a test case with n = k = 0 and should not be processed.

Output

For each test case, write a single line with the highest cumulative average possible after dropping k of the given test scores. The average should be rounded to the nearest integer.

Sample Input

3 1
5 0 2
5 1 6
4 2
1 2 7 9
5 6 7 9
0 0

Sample Output

83
100

Hint

To avoid ambiguities due to rounding errors, the judge tests have been constructed so that all answers are at least 0.001 away from a decision boundary (i.e., you can assume that the average is never 83.4997).

思路：

为了做POJ 2728 ，先做此题，作为练习。

此题有一个算法，叫做01分数规划，目标就是求给定条件下的平均值最大值。平均值最大值是不可以直接有各个平均值累和的，这是因为S(a)/S(b）----s表示求和，这个式子就是平均值。

对于这个式子，很明显是除法运算，所以S(a)/S(b)并不会等于S(a/b),这是显而易见的，而我们现在要做的就是，找出这样一个x，使得S(a)/S(b)与x作比较，并对x进行调整，直到找出满足条件的临界点为止。此时，为了方便计算，我们可以做一点变形，就是S(a)与S(b)*x比较，在这种情况下，我们就可以求出每一点的a-b*x，再进行累和了，因为现在是减法运算。

代码

 #include<iostream>
 #include<algorithm>
 #include<cstdio>
 using namespace std;
 typedef long long ll;
 ll a[],b[];
 int n,k;
 const double eps = 1e-;
 double ans[];
 double num(double m)
 {
     for(int i=;i<=n;i++){
         ans[i]=a[i]-m*b[i];
     }
     sort(ans+,ans++n);
     double sum=;
     for(int i=n;i>=k+;i--){sum+=ans[i];}
     return sum>=;
 }

 int main()
 {
     while(scanf("%d%d",&n,&k)!=EOF&&n+k){
         for(int i=;i<=n;i++){
             scanf("%lld",&a[i]);
         }
         for(int i=;i<=n;i++){
             scanf("%lld",&b[i]);
         }

         double mid,l,r;
         l=,r=;
         while(r-l>eps){
             mid=(l+r)/;
             if(num(mid)){l=mid;}
             else r=mid;
         }
         printf("%.0f\n",mid*);
     }
 }