Mayor's posters POJ - 2528(线段树 + 离散化)

Mayor's posters

Time Limit: 1000MS		Memory Limit: 65536K
Total Submissions: 74745		Accepted: 21574

Description

The citizens of Bytetown, AB, could not stand that the candidates in the mayoral election campaign have been placing their electoral posters at all places at their whim. The city council has finally decided to build an electoral wall for placing the posters and introduce the following rules:

• Every candidate can place exactly one poster on the wall.
• All posters are of the same height equal to the height of the wall; the width of a poster can be any integer number of bytes (byte is the unit of length in Bytetown).
• The wall is divided into segments and the width of each segment is one byte.
• Each poster must completely cover a contiguous number of wall segments.

They have built a wall 10000000 bytes long (such that there is enough place for all candidates). When the electoral campaign was restarted, the candidates were placing their posters on the wall and their posters differed widely in width. Moreover, the candidates started placing their posters on wall segments already occupied by other posters. Everyone in Bytetown was curious whose posters will be visible (entirely or in part) on the last day before elections. 
Your task is to find the number of visible posters when all the posters are placed given the information about posters' size, their place and order of placement on the electoral wall. 

Input

The first line of input contains a number c giving the number of cases that follow. The first line of data for a single case contains number 1 <= n <= 10000. The subsequent n lines describe the posters in the order in which they were placed. The i-th line among the n lines contains two integer numbers l_i and ri which are the number of the wall segment occupied by the left end and the right end of the i-th poster, respectively. We know that for each 1 <= i <= n, 1 <= l_i <= ri <= 10000000. After the i-th poster is placed, it entirely covers all wall segments numbered l_i, l_i+1 ,... , ri.

Output

For each input data set print the number of visible posters after all the posters are placed.

The picture below illustrates the case of the sample input.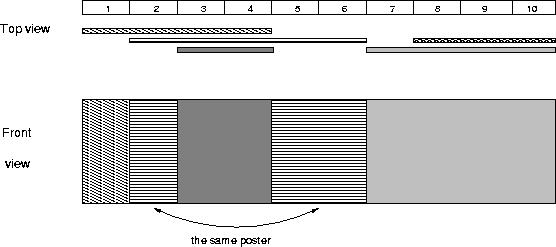 

Sample Input

1
5
1 4
2 6
8 10
3 4
7 10

Sample Output

4

解析：

题目大意：给你一个无限长的板子，然后依次往上面贴n张等高的海报，问你最后能看到多少张海报。

思路分析：线段树区间更新问题，但是要注意，给的长度的可能非常大，有1e9，不加处理直接维护一个线段树肯定会

MLE,TLE,但是我们注意到一共最多只有2e4个点，因此我们可以用离散化的思想先对区间进行预处理，所谓的离散化，

在我理解看来就是将一个很大的区间映射为一个很小的区间，而不改变原有的大小覆盖关系，但是注意简单的离散化可能

会出现错误，给出下面两个简单的例子应该能体现普通离散化的缺陷:
例子一:1-10 1-4 5-10
例子二:1-10 1-4 6-10
普通离散化后都变成了[1,4][1,2][3,4]
线段2覆盖了[1,2],线段3覆盖了[3,4],那么线段1是否被完全覆盖掉了呢?
例子一是完全被覆盖掉了,而例子二没有被覆盖

解决的办法则是对于距离大于1的两相邻点，中间再插入一个点，本题还用到了Lazy标记的思想

直接更新区间进行标记而先不对子节点进行处理，如果需要往下更新再将标记下传一层。

#include <iostream>
#include <cstdio>
#include <cstring>
#include <queue>
#include <algorithm>
#include <cmath>
#define mem(a, b) memset(a, b, sizeof(a))
using namespace std;
const int maxn = , INF = 0x7fffffff;
int t[maxn], a1[maxn], a2[maxn], vis[maxn];
int a, b, x, y, ans;
 struct node{
    int l, r, w, f;
 }Node[maxn*];

 void build(int k, int ll, int rr)
 {
     Node[k].l = ll, Node[k].r = rr;
     Node[k].w = ;
     if(Node[k].l == Node[k].r) return;
     int m = (ll + rr) / ;
     build(k*, ll, m);
     build(k*+, m+, rr);
 }

 void down(int k)
 {
     Node[k*].f = Node[k].f;
     Node[k*+].f = Node[k].f;
     Node[k*].w = Node[k].f;
     Node[k*+].w = Node[k].f;
     Node[k].f = ;
 }

void chinter(int k)
{
    if(Node[k].l >= a && Node[k].r <= b)
    {
        Node[k].w =  y;
        Node[k].f = y;
        return;
    }
    if(Node[k].f) down(k);
    int m = (Node[k].l + Node[k].r) / ;
    if(a <= m) chinter(k*);
    if(b > m) chinter(k*+);
 //   Node[k].w = Node[k*2].w + Node[k*2+1].w;
}

void qp(int k)
{
    if(Node[k].l == Node[k].r)
    {
        ans = Node[k].w;
        return;
    }
    if(Node[k].f) down(k);
    int m = (Node[k].l + Node[k].r) / ;
    if(a <= m) qp(k*);
    else qp(k*+);
}

int main()
{
    int T;
    scanf("%d",&T);
    while(T--)
    {
        mem(vis, );
        int n, cnt = , ret = ;
        scanf("%d",&n);
        for(int i=; i<=n; i++)
        {
            scanf("%d%d",&a1[i],&a2[i]);
            t[cnt++] = a1[i];
            t[cnt++] = a2[i];
        }
        sort(t+, t+cnt);
        int m = unique(t+, t+cnt) - (t+);
        int len = m;
        for(int i=; i<len; i++)
            if(t[i+] - t[i] > ) t[++m] = t[i] + ;
        sort(t+, t+m+);
        build(, , m);
        for(int i=; i<=n; i++)
        {
            a = lower_bound(t+, t+m+, a1[i]) - t;
            b = lower_bound(t+, t+m+, a2[i]) - t;
            y = i;
            chinter();
        }
        for(int i=; i<=m; i++)
        {
            a = i;
            qp();
        //    cout<< ans;
            if(!vis[ans] && ans != )
            {
                vis[ans] = ;
                ret++;
            }
        }
    //    cout<< endl;
        printf("%d\n",ret);
    }
    return ;
}