【学习笔记】Splay

\(\texttt{0x01}\) 前言

Splay 树（伸展树）是一棵二叉搜索树，由 Daniel Sleator 和 Robert Tarjan 于 1985 年发明。它凭借旋转可以有 $O(\log n) $ 插入，删除等的较优秀的时间复杂度。

前置芝士：普通二叉排序树。

推荐博客：

• https://www.cnblogs.com/artalter/p/15916693.html
• https://www.cnblogs.com/artalter/p/15922513.html

\(\texttt{0x02}\) 如何构造一棵 Splay

我们定义一个结构体：

#define val(x) t[x].val
#define ls(x) t[x].ch[0]
#define rs(x) t[x].ch[1]
#define son(x,nxt) t[x].ch[nxt]
#define fa(x) t[x].fa
#define cnt(x) t[x].cnt
#define siz(x) t[x].siz
struct node{
	int val,fa,ch[2],siz,cnt;
}t[N];
int root,tot;

其中构造一个新节点的函数长这样：

void newPoint(int val,int fa,int nxt){ //值为val，父节点为fa，为fa的nxt儿子
	tot++;
	fa(tot)=fa; cnt(tot)=siz(tot)=1; val(tot)=val;
	son(fa,nxt)=tot;
}

\(\texttt{0x03}\) which / pushup / connect

which 的作用是判断 \(x\) 是其父节点的左节点（\(0\)）还是右节点（\(1\)），代码很好写：

bool which(int x){
	return rs(fa(x))==x;
}

---

pushup 的作用是维护当前节点的 \(siz\) 信息，和线段树的 pushup 性质差不多，代码：

void pushup(int x){
	siz(x)=siz(ls(x))+siz(rs(x))+cnt(x);//记得加上当前节点的cnt
}

---

connect 的作用是把 \(x\) 变成 \(y\) 的 \(nxt\) 儿子，无需考虑覆盖的问题，代码也很简洁：

void connect(int x,int y,int nxt){
	son(y,nxt)=x;
	fa(x)=y;
}

\(\texttt{0x04}\) rotate

Splay 的核心操作：旋转。

放两张动图：

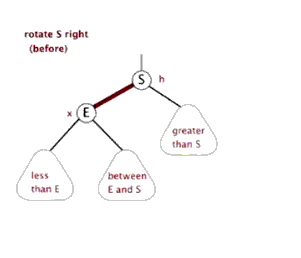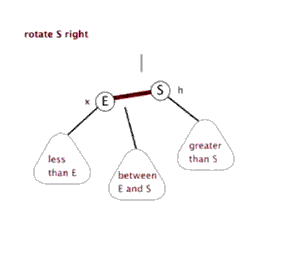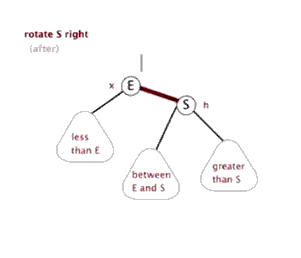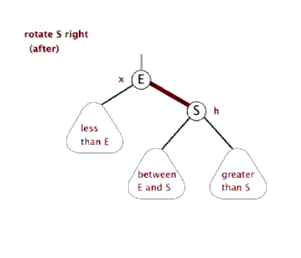
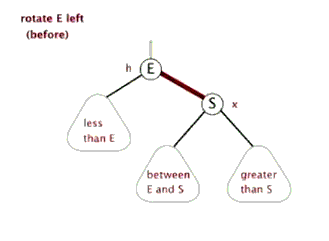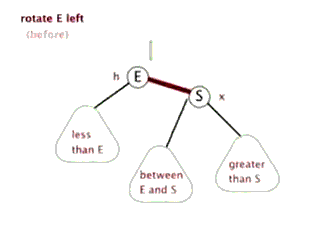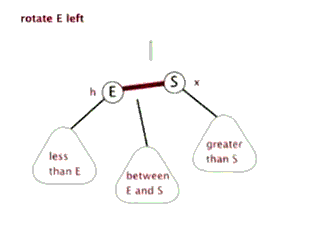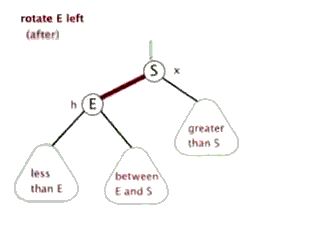
我们会发现：右旋时，E 节点要到 S 节点的位置上，那么 E 节点的右儿子因为它 \(\ge E\) 且 \(\le S\)，所以只能放在 S 节点的左儿子，然后要改变 E 和 S 的父子关系。最后别忘了因为有旋转，所以要自下而上更新节点信息。

左旋同理。

代码：

void rotate(int x){
	int y=fa(x),z=fa(y);
	int fx=which(x),fy=which(y);

	connect(son(x,fx^1),y,fx); //如果x是左儿子，改变它右儿子的位置，反之同理
	connect(y,x,fx^1); //把y接到x的缺失的那一棵子树上
	connect(x,z,fy); //把x接到y的父节点上去

	pushup(y); pushup(x); //别搞错顺序
}

\(\texttt{0x05}\) splay

Splay 树保证时间复杂度正确的核心操作，把 \(x\) 转到 \(y\) 的位置（\(y\) 通常为 \(root\)）。

有几点结论，难证但好记：

• 若 \(fa(x)=y\)，则单旋 \(x\)。
• 若 \(x\)、\(fa(x)\)、\(fa\left(fa(x)\right)\) 不在一条线上，则先单旋 \(fa(x)\)，再单旋 \(x\)。
• 否则旋转两次 \(x\)。

void splay(int x,int y){
	y=fa(y); //避免x=y时出现的错误
	while(fa(x)!=y){
		if(fa(fa(x))==y) // Case 1
			rotate(x);
		else if(which(x)==which(fa(x))) // Case 2
			rotate(fa(x)), rotate(x);
		else // Case 3
			rotate(x), rotate(x);
	}
	if(y==0){ // 如果y是根，把根变为x
		root=x;
		connect(x,0,1);
	}
}

\(\texttt{0x06}\) insert

与普通的二叉排序树基本一致。

• 如果树中已经有值了，则 \(cnt \gets cnt+1\)。
• 如果找到最后都没有值，建个新节点。

记得最后要 splay 一下，把这个点转到根节点。

void insert(int val){
	if(root==0){
		newPoint(val,0,1);
		root=tot;
		return;
	}
	int now=root;
	while(1){
		siz(now)++;
		if(val(now)==val){
			cnt(now)++;
			splay(now,root);
			return;
		}
		int nxt=val(now)<val, son=son(now,nxt);
		if(!son){
			newPoint(val,now,nxt);
			splay(tot,root);
			return;
		}
		now=son;
	}
}

\(\texttt{0x07}\) find

这一步操作是找到树中值为 \(val\) 的节点，并把它旋转到根节点，为 delete 操作做准备。

与普通二叉排序树也基本一致。

int find(int val){
	int now=root;
	while(1){
		if(!now)
			return 0;
		if(val(now)==val){
			splay(now,root);
			return now;
		}
		int nxt=val(now)<val, son=son(now,nxt);
		now=son;
	}
}

\(\texttt{0x08}\) delete

目的是删除树中值为 \(val\) 的节点。

先 find 这个节点，让他转到根，然后分类讨论。

1. 树中没有值为 \(val\) 的节点，删了个寂寞。

2. 树中值为 \(val\) 的节点有不止一个（即 \(cnt \ge 2\)），让 \(cnt \gets cnt-1\) 即可。

3. 这个节点没有左儿子（即根节点只有右子树），把右儿子设为根就行了。

4. 这个节点没有右儿子（即根节点只有左子树），把左儿子设为根就行了。

5. 这个节点（设为 \(x\)）有左右儿子，把它的左子树中值最大的（设为 \(y\)）splay 到根，然后现在的 Splay 树的根就是 \(y\)，左子树是原来的除 \(y\) 之外的左子树，右子树是 \(x\) 和之前的右子树。把之前的右子树 connect 到根就行了。

注意：这里的删除操作都没有回收编号。

void delet(int val){
	int now=find(val);
	if(!now) return;
	if(cnt(now)>1){
		cnt(now)--; siz(now)--;
		return;
	}
	if(!ls(now) && !rs(now)){
		root=0;
	}
	else if(!ls(now)){
		root=rs(root);
		fa(root)=0;
	}
	else if(!rs(now)){
		root=ls(root);
		fa(root)=0;
	}
	else{
		int pos=ls(now);
		while(rs(pos)) pos=rs(pos);

		splay(pos,root);
		connect(rs(now),pos,1);
		pushup(pos);
	}
}

\(\texttt{0x09}\) rnk & find_k

rnk 是返回值为 \(val\) 的数在树中的排名，find_k 是找到树中排名为 k 的数。

与二叉排序树基本完全相同。记得最后要把节点 splay 到根。

int rnk(int val){
	int now=root,s=0;
	while(now){
		if(val(now)==val){
			splay(now,root);
			return siz(ls(now))+1;
		}
		if(val(now)<val){
			s+=siz(ls(now))+cnt(now);
			now=rs(now);
		}
		else{
			now=ls(now);
		}
	}
	return s+1;
}
int find_k(int k){
	int now=root;
	while(1){
		int used=siz(now)-siz(rs(now));
		if(k>siz(ls(now)) && k<=used){
			break;
		}
		if(k>=used){
			k-=used;
			now=rs(now);
		}
		else{
			now=ls(now);
		}
	}
	splay(now,root);
	return val(now);
}

\(\texttt{0x0A}\) lower & upper

返回值为 \(val\) 的数的前驱和后继。

int lower(int val){
	int ans=-2147483647;
	int now=root;
	while(now){
		if(val(now)<val && val(now)>ans){
			ans=val(now);
		}
		if(val>val(now)){
			now=rs(now);
		}
		else{
			now=ls(now);
		}
	}
	return ans;
}
int upper(int val){
	int ans=2147483647;
	int now=root;
	while(now){
		if(val(now)>val && val(now)<ans){
			ans=val(now);
		}
		if(val<val(now)){
			now=ls(now);
		}
		else{
			now=rs(now);
		}
	}
	return ans;
}

\(\texttt{0x0B}\) 完整代码

#include<bits/stdc++.h>
using namespace std;
constexpr int N = 5e5+5;

template <typename T> void read(T &x){x=0; T f(0); char ch=getchar(); while(ch<'0'||ch>'9'){f|=ch=='-';ch=getchar();} while(ch>='0'&&ch<='9'){x=(x<<1)+(x<<3)+(ch^48); ch=getchar();} x=f?-x:x;}
template <typename T,typename ...Arg>void read(T& x,Arg& ...arg){read(x);read(arg...);}
template <typename T> inline void write(T x){static char buf[64]; static int tot(0); if(x<0) putchar('-'),x=-x; do buf[++tot]=(x%10)+48,x/=10; while(x); do putchar(buf[tot--]); while(tot);}
template <typename T> void write(T x,char c){static char buf[64]; static int tot(0); if(x<0) putchar('-'),x=-x; do buf[++tot]=(x%10)+48,x/=10; while(x); do putchar(buf[tot--]); while(tot); putchar(c);}

class Splay{
#define val(x) t[x].val
#define ls(x) t[x].ch[0]
#define rs(x) t[x].ch[1]
#define son(x,nxt) t[x].ch[nxt]
#define fa(x) t[x].fa
#define cnt(x) t[x].cnt
#define siz(x) t[x].siz
private:
	struct node{
		int val,fa,ch[2],siz,cnt;
	}t[N];
	int root,tot;
public:
	bool which(int x){
		return rs(fa(x))==x;
	}
	void pushup(int x){
		siz(x)=siz(ls(x))+siz(rs(x))+cnt(x);
	}
	void connect(int x,int y,int nxt){
		son(y,nxt)=x;
		fa(x)=y;
	}
	void rotate(int x){
		int y=fa(x),z=fa(y);
		int fx=which(x),fy=which(y);

		connect(son(x,fx^1),y,fx);
		connect(y,x,fx^1);
		connect(x,z,fy);

		pushup(y); pushup(x);
	}
	void splay(int x,int y){
		y=fa(y);
		while(fa(x)!=y){
			if(fa(fa(x))==y)
				rotate(x);
			else if(which(x)==which(fa(x)))
				rotate(fa(x)), rotate(x);
			else
				rotate(x), rotate(x);
		}
		if(y==0){
			root=x;
			connect(x,0,1);
		}
	}
	void newPoint(int val,int fa,int nxt){
		tot++;
		fa(tot)=fa; cnt(tot)=siz(tot)=1; val(tot)=val;
		son(fa,nxt)=tot;
	}
	void insert(int val){
		if(root==0){
			newPoint(val,0,1);
			root=tot;
			return;
		}
		int now=root;
		while(1){
			siz(now)++;
			if(val(now)==val){
				cnt(now)++;
				splay(now,root);
				return;
			}
			int nxt=val(now)<val, son=son(now,nxt);
			if(!son){
				newPoint(val,now,nxt);
				splay(tot,root);
				return;
			}
			now=son;
		}
	}
	int find(int val){
		int now=root;
		while(1){
			if(!now)
				return 0;
			if(val(now)==val){
				splay(now,root);
				return now;
			}
			int nxt=val(now)<val, son=son(now,nxt);
			now=son;
		}
	}
	void delet(int val){
		int now=find(val);
		if(!now) return;
		if(cnt(now)>1){
			cnt(now)--; siz(now)--;
			return;
		}
		if(!ls(now) && !rs(now)){
			root=0;
		}
		else if(!ls(now)){
			root=rs(root);
			fa(root)=0;
		}
		else if(!rs(now)){
			root=ls(root);
			fa(root)=0;
		}
		else{
			int pos=ls(now);
			while(rs(pos)) pos=rs(pos);

			splay(pos,root);
			connect(rs(now),pos,1);
			pushup(pos);
		}
	}
	int rnk(int val){
		int now=root,s=0;
		while(now){
			if(val(now)==val){
				splay(now,root);
				return siz(ls(now))+1;
			}
			if(val(now)<val){
				s+=siz(ls(now))+cnt(now);
				now=rs(now);
			}
			else{
				now=ls(now);
			}
		}
		return s+1;
	}
	int find_k(int k){
		int now=root;
		while(1){
			int used=siz(now)-siz(rs(now));
			if(k>siz(ls(now)) && k<=used){
				break;
			}
			if(k>=used){
				k-=used;
				now=rs(now);
			}
			else{
				now=ls(now);
			}
		}
		splay(now,root);
		return val(now);
	}
	int lower(int val){
		int ans=-2147483647;
		int now=root;
		while(now){
			if(val(now)<val && val(now)>ans){
				ans=val(now);
			}
			if(val>val(now)){
				now=rs(now);
			}
			else{
				now=ls(now);
			}
		}
		return ans;
	}
	int upper(int val){
		int ans=2147483647;
		int now=root;
		while(now){
			if(val(now)>val && val(now)<ans){
				ans=val(now);
			}
			if(val<val(now)){
				now=ls(now);
			}
			else{
				now=rs(now);
			}
		}
		return ans;
	}
}tr;

int n,opt,val;

int main(){
	read(n);
	while(n--){
		read(opt,val);
		int ans;
		switch(opt){
			case 1:{
				tr.insert(val);
				break;
			}
			case 2:{
				tr.delet(val);
				break;
			}
			case 3:{
				ans=tr.rnk(val);
				break;
			}
			case 4:{
				ans=tr.find_k(val);
				break;
			}
			case 5:{
				ans=tr.lower(val);
				break;
			}
			case 6:{
				ans=tr.upper(val);
				break;
			}
		}
		if(opt>2) write(ans,'\n');
	}
}