POJ——3070Fibonacci（矩阵快速幂）

Fibonacci

Time Limit: 1000MS		Memory Limit: 65536K
Total Submissions: 12329		Accepted: 8748

Description

In the Fibonacci integer sequence, F₀ = 0, F₁ = 1, and F_n = F_{n − 1} + F_{n − 2} for n ≥ 2. For example, the first ten terms of the Fibonacci sequence are:

0, 1, 1, 2, 3, 5, 8, 13, 21, 34, …

An alternative formula for the Fibonacci sequence is

.

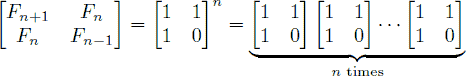Given an integer n, your goal is to compute the last 4 digits of F_n.

Input

The input test file will contain multiple test cases. Each test case consists of a single line containing n (where 0 ≤ n ≤ 1,000,000,000). The end-of-file is denoted by a single line containing the number −1.

Output

For each test case, print the last four digits of F_n. If the last four digits of F_n are all zeros, print ‘0’; otherwise, omit any leading zeros (i.e., print F_n mod 10000).

Sample Input

0
9
999999999
1000000000
-1

Sample Output

0
34
626
6875

Hint

As a reminder, matrix multiplication is associative, and the product of two 2 × 2 matrices is given by

.

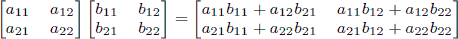Also, note that raising any 2 × 2 matrix to the 0th power gives the identity matrix:

.

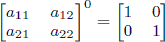看了很多关于矩阵快速幂的题解，感觉矩阵快速幂得到的是一个含有多个项的矩阵，而答案只是其中一项，而且之还需要特判指数。入门的矩阵快速幂题。感受一下再写其它的题目。拿这题为例。题目中给出的项从0开始，F₀=0，F₁=1，F₂=1，F₃=2。。就是一个斐波那契的数列。由于用矩阵，那至少要两项来组成矩阵，根据他的递推公式。可以得到

[F_n，F_n-1]=[1*F_n-1+1*F_n-2，1*F_n-1+0*F_n-2]

代码：

#include<iostream>
#include<algorithm>
#include<cstdlib>
#include<sstream>
#include<cstring>
#include<cstdio>
#include<string>
#include<deque>
#include<stack>
#include<cmath>
#include<queue>
#include<set>
#include<map>
using namespace std;
typedef long long LL;
#define INF 0x3f3f3f3f
struct mat
{
	LL m[2][2];
	mat(){memset(m,0,sizeof(m));}
};
mat cheng(mat a,mat b)
{
	mat c;
	for (int i=0 ;i<2; i++)
	{
		for (int j=0; j<2; j++)
		{
			for (int k=0; k<2; k++)
			{
				c.m[i][j]+=(a.m[i][k]*b.m[k][j])%10000;
			}
		}
	}
	return c;
}
mat zxc(mat a,LL b)
{
	mat c;
	c.m[0][0]=c.m[1][1]=1;
	while (b!=0)
	{
		if(b&1)
			c=cheng(c,a);
		a=cheng(a,a);
		b>>=1;
	}
	return c;
}
int main(void)
{
	LL n;
	while (cin>>n&&n!=-1)
	{
		mat one;
		if(n==0)
		{
			cout<<0<<endl;
			continue;
		}
		else if(n==1)
		{
			cout<<1<<endl;
			continue;
		}
		one.m[0][0]=one.m[1][0]=one.m[0][1]=1;
		one=zxc(one,n-1);
		cout<<one.m[0][0]%10000<<endl;;
	}
	return 0;
}