POJ-1681
| Time Limit: 1000MS | Memory Limit: 10000K | |
| Total Submissions: 4839 | Accepted: 2350 |
Description
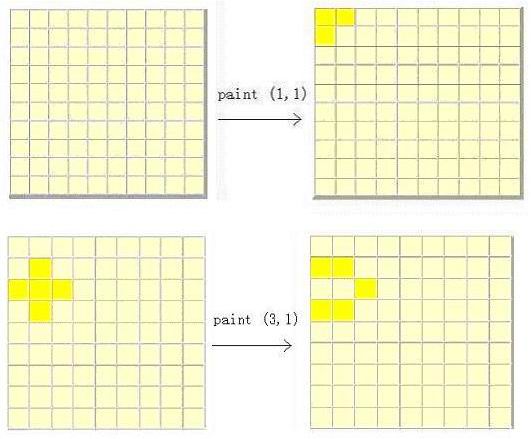
Input
first line contains a single integer t (1 <= t <= 20) that
indicates the number of test cases. Then follow the t cases. Each test
case begins with a line contains an integer n (1 <= n <= 15),
representing the size of wall. The next n lines represent the original
wall. Each line contains n characters. The j-th character of the i-th
line figures out the color of brick at position (i, j). We use a 'w' to
express a white brick while a 'y' to express a yellow brick.
Output
each case, output a line contains the minimum number of bricks Bob
should paint. If Bob can't paint all the bricks yellow, print 'inf'.
Sample Input
2
3
yyy
yyy
yyy
5
wwwww
wwwww
wwwww
wwwww
wwwww
Sample Output
0
15
Source
/**
题意:根据给出的图,问有多少种方法使得变为全‘y’
做法:高斯消元 建一个n*n的矩阵
**/
#include <iostream>
#include <string.h>
#include <stdio.h>
#include <algorithm>
#include <cmath>
#define maxn 250
using namespace std;
int mmap[maxn][maxn];
int x[maxn];
int equ,val;
char ch[][];
int free_x[maxn];
int gcd(int a,int b)
{
if(b == ) return a;
return gcd(b,a%b);
}
int Lcm(int a,int b)
{
return a/gcd(a,b)*b;
}
int Guess()
{
int lcm;
int ta;
int tb;
int max_r;
int k;
int col;
col = ;
for(k = ; k<equ&&col < val; k++,col++)
{
max_r = k;
for(int i=k+; i<equ; i++)
{
if(abs(mmap[i][col]) > abs(mmap[max_r][col]))
{
max_r = i;
}
}
if(mmap[max_r][col] == )
{
k--;
continue;
}
if(max_r != k)
{
for(int i=col; i<val+; i++)
{
swap(mmap[max_r][i],mmap[k][i]);
}
}
for(int i=k+; i<equ; i++)
{
if(mmap[i][col] != )
{
for(int j=col; j<val+; j++)
{
mmap[i][j] ^= mmap[k][j];
}
}
}
}
for(int i=k; i<equ; i++)
{
if(mmap[i][col] != ) return -;
}
for(int i=val-; i>=; i--)
{
x[i] = mmap[i][val];
for(int j=i+; j<val; j++)
{
x[i] ^= (mmap[i][j] & x[j]);
}
}
return ;
}
void init(int n)
{
memset(x,,sizeof(x));
memset(mmap,,sizeof(mmap));
for(int i=; i<n; i++)
{
for(int j=; j<n; j++)
{
int tt = i * n +j;
mmap[tt][tt] = ;
if(i > ) mmap[(i-)*n+j][tt] = ;
if(i < n-) mmap[(i+)*n+j][tt] = ;
if(j > ) mmap[i*n + j - ][tt] = ;
if(j < n-) mmap[i*n + j + ][tt] = ;
}
}
}
void solve(int tt)
{
int res = Guess();
if(res == -) printf("inf\n");
else if(res == )
{
int ans = ;
for(int i=; i<=tt; i++)
{
ans += x[i];
}
printf("%d\n",ans);
return;
}
else
{
int ans = 0x3f3f3f3f;
int tot = (<<res);
for(int i=; i<tot; i++)
{
int cnt = ;
for(int j=; j<res; j++)
{
if(i &(<<j))
{
x[free_x[j]] = ;
cnt++;
}
else x[free_x[j]] = ;
}
for(int j=val-tt-; j>=; j--)
{
int k;
for( k=j; k<val; k++)
if(mmap[j][k]) break;
x[k] = mmap[j][val];
for(int l=k+; l < val; l++)
if(mmap[j][l]) x[k] ^= x[l];
cnt += x[k]; }
ans = min(ans,cnt);
}
printf("%d\n",ans);
}
return;
}
int main()
{
//#ifndef ONLINE_JUDGE
// freopen("in.txt","r",stdin);
//#endif // ONLINE_JUDGE
int T;
scanf("%d",&T);
while(T--)
{
int n;
scanf("%d",&n);
char c[];
init(n);
int tt = n*n;
equ = val = tt;
for(int i=; i<n; i++)
{
scanf("%s",c);
for(int j=; j<n; j++)
{
if(c[j] == 'y') mmap[i*n+j][tt] = ;
else mmap[i*n+j][tt] = ; }
}
solve(tt);
}
return ;
}
POJ-1681的更多相关文章
- POJ 1222 POJ 1830 POJ 1681 POJ 1753 POJ 3185 高斯消元求解一类开关问题
http://poj.org/problem?id=1222 http://poj.org/problem?id=1830 http://poj.org/problem?id=1681 http:// ...
- POJ 1681 (开关问题+高斯消元法)
题目链接: http://poj.org/problem?id=1681 题目大意:一堆格子,或白或黄.每次可以把一个改变一个格子颜色,其上下左右四个格子颜色也改变.问最后使格子全部变黄,最少需要改变 ...
- OpenJudge 2813 画家问题 / Poj 1681 Painter's Problem
1.链接地址: http://bailian.openjudge.cn/practice/2813 http://poj.org/problem?id=1681 2.题目: 总时间限制: 1000ms ...
- POJ 1681 Painter's Problem(高斯消元+枚举自由变元)
http://poj.org/problem?id=1681 题意:有一块只有黄白颜色的n*n的板子,每次刷一块格子时,上下左右都会改变颜色,求最少刷几次可以使得全部变成黄色. 思路: 这道题目也就是 ...
- POJ 1681 Painter's Problem 【高斯消元 二进制枚举】
任意门:http://poj.org/problem?id=1681 Painter's Problem Time Limit: 1000MS Memory Limit: 10000K Total ...
- poj 1681 Painter's Problem(高斯消元)
id=1681">http://poj.org/problem? id=1681 求最少经过的步数使得输入的矩阵全变为y. 思路:高斯消元求出自由变元.然后枚举自由变元,求出最优值. ...
- poj 1681 Painter's Problem
Painter's Problem 题意:给一个n*n(1 <= n <= 15)具有初始颜色(颜色只有yellow&white两种,即01矩阵)的square染色,每次对一个方格 ...
- poj 1681(Gauss 消元)
Painter's Problem Time Limit: 1000MS Memory Limit: 10000K Total Submissions: 5875 Accepted: 2825 ...
- POJ 1681 Painter's Problem (高斯消元)
题目链接 题意:有一面墙每个格子有黄白两种颜色,刷墙每次刷一格会将上下左右中五个格子变色,求最少的刷方法使得所有的格子都变成yellow. 题解:通过打表我们可以得知4*4的一共有4个自由变元,那么我 ...
- POJ 1681 Painter's Problem (高斯消元 枚举自由变元求最小的步数)
题目链接 题意: 一个n*n 的木板 ,每个格子 都 可以 染成 白色和黄色,( 一旦我们对也个格子染色 ,他的上下左右 都将改变颜色): 给定一个初始状态 , 求将 所有的 格子 染成黄色 最少需要 ...
随机推荐
- bzoj 2588 Count on a tree 解题报告
Count on a tree 题目描述 给定一棵\(N\)个节点的树,每个点有一个权值,对于\(M\)个询问\((u,v,k)\),你需要回答\(u\) \(xor\) \(lastans\)和\( ...
- Mac添加锁屏快捷键
Mac要想添加锁屏快捷键,必须使用Automator. 1. 打开Automator,创建一个新的服务. 2. 在左侧栏中找到 启动屏幕保护 ,将其拖曳到右侧窗口内,并且修改 服务收到改为" ...
- java 实现多个接口 方法重名的解决办法——内部类
package com.kk.innerClass; /** * 通过内部类实现接口 * 解决多个接口中方法重名问题 * */interface Machine { void run();} clas ...
- 直通BAT面试算法精讲课1
1.有一棵二叉树,请设计一个算法,按照层次打印这棵二叉树. 给定二叉树的根结点root,请返回打印结果,结果按照每一层一个数组进行储存,所有数组的顺序按照层数从上往下,且每一层的数组内元素按照从左往右 ...
- angularJS 条件查询 品优购条件查询品牌(条件查询和列表展示公用方法解决思路 及 post请求混合参数提交方式)
Brand.html <!DOCTYPE html> <html> <head> <meta charset="utf-8"> &l ...
- CursorAdapter中getView newView bindView异同
Adapter的作用是界面与数据之间的桥梁,通过设置适配器至ListView控件后(如调用ListView的 setAdapter(ListAdapter adapter) ...
- 二叉树系列 - 二叉树里的最长路径 例 [LeetCode] Binary Tree Maximum Path Sum
题目: Binary Tree Maximum Path Sum Given a binary tree, find the maximum path sum. The path may start ...
- windows版本redis下载安装
官方网站:http://redis.io/ 官方下载:http://redis.io/download 可以根据需要下载不同版本 在官方下载网页中有一个window版本的说明,说redis官方没有wi ...
- javascript拖拽原理与简单实现方法[demo]
美国人有一句常用的俗语—“Re-inventing the Wheel”,从字面上来解释就是“重新发明轮子”.可是轮子早已问世,再要去发明岂非劳而无功? 产品经理发下需求,实施者再到网上搜索代码,也许 ...
- OPENId是什么, OAUTH 是什么
what is openId open id is said to be a protocol which uses url as username, so if a website supports ...
