The Problem of Overfitting

Cost Function

Regularized Linear Regression

Note: [8:43 - It is said that X is non-invertible if m ≤ n. The correct statement should be that X is non-invertible if m < n, and may be non-invertible if m = n.

We can apply regularization to both linear regression and logistic regression. We will approach linear regression first.

Regularized Logistic Regression

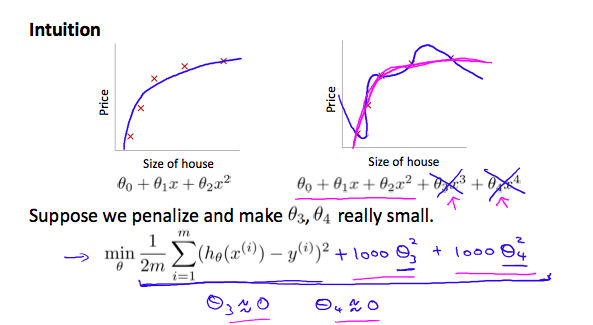
We can regularize logistic regression in a similar way that we regularize linear regression. As a result, we can avoid overfitting. The following image shows how the regularized function, displayed by the pink line, is less likely to overfit than the non-regularized function represented by the blue line:

Solving the Problem of Overfitting的更多相关文章

  1. machine learning(13) -- solving the problem of overfitting:regularization

    solving the problem of overfitting:regularization 发生的在linear regression上面的overfitting问题 发生在logistic ...

  2. 机器学习(四)正则化与过拟合问题 Regularization / The Problem of Overfitting

    文章内容均来自斯坦福大学的Andrew Ng教授讲解的Machine Learning课程,本文是针对该课程的个人学习笔记,如有疏漏,请以原课程所讲述内容为准.感谢博主Rachel Zhang 的个人 ...

  3. The first step in solving any problem is recognizing there is one.

    The first step in solving any problem is recognizing there is one.解决问题的第一步是要承认确实存在问题.

  4. 吴恩达机器学习笔记19-过拟合的问题（The Problem of Overfitting）

    到现在为止,我们已经学习了几种不同的学习算法,包括线性回归和逻辑回归,它们能够有效地解决许多问题,但是当将它们应用到某些特定的机器学习应用时,会遇到过拟合(over-fitting)的问题,可能会导致 ...

  5. 1763 An Essay towards solving a Problem in the Doctrine of Chances

    https://en.wikipedia.org/wiki/An_Essay_towards_solving_a_Problem_in_the_Doctrine_of_Chances

  6. Ng第七课：正则化与过拟合问题 Regularization/The Problem of Overfitting

    7.1  过拟合的问题 7.2  代价函数 7.3  正则化线性回归 7.4  正则化的逻辑回归模型 7.1  过拟合的问题 如果我们有非常多的特征,我们通过学习得到的假设预测可能能够非常好地适应训练 ...

  7. Machine Learning - 第3周（Logistic Regression、Regularization）

    Logistic regression is a method for classifying data into discrete outcomes. For example, we might u ...

  8. 《Machine Learning》系列学习笔记之第三周

    第三周 第一部分 Classification and Representation Classification 为了尝试分类,一种方法是使用线性回归,并将大于0.5的所有预测映射为1,所有小于0. ...

  9. Andrew Ng机器学习课程笔记--week3（逻辑回归&正则化参数）

    Logistic Regression 一.内容概要 Classification and Representation Classification Hypothesis Representatio ...

随机推荐

  1. C# WPF开源控件库MaterialDesign介绍

    介绍 1.由于前端时间萌发开发一个基础架构得WPF框架得想法, 然后考虑到一些界面层元素统一, 然后就无意间在GitHub上发现一个开源WPF UI, 于是下载下来了感觉不错. 官网地址:http:/ ...

  2. Java反射之getInterfaces()方法

    今天学习Spring3框架,在理解模拟实现Spring Ioc容器的时候遇到了getInterfaces()方法.getInterfaces()方法和Java的反射机制有关.它能够获得这个对象所实现的 ...

  3. 堆－heap

    #include <vector> #include <cstdio> using namespace std; class Heap { private : vector&l ...

  4. zenDiscovery和master选举

    上一篇通过 ElectMasterService源码,分析了master选举的原理的大部分内容:master候选节点ID排序保证选举一致性及通过设置最小可见候选节点数目避免brain split.节点 ...

  5. Bean复制的几种框架性能比较（Apache BeanUtils、PropertyUtils,Spring BeanUtils,Cglib BeanCopier）

    转自:http://www.cnblogs.com/kaka/archive/2013/03/06/2945514.html 比较的是四种复制的方式,分别为Apache的BeanUtils和Prope ...

  6. ReactJs 入门DEMO（转自别人）

    附件是分享的一些他人的ReactJs入门DEMO,以前版本使用的是JSXTransformer.js,新版的用browser.min.js替代了. DEMO 下载地址:http://files.cnb ...

  7. 免费的EmBitz可替代Keil MDK开发STM32、NXP项目

    一.背景 由于使用之前开发STM32是基于Keil MDK编译环境开发的,由于该软件是收费的,想用个免费开源的软件来替代Keil,EmBitz编译器是免费的,可以完全替代开发.下载程序支持J-Link ...

  8. hibernate中的事务管理是怎么概念?

    1.JDBC事务 JDBC 事务是用 Connection 对象控制的.JDBC Connection 接口( java.sql.Connection )提供了两种事务模式:自动提交和手工提交. ja ...

  9. 洛谷——P1443 马的遍历

    https://www.luogu.org/problem/show?pid=1443#sub 题目描述 有一个n*m的棋盘(1<n,m<=400),在某个点上有一个马,要求你计算出马到达 ...

  10. shell实例浅谈之三产生随机数七种方法

    一.问题 Shell下有时须要使用随机数,在此总结产生随机数的方法.计算机产生的的仅仅是"伪随机数".不会产生绝对的随机数(是一种理想随机数).伪随机数在大量重现时也并不一定保持唯 ...