SMU Summer 2024 Contest Round 4

SMU Summer 2024 Contest Round 4

Made Up

题意

给你三个序列 \(A，B，C\) ，问你满足 \(A_i = B_{C_j}\) 的 \((i,j)\) 对有多少。

思路

由于 \(1\le A_i,B_i,C_i\le N\) ，所以可以统计 \(Cnt[A_i]\) 和 \(Cnt[B_{C_i}]\) 的个数，两者相乘累加即可。

代码

#include<bits/stdc++.h>

using namespace std;

using i64 = long long;

int main() {
    ios::sync_with_stdio(false);
    cin.tie(nullptr);

    int n;
    cin >> n;

    vector<int> a(n), b(n), c(n);

    vector<i64> cnta(n + 1);
    for (auto &i : a) {
        cin >> i;
        cnta[i] ++;
    }

    for (auto &i : b)
        cin >> i;

    vector<i64> cntb(n + 1);
    for (auto &i : c) {
        cin >> i;
        cntb[b[--i]] ++;
    }

    i64 ans = 0;
    for (int i = 1; i <= n; i ++) {
        ans += cnta[i] * cntb[i];
    }

    cout << ans << '\n';

    return 0;
}

H and V

题意

给你 \(H\times W\) 的只包含. 和#的矩阵， 你可以选择任意行任意列改成.，问你修改后使得矩阵中#的个数等于 k 的方案有多少种。

思路

因为 \(1\le H,W\le 6\)，所以直接对边和列枚举改哪些行和列即可。

代码

#include<bits/stdc++.h>

using namespace std;

using i64 = long long;

int main() {
    ios::sync_with_stdio(false);
    cin.tie(nullptr);

    int h, w, k;
    cin >> h >> w >> k;

    vector<string> s(h);
    for (auto &i : s)
        cin >> i;

    auto calc = [](vector<string> ss) {
        int res = 0;
        for (auto i : ss)
            for (auto c : i)
                res += (c == '#');
        return res;
    };

    int ans = 0;
    for (int i = 0; i < (1 << h); i ++) {
        for (int j = 0; j < (1 << w); j ++) {
            auto S = s;
            for (int k = 0; k < h; k ++)
                if ((i >> k) & 1)
                    S[k] = string(w, '.');
            for (int k = 0; k < w; k ++)
                if ((j >> k) & 1) {
                    for (int p = 0; p < h; p ++)
                        S[p][k] = '.';
                }

            if (calc(S) == k)
                ans ++;
        }
    }

    cout << ans << '\n';

    return 0;
}

Moving Piece

题意

给你 n 个点，然后每个点 可以到达其他第 \(P_i\) 个点，到达下个点后可以获得对应的 \(C_i\) 分数，你可以选择某个点为起始点走 \(K\) 步，起始点的分数不计，问你最大能获得多少分。

思路

因为每个点只有一个方向，所以将它们抽象成图以后就是多个环，而你需要在这些环上找一个环走 \(K\) 步。

当走的步数大于环上的点数时，直接计算一下会经过多少次这个环，乘以环的总分数即可。

代码

#include<bits/stdc++.h>

using namespace std;

using i64 = long long;

int main() {
    ios::sync_with_stdio(false);
    cin.tie(nullptr);

    int n, k;
    cin >> n >> k;

    vector<int> p(n + 1);
    for (int i = 1; i <= n; i ++)
        cin >> p[i];

    vector<int> C(n + 1);
    for (int i = 1; i <= n; i ++)
        cin >> C[i];

    i64 ans = INT_MIN;
    for (int i = 1; i <= n; i ++) {
        i64 to = i, sum = 0;
        vector<i64> path;
        while (true) {
            to = p[to];
            sum += C[to];
            path.push_back(sum);
            if (to == i) break;
        }

        const int dis = path.size();
        for (int j = 0; j < dis && j < k; j ++) {
            int CircleNum = (k - 1 - j) / dis;
            ans = max(ans, path[j]);
            if(CircleNum > 0)
                ans = max(ans, path[j] + CircleNum * sum);
        }
    }

    cout << ans << '\n';

    return 0;
}

Sum of Divisors

题意

设 \(f(X)\) 为 \(X\) 的所有因子数，求 \(\sum_{K=1}^NK\times f(K)\)。

思路

考虑一个数产生的贡献。

对于一个数，它只会在它的倍数中产生贡献，例如，2 只会在 \(2,4,6,8,10\dots\) 中产生贡献，而在上界为 \(N\) 的情况下只会有 \(\frac{N}{i}\) 个 \(i\) 的倍数，而对于 2 而言，它在 2 中产生的贡献为 2，在4 中的产生的贡献为 4，在 6 中产生的贡献为 6，\(\dots\)，其他数同理，观察可得一个数产生的贡献为 \(i + 2i+3i+4i+\dots\)，没错，这就是一个首项为 \(i\) ，公差为 \(i\)，项数为 \(\frac{N}{i}\)，末项为 \(\lfloor\frac{N}{i}\rfloor\times i\) 的等差数列，所以从 1 到 n 累加求和即可。

\[\sum_{i=1}^n\sum_{j|i}^nj=\sum_{i=1}^n\sum_{j=1}^{\frac{n}{i}}j\times i=\sum_{i=1}^n\lfloor\frac{N}{i}\rfloor\times \frac{i+\lfloor\frac{N}{i}\rfloor\times i}{2}
\]

代码

#include<bits/stdc++.h>

using namespace std;

using i64 = long long;

int main() {
    ios::sync_with_stdio(false);
    cin.tie(nullptr);

    int n;
    cin >> n;

    i64 ans = 0;
    for(int i = 1;i <= n;i ++){
        i64 d = i, a1 = i, len = n / i, an = d * len;
        ans += len * (a1 + an) / 2;
    }

    cout << ans << '\n';

    return 0;
}

Red and Green Apples

题意

给你三个序列 \(A，B，C\)，\(C\) 序列中的任何数可以替换 \(A，B\) 中的任何数，你要选出 A 中 X 个，B 中 Y 个，求它们的和最大。

思路

将 A，B 排序后取前 X 个和前 Y 个放入 C 中，再将 C 排序，取出前 X+Y 个即可。

代码

#include<bits/stdc++.h>

using namespace std;

using i64 = long long;

int main() {
    ios::sync_with_stdio(false);
    cin.tie(nullptr);

    int x, y, a, b, c;
    cin >> x >> y >> a >> b >> c;

    vector<int> A(a), B(b), C(c);
    for (auto &i : A)
        cin >> i;

    for (auto &i : B)
        cin >> i;

    for (auto &i : C)
        cin >> i;

    sort(A.begin(), A.end(), greater<>());
    sort(B.begin(), B.end(), greater<>());

    for(int i = 0;i < x;i ++)
        C.push_back(A[i]);

    for(int i = 0;i < y;i ++)
        C.push_back(B[i]);

    sort(C.begin(),C.end(),greater<>());

    i64 ans = 0;
    for(int i = 0;i < x + y;i ++)
        ans += C[i];

    cout << ans << '\n';

    return 0;
}

Rem of Sum is Num

题意

给你序列 A，求 A 中连续子序列之和模 K 后等于该子序列中元素数量的连续子序列数量。

思路

转化题意，即求:

\[\sum_{l=1}^{n}\sum_{r=l}^n[\sum_{k=l}^rA_k\bmod k=r-(l-1)]
\]

复杂度\(O(n^3)\)，显然超时。

考虑优化，前缀和优化即：

\[(sum_r-sum_{l-1})\bmod k = r-(l-1)\\
\]

\[((sum_r-sum_{l-1})-(r-(l-1)))\bmod k = 0\\
\]

\[(sum_r-r)\bmod k=(sum_{l-1}-(l-1))\bmod k
\]

即当满足以上条件相等时，说明 \((l-1,r]\) 是一段满足要求的连续子序列。

因为这里 r 从 1 开始时，l - 1 会等于 0 ，所以需要我们将 0 的方案数初始化为 1 。

至此，这道题就变成了求区间长度最大为 k 的 满足以上条件的方案数，在模 k 后其区间元素数量最多为 k ，否则就不满足条件，超过区间长度需减掉。

代码

#include<bits/stdc++.h>

using namespace std;

using i64 = long long;

int main() {
    ios::sync_with_stdio(false);
    cin.tie(nullptr);

    i64 n, k;
    cin >> n >> k;

    vector<i64> a(n + 1), pre(n + 1);
    for (int i = 1; i <= n; i ++) {
        cin >> a[i];
        pre[i] = pre[i - 1] + a[i];
    }

    for (int i = 1; i <= n; i ++) {
        pre[i] = (pre[i] - i) % k;
    }

    i64 ans = 0;
    int len = min(k-1, n);
    map<i64, i64> mp;

	mp[0] = 1;
    for (int i = 1; i <= n; i ++) {
        if (i > len) mp[pre[i - k]]--;
        ans += mp[pre[i]];
        mp[pre[i]] ++;
    }

    cout << ans << '\n';

    return 0;
}

Keep Connect

题意

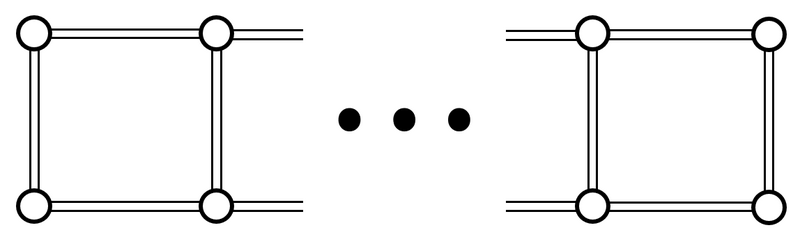
给定 $ n, p $，存在如图的 $ 2 \times n $ 的网格图，显然初始共有 $ 2n $ 个顶点和 $ 3n - 2 $ 条边，分别求删除 $ i \in [1, n - 1] $ 条边后仍使图连通的删边方案数，对 $ p $ 取模。

思路

将上下的两个点看成一列，考虑 dp。

设 \(dp_{i,j,0/1}\) 为前 i 列中删掉 j 条边是否连通的方案数。

首先假设第 i 列连通：

• 那么会有四种情况使得第 i+1 列连通：

  • 前三种：
    
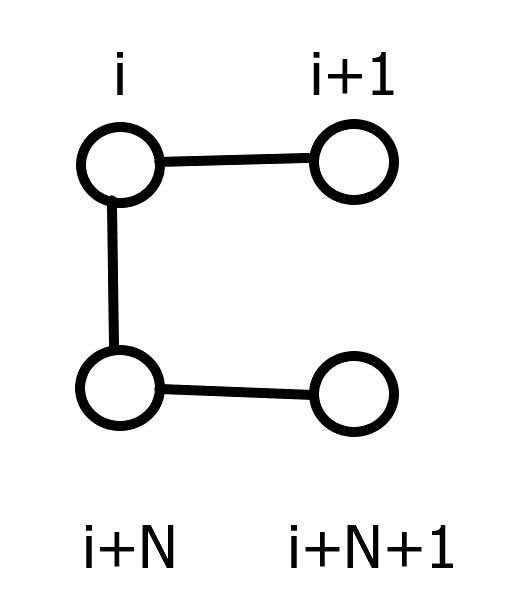
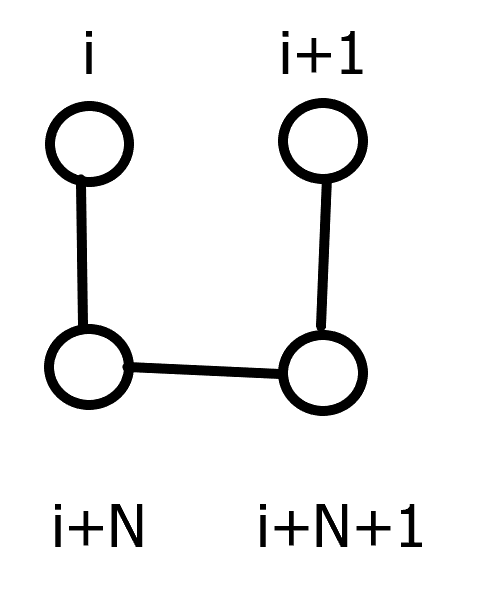
    得出转移方程为 \(dp_{i+1,j+1,1} += dp_{i,j,1}\times 3\)

  • 第四种：

    得出转移方程为 \(dp_{i+1,j,1}+=dp_{i,j,1}\)

• 会有两种情况使得第 i+1 列无法连通：

  

  

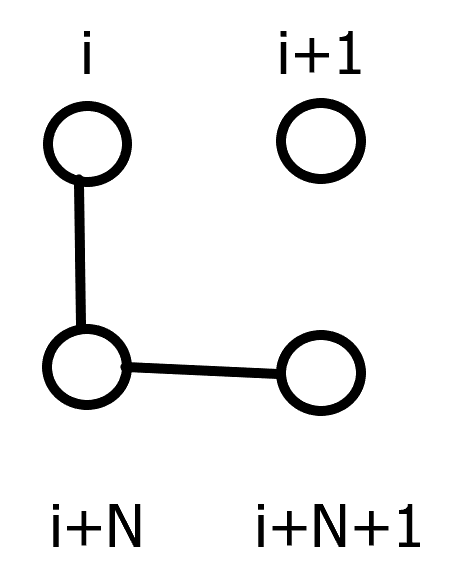
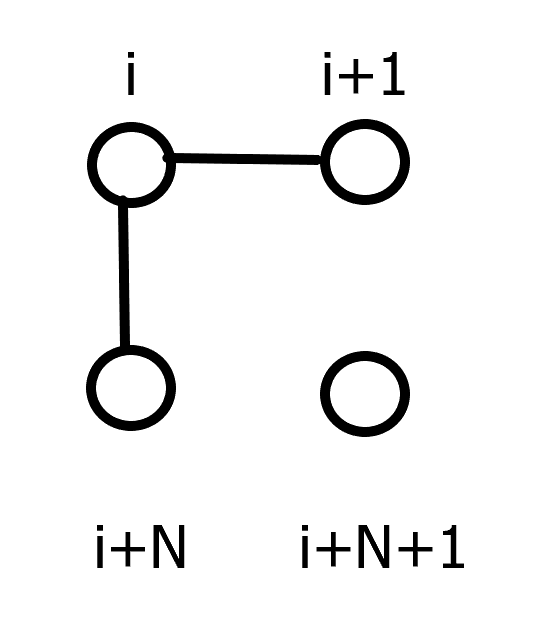
  得出转移方程为 \(dp_{i+1,j+2,0}+=dp_{i,j,1}\times 2\)

假设第 i 列不连通：

• 使得第 i+1 列连通：

  

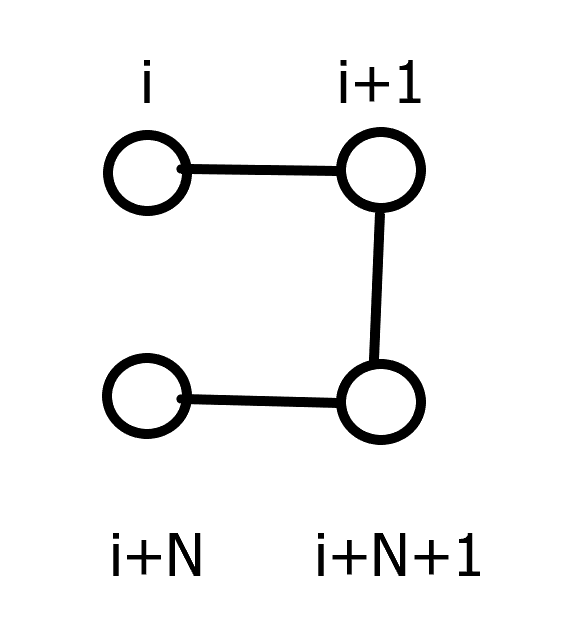
  得出转移方程为 \(dp_{i+1,j,1}+=dp_{i,j,0}\)

• 使得第 i+1 列不连通：

  

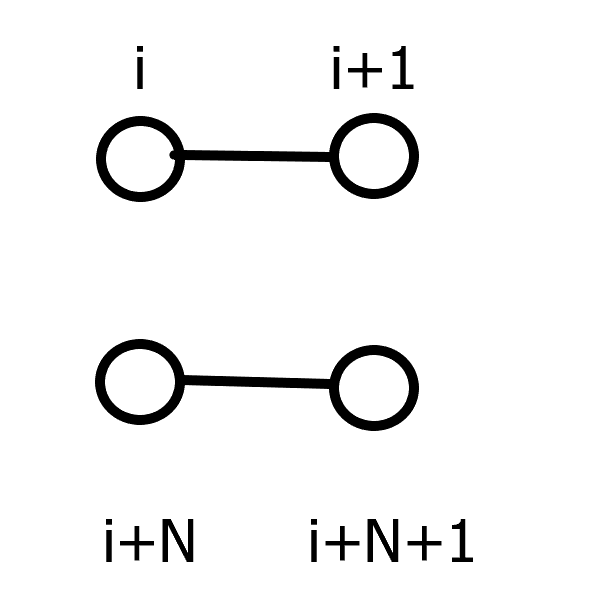
  得出转移方程为 \(dp_{i+1,j+1,0}+=dp_{i,j,0}\)

另外还有些减去三边的情况，但是那种情况产生后后面都不可能再连通，对答案无贡献。

代码

#include<bits/stdc++.h>

using namespace std;

using i64 = long long;

const int N = 3e3 + 10;
i64 dp[N][N][2];

int main() {
    ios::sync_with_stdio(false);
    cin.tie(nullptr);

    i64 n, p;
    cin >> n >> p;

    dp[1][0][1] = dp[1][1][0] = 1;
    for (int i = 1; i <= n; i ++) {
        for (int j = 0; j < n; j ++) {
            dp[i + 1][j + 1][1] = (dp[i + 1][j + 1][1] + dp[i][j][1] * 3) % p;
            dp[i + 1][j][1] = (dp[i + 1][j][1] + dp[i][j][1]) % p;
            dp[i + 1][j + 2][0] = (dp[i + 1][j + 2][0] + dp[i][j][1] * 2) % p;
            dp[i + 1][j][1] = (dp[i + 1][j][1] + dp[i][j][0]) % p;
            dp[i + 1][j + 1][0] = (dp[i + 1][j + 1][0] + dp[i][j][0]) % p;
        }
    }

    for (int i = 1; i < n; i ++)
        cout << dp[n][i][1] << " \n"[i == n - 1];

    return 0;
}