uva1424

Traveling salesmen of nhn. (the prestigious Korean internet company) report their current location to the company on a regular basis. They also have to report their new location to the company if they are moving to another location. The company keep each salesman's working path on a map of his working area and uses this path information for the planning of the next work of the salesman. The map of a salesman's working area is represented as a connected and undirected graph, where vertices represent the possible locations of the salesman an edges correspond to the possible movements between locations. Therefore the salesman's working path can be denoted by a sequence of vertices in the graph. Since each salesman reports his position regularly an he can stay at some place for a very long time, the same vertices of the graph can appear consecutively in his working path. Let a salesman's working path be correct if two consecutive vertices correspond either the same vertex or two adjacent vertices in the graph.

For example on the following graph representing the working area of a salesman,

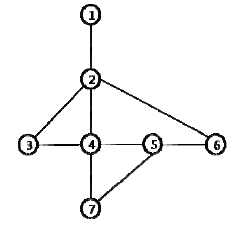<tex2html_verbatim_mark>

a reported working path [1 2 2 6 5 5 5 7 4] is a correct path. But a reported working path [1 2 2 7 5 5 5 7 4] is not a correct path since there is no edge in the graph between vertices 2 a 7. If we assume that the salesman reports his location every time when he has to report his location (but possibly incorrectly), then the correct path could be [1 2 2 4 5 5 5 7 4], [1 2 4 7 5 5 5 7 4], or [1 2 2 6 5 5 5 7 4].

The length of a working path is the number of vertices in the path. We define the distance between two pathsA = a₁a₂...a_n <tex2html_verbatim_mark>and B = b₁b₂...b_n <tex2html_verbatim_mark>of the same length n <tex2html_verbatim_mark>as

dist(A, B) = d (a_i, b_i)

<tex2html_verbatim_mark>

where

d (a, b) = 

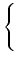
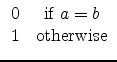
<tex2html_verbatim_mark>

Given a graph representing the working area of a salesman and a working path (possible not a correct path),A <tex2html_verbatim_mark>, of a salesman, write a program to compute a correct working path, B <tex2html_verbatim_mark>, of the same length where the distance dist(A, B) <tex2html_verbatim_mark>is minimized.

Input

The program is to read the input from standard input. The input consists of T <tex2html_verbatim_mark>test cases. The number of test cases (T) <tex2html_verbatim_mark>is given in the first line of the input. The first line of each test case contains two integers n₁<tex2html_verbatim_mark>, n₂ <tex2html_verbatim_mark>(3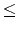n₁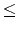100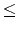, 2n₂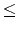4, 950) <tex2html_verbatim_mark>where n₁ <tex2html_verbatim_mark>is the number of vertices of the graph representing the working map of a salesman and n₂ <tex2html_verbatim_mark>is the number of edges in the graph. The input graph is a connected graph. Each vertex of the graph is numbered from 1 to n₁ <tex2html_verbatim_mark>. In the following n₂ <tex2html_verbatim_mark>lines, each line contains a pair of vertices which represent an edge of the graph. The last line of each test case contains information on a working path of the salesman. The first integer n <tex2html_verbatim_mark>(2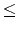n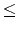200) <tex2html_verbatim_mark>in the line is the length of the path and the following n integers represent the sequence of vertices in the working path.

Output

Your program is to write to standard output. Print one line for each test case. The line should contain the minimum distance of the input path to a correct path of the same length.

Sample Input

2
7 9
1 2
2 3
2 4
2 6
3 4
4 5
5 6
7 4
7 5
9 1 2 2 7 5 5 5 7 4
7 9
1 2
2 3
2 4
2 6
3 4
4 5
5 6
7 4
7 5
9 1 2 2 6 5 5 5 7 4

Sample Output

1
0
这题说的是给了一个图，如上，一个人从图中的任意位置出发，总共返回了n次自己的位置，这些位置可能是不合法的也就是所两点之间不相邻但是他们却在返回的点中相邻，返回的点中允许有相同的点相邻，（表明他也一直在这个点上）， 
输入 给n个点，表示返回的n个点。
输出 找出一条路径同样拥有n个点（合法的），使得这两序列的最长公共子序列最长，输出差异的几个数
   先用floyd 求出任意点之间的距离，然后dp[i] 表示到i为止合法的最小差异， 初始化的是dp[i]=i;
然后 当他们的距离小于等于他们在序列中出现的顺序的时候 dp[j]=min(dp[j],dp[i]+j-i-1);
输出dp[n-1]

#include <iostream>
#include <cstdio>
#include <string.h>
#include <algorithm>
#include <cmath>
using namespace std;
const int maxn=;
const int INF =;
int dist[maxn][maxn];
int sa[maxn*];
int dp[maxn*];
void inti(int n1){
      for(int k=; k<=n1; ++k)
      for(int i=; i<=n1; ++i)
         for(int j=; j<=n1; ++j)
              if(dist[i][k]<INF&&dist[k][j]<INF)
            dist[i][j]=dist[i][j]<(dist[i][k]+dist[k][j])?dist[i][j]:(dist[i][k]+dist[k][j]);

}
int main()
{
     int cas;
     scanf("%d",&cas);
     while(cas--){
         int n1,n2;
         scanf("%d%d",&n1,&n2);
         for(int i=; i<=n1; i++)
           for(int j=; j<=n1; ++j )
             dist[i][j]=i==j?:INF;
         for(int i=; i<n2; ++i){
             int a,b;
             scanf("%d%d",&a,&b);
            dist[a][b]=dist[b][a]=;
         }
         inti(n1);
        int n;
        scanf("%d",&n);
        for(int i=; i<n; ++i)
            scanf("%d",&sa[i]);
        for(int i=; i<n; ++i)
            dp[i]=i;
        for(int i=; i<n; ++i)
             for( int j=i+; j<n; ++j)
               if(dist[ sa[i] ][ sa[j] ]<=j-i)
                dp[j]=min(dp[j], dp[i]+j-i-);
       printf("%d\n",dp[n-]);
     }
    return ;
}