2019-2020 ACM-ICPC Brazil Subregional Programming Contest (11/13)
\(2019-2020\ ACM-ICPC\ Brazil\ Subregional\ Programming\ Contest\)
\(A.Artwork\)
并查集,把检测区域能在一起的检测器放在一个并查集里,然后判断是否有一个集合能够封住左边和上边的其中一个还有右边和下边的其中一个即可
//#pragma comment(linker, "/STACK:1024000000,1024000000")
#include<bits/stdc++.h>
using namespace std;
function<void(void)> ____ = [](){ios_base::sync_with_stdio(false); cin.tie(0); cout.tie(0);};
const int MAXN = 1111;
const int INF = 0x3f3f3f3f;
int n,m,k,root[MAXN],tot;
pair<pair<int,int>,int> sensor[MAXN];
vector<int> SET[MAXN];
map<int,int> mp;
int findx(int x){
if(x!=root[x]) root[x] = findx(root[x]);
return root[x];
}
int dist(const pair<int,int> &A, const pair<int,int> &B){
return (A.first-B.first)*(A.first-B.first)+(A.second-B.second)*(A.second-B.second);
}
int main(){
scanf("%d %d %d",&n,&m,&k);
for(int i = 1; i <= k; i++) scanf("%d %d %d",&sensor[i].first.first,&sensor[i].first.second,&sensor[i].second);
for(int i = 1; i <= k; i++) root[i] = i;
for(int i = 1; i <= k; i++) for(int j = i+1; j <= k; j++){
int fa = findx(i);
int fb = findx(j);
if(fa==fb) continue;
if((sensor[i].second+sensor[j].second)*(sensor[i].second+sensor[j].second)>=dist(sensor[i].first,sensor[j].first)){
root[fa] = fb;
}
}
for(int i = 1; i <= k; i++){
if(!mp.count(findx(i))) mp.insert(make_pair(findx(i),++tot));
SET[mp.at(findx(i))].emplace_back(i);
}
bool ok = true;
for(int i = 1; i <= tot; i++){
int u = -INF, d = INF, l = INF, r = -INF;
for(auto p : SET[i]){
u = max(u,sensor[p].first.second+sensor[p].second);
d = min(d,sensor[p].first.second-sensor[p].second);
l = min(l,sensor[p].first.first-sensor[p].second);
r = max(r,sensor[p].first.first+sensor[p].second);
}
if((u>=m||l<=0)&&(d<=0||r>=n)){
ok = false;
break;
}
}
puts(ok?"S":"N");
return 0;
}
\(B.Buffoon\)
签到
#include<bits/stdc++.h>
using namespace std;
const int N = 1e5+10;
int a[N],n,maxi;
int main(){
cin>>n;
for(int i=1;i<=n;++i) cin >> a[i], maxi = max(maxi,a[i]);
if(maxi==a[1]) cout << "S";
else cout << "N";
return 0;
}
\(C.Crossings\ With\ Danger\)
\(D.Denouncing Mafia\)
树形DP+优先队列 对于每个节点记录它的最深节点的位置,把链长为关键字丢到优先队列中,每次找最长的没有被遍历过的链
//#pragma comment(linker, "/STACK:1024000000,1024000000")
#include<bits/stdc++.h>
using namespace std;
function<void(void)> ____ = [](){ios_base::sync_with_stdio(false); cin.tie(0); cout.tie(0);};
const int MAXN = 1e5+7;
int n,m,par[MAXN],depth[MAXN],vis[MAXN];
vector<int> G[MAXN];
priority_queue<pair<int,pair<int,int>>,vector<pair<int,pair<int,int>>>,less<pair<int,pair<int,int>>>> que;
int dfs(int u){
depth[u] = depth[par[u]]+1;
int maxdep = u;
for(int v : G[u]){
int p = dfs(v);
maxdep = depth[maxdep]<depth[p]?p:maxdep;
}
que.push(make_pair(depth[maxdep]-depth[u]+1,make_pair(maxdep,u)));
return maxdep;
}
int main(){
scanf("%d %d",&n,&m);
for(int i = 2; i <= n; i++){
scanf("%d",&par[i]);
G[par[i]].emplace_back(i);
}
dfs(1);
int ans = 0;
while(!que.empty()&&m){
auto p = que.top();
que.pop();
if(vis[p.second.second]) continue;
m--;
ans += p.first;
while(p.second.first!=par[p.second.second]){
vis[p.second.first] = 1;
p.second.first = par[p.second.first];
}
}
printf("%d\n",ans);
return 0;
}
\(E.Exhibition\ of\ Clownfish\)
\(F.Forests\ in\ Danger\)
二分河流宽度,然后得到的矩形先和大矩形求交,再把所有矩形求面积并即可
//#pragma comment(linker, "/STACK:1024000000,1024000000")
#include<bits/stdc++.h>
using namespace std;
function<void(void)> ____ = [](){ios_base::sync_with_stdio(false); cin.tie(0); cout.tie(0);};
using LL = int_fast64_t;
const int MAXN = 1e5+7;
struct Rectangle{
int x1,y1,x2,y2;
Rectangle (int _x1 = 0, int _y1 = 0, int _x2 = 0, int _y2 = 0){
x1 = _x1; y1 = _y1;
x2 = _x2; y2 = _y2;
}
Rectangle intersect(const Rectangle &rhs){
int nx1 = max(x1,rhs.x1);
int ny1 = max(y1,rhs.y1);
int nx2 = min(x2,rhs.x2);
int ny2 = min(y2,rhs.y2);
return Rectangle(nx1,ny1,nx2,ny2);
}
}river[MAXN],Forest;
vector<pair<pair<int,int>,int> > line[MAXN];
int n,p;
struct SegmentTree{
int sz[MAXN<<2],cover[MAXN<<2],l[MAXN<<2],r[MAXN<<2];
#define ls(rt) rt << 1
#define rs(rt) rt << 1 | 1
void pushup(int rt){
if(l[rt]+1==r[rt]){
if(cover[rt]) sz[rt] = 1;
else sz[rt] = 0;
return;
}
if(cover[rt]) sz[rt] = r[rt] - l[rt];
else sz[rt] = sz[ls(rt)] + sz[rs(rt)];
}
void build(int L, int R, int rt){
l[rt] = L, r[rt] = R;
sz[rt] = cover[rt] = 0;
if(L+1==R) return;
int mid = (L+R) >> 1;
build(L,mid,ls(rt));
build(mid,R,rs(rt));
}
void update(int L, int R, int rt, int x){
if(l[rt]>=R || L>=r[rt]) return;
if(L<=l[rt] && r[rt]<=R){
cover[rt] += x;
pushup(rt);
return;
}
update(L,R,ls(rt),x);
update(L,R,rs(rt),x);
pushup(rt);
}
int query(){ return sz[1]; }
}ST;
bool check(int mid){
for(int i = 0; i < MAXN; i++) line[i].clear();
for(int i = 1; i <= n; i++){
Rectangle tp(river[i].x1-mid,river[i].y1-mid,river[i].x2+mid,river[i].y2+mid);
tp = tp.intersect(Forest);
line[tp.y1].emplace_back(make_pair(make_pair(tp.x1,tp.x2),1));
line[tp.y2].emplace_back(make_pair(make_pair(tp.x1,tp.x2),-1));
}
LL area = 0;
ST.build(0,Forest.x2+1,1);
for(int i = Forest.y1; i <= Forest.y2; i++){
area += ST.query();
for(auto p : line[i]) ST.update(p.first.first,p.first.second,1,p.second);
}
return area*100 >= (LL(Forest.x2)-Forest.x1)*(Forest.y2-Forest.y1)*p;
}
int solve(){
int l = 1, r = MAXN;
while(l<=r){
int mid = (l+r) >> 1;
if(check(mid)) r = mid - 1;
else l = mid + 1;
}
return l;
}
int main(){
scanf("%d",&n);
for(int i = 1; i <= n; i++){
scanf("%d %d %d %d",&river[i].x1,&river[i].y1,&river[i].x2,&river[i].y2);
if(river[i].x1>river[i].x2) swap(river[i].x1,river[i].x2);
if(river[i].y1>river[i].y2) swap(river[i].y1,river[i].y2);
}
scanf("%d",&p);
scanf("%d %d %d %d",&Forest.x1,&Forest.y1,&Forest.x2,&Forest.y2);
if(Forest.x1>Forest.x2) swap(Forest.x1,Forest.x2);
if(Forest.y1>Forest.y2) swap(Forest.y1,Forest.y2);
printf("%d\n",solve());
return 0;
}
\(G.Getting\ Confidence\)
将乘法变成对数的加法然后就是KM匹配的模板了
//#pragma comment(linker, "/STACK:1024000000,1024000000")
#include<bits/stdc++.h>
using namespace std;
function<void(void)> ____ = [](){ios_base::sync_with_stdio(false); cin.tie(0); cout.tie(0);};
const int MAXN = 111;
const int INF = 0x3f3f3f3f;
int n,LX[MAXN],LY[MAXN],visx[MAXN],visy[MAXN],match[MAXN],slack[MAXN],G[MAXN][MAXN];
bool dfs(int x){
visx[x] = 1;
for(int y = 1; y <= n; y++){
if(visy[y]) continue;
int gap = LX[x] + LY[y] - G[x][y];
if(!gap){
visy[y] = 1;
if(match[y]==-1||(!visx[match[y]]&&dfs(match[y]))){
match[y] = x;
return true;
}
}
else slack[y] = min(slack[y],gap);
}
return false;
}
void KM_match(){
memset(match,255,sizeof(match));
for(int i = 1; i <= n; i++) LX[i] = *max_element(G[i]+1,G[i]+1+n);
memset(LY,0,sizeof(LY));
for(int i = 1; i <= n; i++){
memset(slack,INF,sizeof(slack));
while(true){
memset(visx,0,sizeof(visx));
memset(visy,0,sizeof(visy));
if(dfs(i)) break;
int d = INF;
for(int j = 1; j <= n; j++) if(!visy[j]) d = min(d,slack[j]);
for(int j = 1; j <= n; j++) if(visx[j]) LX[j]-=d;
for(int j = 1; j <= n ;j++) if(visy[j]) LY[j]+=d;
else slack[j]-=d;
}
}
for(int i = 1; i <= n; i++) printf("%d ",match[i]);
}
int main(){
scanf("%d",&n);
for(int i = 1; i <= n; i++) for(int j = 1; j <= n; j++){
scanf("%d",&G[i][j]);
G[i][j] = log(G[i][j])*1000000;
}
KM_match();
puts("");
return 0;
}
\(H.Hour\ for\ a\ Run\)
签到
//#pragma comment(linker, "/STACK:1024000000,1024000000")
#include<bits/stdc++.h>
using namespace std;
function<void(void)> ____ = [](){ios_base::sync_with_stdio(false); cin.tie(0); cout.tie(0);};
int n,m;
int main(){
____();
cin >> n >> m;
for(int i = 1; i < 10; i++) cout << int(ceil((n*m*i)/10.0)) << ' '; cout << endl;
return 0;
}
\(I.Interplanetary\)
离线之后按顺序加点跑floyd
//#pragma comment(linker, "/STACK:1024000000,1024000000")
#include<bits/stdc++.h>
using namespace std;
function<void(void)> ____ = [](){ios_base::sync_with_stdio(false); cin.tie(0); cout.tie(0);};
const int MAXN = 1e5+7;
const int INF = 0x3f3f3f3f;
struct Query{
int u,v,k,id;
Query(){}
Query(int u, int v, int k, int id){
this->u = u;
this->v = v;
this->k = k;
this->id = id;
}
}Q[2][MAXN];
int n,m,qs,res[MAXN],temp[MAXN],initG[444][444],q[2],G[444][444];
void solve(){
vector<int> tmp(n),nodes(n);
for(int i = 0; i < n; i++){
tmp[i]=temp[i+1];
nodes[i]=i+1;
}
sort(tmp.begin(),tmp.end());
tmp.erase(unique(tmp.begin(),tmp.end()),tmp.end());
function<bool(int,int)> cmp[2] = {
[](int x, int y){ return temp[x] < temp[y]; },
[](int x, int y){ return temp[x] > temp[y]; }
};
for(int op = 0; op <= 1; op++){
for(int i = 1; i <= n; i++) for(int j = 1; j <= n; j++) G[i][j] = initG[i][j];
sort(nodes.begin(),nodes.end(),cmp[op]);
if(!op) sort(tmp.begin(),tmp.end(),less<int>());
else sort(tmp.begin(),tmp.end(),greater<int>());
sort(Q[op]+1,Q[op]+1+q[op],[](const Query &x, const Query &y){ return x.k < y.k; });
for(int i = 1, cur = 0; i <= q[op]; i++){
while(cur<n){
if((!op&&temp[nodes[cur]]>tmp[Q[op][i].k-1])||(op&&temp[nodes[cur]]<tmp[Q[op][i].k-1])) break;
int node = nodes[cur++];
for(int u = 1; u <= n; u++) for(int v = 1; v <= n; v++)
G[u][v] = G[v][u] = min(G[u][v],G[u][node]+G[node][v]);
}
int u = Q[op][i].u, v = Q[op][i].v, ID = Q[op][i].id;
res[ID] = G[u][v]==INF?-1:G[u][v];
}
}
}
int main(){
scanf("%d %d",&n,&m);
for(int i = 1; i <= n; i++) scanf("%d",&temp[i]);
memset(initG,INF,sizeof(initG));
for(int i = 1; i <= n; i++) initG[i][i] = 0;
for(int i = 1; i <= m; i++){
int u, v, d;
scanf("%d %d %d",&u,&v,&d);
initG[u][v] = initG[v][u] = d;
}
scanf("%d",&qs);
for(int i = 1; i <= qs; i++){
int u, v, k, op;
scanf("%d %d %d %d",&u,&v,&k,&op);
Q[op][++q[op]] = Query(u,v,k,i);
}
solve();
for(int i = 1; i <= qs; i++) printf("%d\n",res[i]);
return 0;
}
\(J.Jar\ of\ Water\ Game\)
按题意模拟即可
//#pragma comment(linker, "/STACK:1024000000,1024000000")
#include<bits/stdc++.h>
using namespace std;
function<void(void)> ____ = [](){ios_base::sync_with_stdio(false); cin.tie(0); cout.tie(0);};
int n,m;
int dec(char ch){
if(ch=='A') return 1;
else if(ch=='D') return 10;
else if(ch=='Q') return 11;
else if(ch=='J') return 12;
else if(ch=='K') return 13;
else return ch-'0';
}
struct P{
bool wildcard,tag;
vector<int> card;
int get(){
if(wildcard){
if(tag) tag = false;
else{
wildcard = false;
return -1;
}
}
map<int,int> cards;
for(int c : card) cards[c]++;
int minn = 6;
for(auto p : cards) minn = min(minn,p.second);
for(auto p : cards){
if(p.second!=minn) continue;
card.erase(find(card.begin(),card.end(),p.first));
return p.first;
}
}
void insert(int c){
if(c==-1) wildcard = tag = true;
else card.emplace_back(c);
}
}player[20];
bool check(int ID){
if(player[ID].wildcard) return false;
if(player[ID].card[0]!=player[ID].card[1]) return false;
if(player[ID].card[1]!=player[ID].card[2]) return false;
if(player[ID].card[2]!=player[ID].card[3]) return false;
return true;
}
int main(){
scanf("%d %d",&n,&m);
for(int i = 1; i <= n; i++){
char cd[10];
scanf("%s",cd);
player[i].card.resize(4);
for(int k = 0; k < 4; k++) player[i].card[k] = dec(cd[k]);
if(i==m) player[i].wildcard = player[i].tag = true;
else player[i].wildcard = player[i].tag = false;
}
for(int i = 1; i <= n; i++) if(check(i)) return printf("%d\n",i),0;
int now = m;
while(true){
int nxt = now+1==n+1?1:now+1;
player[nxt].insert(player[now].get());
if(check(now)) break;
now = nxt;
}
printf("%d\n",now);
return 0;
}
\(K.Keep\ Calm\ and\ Sell\ Balloons\)
矩阵快速幂,要找出递推公式
首先我们令\(f[n]\)表示\(2·n\)的矩阵从一个角开始走回到它垂直位置的方案数,可以发现\(f[n]=2·f[n-1], f[1]=1\),即\(f[n]=2^{n-1}\)
现在我们定义\(g[n]\)为从矩阵的一个角开始走走完整个矩阵的方案数,模拟一下方案数可以找到递推式:\(g[n]=f[n]+2·g[n-1]+4·g[n-2]\)
即\(g[n]\ =\ 2·g[n-1]+4·g[n-2]+2^{n-1},g[0]=0,g[1]=1\)
\(f[n]\)容易得出,\(2·g[n-1]\)的情况在第一张图,\(4·g[n-2]\)的情况在第二张图
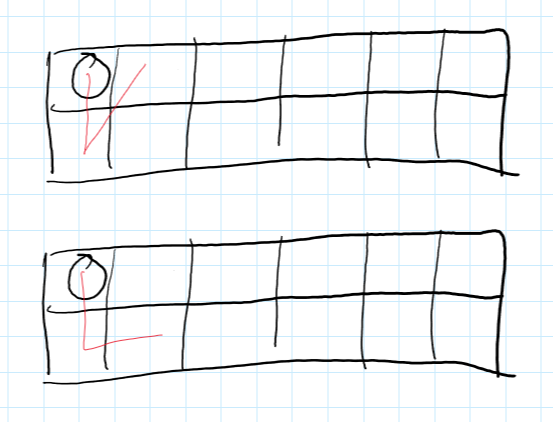
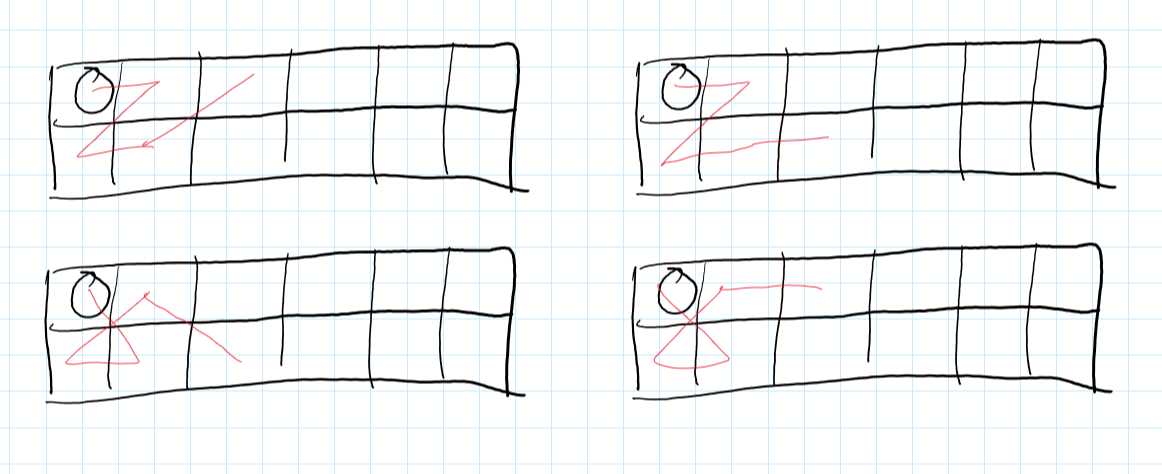
接下来我们定义\(h[n]\)为从矩阵的中间出发走完整个矩阵的方案数,可以把从中间出发走完整个矩阵分成两部分:
- 先从一边走回到垂直位置
- 再走完另一边
可以得到如下递推式:
\]
其中第一个\(2\)是因为有上下两块,第二个\(2\)是因为可以先向右回到垂直位置再走完左边,也可以先向左回到垂直位置再走完右边,方案是对称的
\]
\]
\]
然后找一下\(h[n]\)和\(h[n-1]\)的关系
\]
所以最后答案就是\(h[n]+4·g[n]\)
!注意啊要从\(2\)开始递推,\(g[2] \ne 2·g[1]+4·g[0]+f[2]\)
//#pragma comment(linker, "/STACK:1024000000,1024000000")
#include<bits/stdc++.h>
using namespace std;
function<void(void)> ____ = [](){ios_base::sync_with_stdio(false); cin.tie(0); cout.tie(0);};
using LL = int_fast64_t;
const LL MOD = 1e9+7;
struct Matrix{
LL m[6][6];
Matrix(int init = 1){
for(int i = 1; i <= 5; i++) for(int j = 1; j <= 5; j++){
if(i!=j) m[i][j] = 0;
else m[i][j] = init;
}
}
Matrix operator*(const Matrix rhs){
Matrix res(0);
for(int i = 1; i <= 5; i++){
for(int j = 1; j <= 5; j++){
for(int k = 1; k <= 5; k++)
res.m[i][j] = (res.m[i][j]+m[i][k]*rhs.m[k][j])%MOD;
}
}
return res;
}
Matrix operator*=(const Matrix rhs){
return *this = (*this)*rhs;
}
};
Matrix qpow(Matrix A, LL b){
Matrix res(1);
while(b){
if(b&1) res*=A;
b >>= 1;
A*=A;
}
return res;
}
LL n;
int main(){
scanf("%I64d",&n);
if(n==1) return puts("2"),0;
Matrix mat(0);
mat.m[1][1] = 2;
mat.m[1][3] = 16;
mat.m[2][2] = 2;
mat.m[2][3] = 4;
mat.m[2][4] = 2;
mat.m[3][2] = 1;
mat.m[4][4] = 2;
mat.m[5][5] = 1;
mat = qpow(mat,n-2);
printf("%I64d\n",(6*mat.m[1][2]+mat.m[1][3]+2*mat.m[1][4]+mat.m[1][5]+4*(6*mat.m[2][2]+mat.m[2][3]+2*mat.m[2][4]+mat.m[2][5]))%MOD);
return 0;
}
\(L.Less\ Coin\ Tosses\)
答案就是\(C(n,0)%2+C(n,1)%2+...+C(n,n-1)%2+C(n,n-1)%2\)
根据卢卡斯定理,答案就是2的\(N\)二进制位中1的个数次幂
//#pragma comment(linker, "/STACK:1024000000,1024000000")
#include<bits/stdc++.h>
using namespace std;
function<void(void)> ____ = [](){ios_base::sync_with_stdio(false); cin.tie(0); cout.tie(0);};
int_fast64_t n;
#define lowbit(x) ((x)&(-x))
int main(){
scanf("%I64d",&n);
int_fast64_t res = 1;
while(n) res <<= 1,n-=lowbit(n);
printf("%I64d\n",res);
return 0;
}
\(M.Maratona\ Brasileira\ de\ Popcorn\)
二分答案判定即可
//#pragma comment(linker, "/STACK:1024000000,1024000000")
#include<bits/stdc++.h>
using namespace std;
function<void(void)> ____ = [](){ios_base::sync_with_stdio(false); cin.tie(0); cout.tie(0);};
const int MAXN = 1e5+7;
using LL = int_fast64_t;
int n,c,t,A[MAXN];
bool check(int mid){
int cur = 0, tot = 1;
for(int i = 1; i <= n; i++){
if(mid*1ll*t<A[i]) return false;
if(mid*1ll*t>=cur+A[i]) cur+=A[i];
else{
tot++;
cur = A[i];
}
}
return tot<=c;
}
int solve(){
int l = 1, r = 1e9+7;
while(l<=r){
int mid = (l+r) >> 1;
if(check(mid)) r = mid - 1;
else l = mid + 1;
}
return l;
}
int main(){
scanf("%d %d %d",&n,&c,&t);
for(int i = 1; i <= n; i++) scanf("%d",&A[i]);
printf("%d\n",solve());
return 0;
}
2019-2020 ACM-ICPC Brazil Subregional Programming Contest (11/13)的更多相关文章
- (寒假GYM开黑)2018-2019 ACM-ICPC Brazil Subregional Programming Contest
layout: post title: 2018-2019 ACM-ICPC Brazil Subregional Programming Contest author: "luowenta ...
- 2018-2019 ACM-ICPC Brazil Subregional Programming Contest PART (10/13)
$$2018-2019 ACM-ICPC Brazil Subregional Programming Contest$$ \(A.Slackline\ Adventure\) \(B.Marbles ...
- 2018-2019 ACM-ICPC Brazil Subregional Programming Contest
A:留坑 B:二维sg函数,特判边界情况 //#pragma GCC optimize(2) //#pragma GCC optimize(3) //#pragma GCC optimize(4) / ...
- Gym.101908 Brazil Subregional Programming Contest(寒假自训第六场)
这几天睡眠时间都不太够,室友晚上太会折腾了,感觉有点累,所以昨天的题解也没写,看晚上能不能补起来. B . Marbles 题意:给定N组数(xi,yi),玩家轮流操作,每次玩家可以选择其中一组对其操 ...
- 2013-2014 ACM-ICPC Brazil Subregional Programming Contest 题解
[题目链接] 这场比赛题面英文都好长... ... A - Zero or One 模拟. #include <bits/stdc++.h> using namespace std; in ...
- ACM ICPC, JUST Collegiate Programming Contest (2018) Solution
A:Zero Array 题意:两种操作, 1 p v 将第p个位置的值改成v 2 查询最少的操作数使得所有数都变为0 操作为可以从原序列中选一个非0的数使得所有非0的数减去它,并且所有数不能 ...
- ACM ICPC, Amman Collegiate Programming Contest (2018) Solution
Solution A:Careful Thief 题意:给出n个区间,每个区间的每个位置的权值都是v,然后找长度为k的区间,使得这个区间的所有位置的权值加起来最大,输出最大权值, 所有区间不重叠 思路 ...
- Codeforces Gym101473 A.Zero or One (2013-2014 ACM-ICPC Brazil Subregional Programming Contest)
代码: #include<iostream> #include<cstring> #include<cstdio> #include<cmath> #i ...
- Codeforces Gym101473 F.Triangles-前缀和 (2013-2014 ACM-ICPC Brazil Subregional Programming Contest)
前缀和. 代码: 1 #include<iostream> 2 #include<cstring> 3 #include<cstdio> 4 #include< ...
随机推荐
- java环境配置-win10(傻瓜式教程)
java环境配置 – 小学弟要开始学java了,由于本人较懒,表达能力有限,所以来这,写一篇简单的指导,帮学弟装下java环境. 首先打开浏览器,输入这个网址https://www.oracle.co ...
- Java开发手册之数据库规约
1.不要使用 count(列名)或 count(常量)来替代 count(*),count(*)是 SQL92 定义的标准统计行数的语法,跟数据库无关,跟 NULL 和非 NULL 无关. 2.cou ...
- 在IDEA中通过Module管理多个项目
你身边有没有这种顽固的Eclipse忠实用户:IDEA不能一个窗口管理多个项目!太不方便了! 对于一个窗口同时管理多个项目的需求,在我们日常开发时候是经常需要的.尤其当我们在分布式环境下,在一个窗口中 ...
- drop table 命令不回收以前的相关访问权限
drop table 命令不回收以前的相关访问权限,也就是说假如我现在把表删除了,然后再创建一个同名的表时,会自动赋予权限的.
- HTML DOM 定义了访问和操作 HTML 文档标准
HTML DOM 定义了访问和操作 HTML 文档的标准. 您应该具备的基础知识 在您继续学习之前,您需要对以下内容拥有基本的了解: HTML CSS JavaScript 如果您需要首先学习这些项目 ...
- CodeMonkey少儿编程第2章 turnTo对象
目标 了解对象的概念 了解方法与对象的关系 掌握turnTo指令的用法 在开始本章的学习之前,我们先来复习一下上一章的知识点. 在第1章中,我们学会了在这个游戏中最简单的两个指令. step x 其中 ...
- mybatis中传集合时 报异常 invalid comparison: java.util.Arrays$ArrayList and java.lang.String
犯了一个低级的错误,在传集合类型的参数时,把他当成字符串处理了,导致报类型转换的错误 把 and nsrsbh!=' ' 删掉就行了
- Linux Centos7之由Python2升级到Python3教程
1.先查看当前系统Python版本,默认都是Python2.7,命令如下: [root@localhost gau]# python -V Python 2.7.5 2.安装Python3,安装方法很 ...
- 开心!再也不用担心 IntelliJ IDEA 试用过期了
背景 前段时间 Review 团队小伙伴代码,发现当他把鼠标挪到一个方法上时,就自动显示了该方法的所有注释信息,像下图这样,他和我用的 IDE 都是 IntelliJ IDEA. 而我还按古老的方式, ...
- 自监督SOTA框架 | BYOL(优雅而简洁) | 2020
文章原创自微信公众号「机器学习炼丹术」 作者:炼丹兄 联系方式:微信cyx645016617 本篇文章主要讲解两个无监督2020年比较新比较火的论文: 论文名称:"Bootstrap You ...
