CF455D Serega and Fun 题解

题目链接：CF 或者洛谷

本题是可以用平衡树去做的，具体的为每个 \(k\) 开一棵平衡树去维护相对位置，而这种移动操作用平衡树维护又是很容易做到的，这种做法是双 \(log\)。在 \(1e5\) 的数据下，我们来说说好写的分块该如何去写。

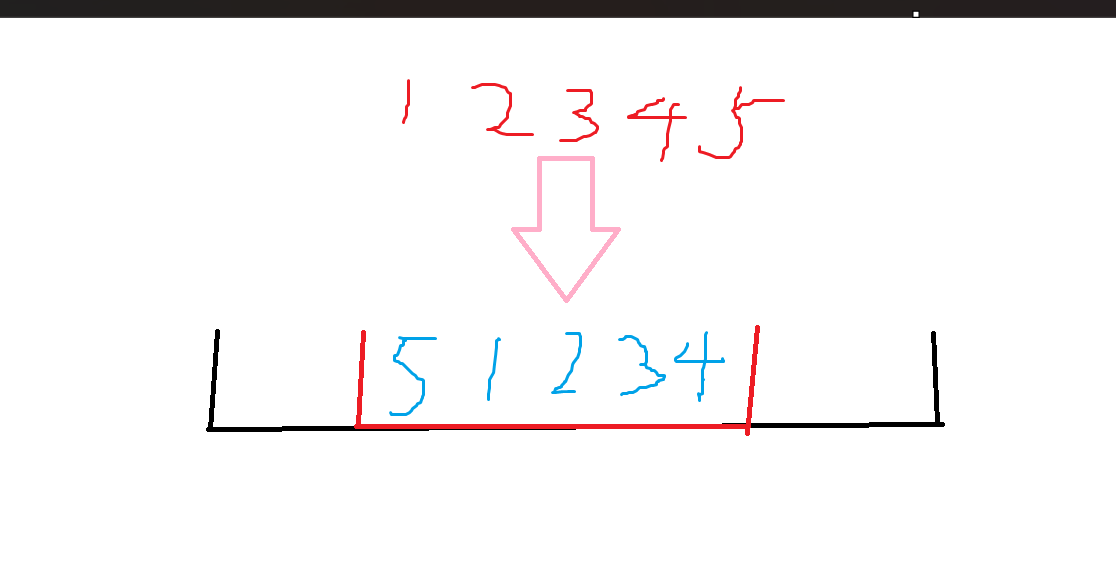
黑色的代表一个块，考虑暴力修改情况，假如原来的数字为 \([1,2,3,4,5]\) 显然变为了 \([2,3,4,5,1]\)，很显然，我们可以用一个基础的算法实现这样的一个功能，具体的：

令 \(pre=5\)，每次交换 \(a_i\) 与 \(pre\)，即让前一个数用一个变量保存，然后每次交换即可。当然，你也可以拷贝一份，然后直接做一次这种操作也行，反正就是将一个普通数组做一次这样的操作，你至少需要会怎么弄。

假如这是多块修改，中间整块怎么处理，我们容易发现：

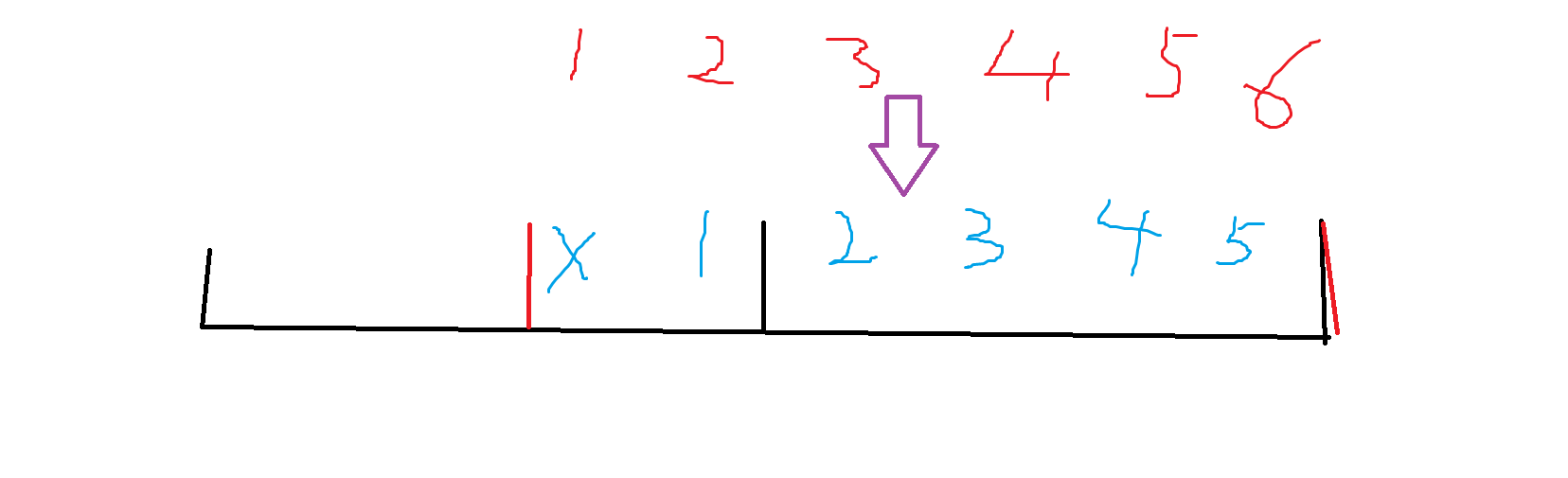
观察下这时候有啥变化，\(x\) 就是 \(r\) 对应的数，显然每个整块可以看做头部 \(+\) 了个上一个块的末尾数，尾部少了一个数去作为下一个块的头部的数，所以显然有一类数据结构可以很好地支持这样的一个功能，那就是双端队列，为每一个块维护双端队列，整块操作就可以优化到：\(O(1)\) 加入与修改，散块依旧暴力。

看看查询，问查 \(k\) 的数量，\(k\) 比较小，每个块开一个桶记录就行了，在做修改的时候注意维护就行了。到此结束。

参照代码

#include <bits/stdc++.h>

// #pragma GCC optimize("Ofast,unroll-loops")
// #pragma GCC optimize(2)

// #define isPbdsFile

#ifdef isPbdsFile

#include <bits/extc++.h>

#else

#include <ext/pb_ds/priority_queue.hpp>
#include <ext/pb_ds/hash_policy.hpp>
#include <ext/pb_ds/tree_policy.hpp>
#include <ext/pb_ds/trie_policy.hpp>
#include <ext/pb_ds/tag_and_trait.hpp>
#include <ext/pb_ds/hash_policy.hpp>
#include <ext/pb_ds/list_update_policy.hpp>
#include <ext/pb_ds/assoc_container.hpp>
#include <ext/pb_ds/exception.hpp>
#include <ext/rope>

#endif

using namespace std;
using namespace __gnu_cxx;
using namespace __gnu_pbds;
typedef long long ll;
typedef long double ld;
typedef pair<int, int> pii;
typedef pair<ll, ll> pll;
typedef tuple<int, int, int> tii;
typedef tuple<ll, ll, ll> tll;
typedef unsigned int ui;
typedef unsigned long long ull;
typedef __int128 i128;
#define hash1 unordered_map
#define hash2 gp_hash_table
#define hash3 cc_hash_table
#define stdHeap std::priority_queue
#define pbdsHeap __gnu_pbds::priority_queue
#define sortArr(a, n) sort(a+1,a+n+1)
#define all(v) v.begin(),v.end()
#define yes cout<<"YES"
#define no cout<<"NO"
#define Spider ios_base::sync_with_stdio(false);cin.tie(nullptr);cout.tie(nullptr);
#define MyFile freopen("..\\input.txt", "r", stdin),freopen("..\\output.txt", "w", stdout);
#define forn(i, a, b) for(int i = a; i <= b; i++)
#define forv(i, a, b) for(int i=a;i>=b;i--)
#define ls(x) (x<<1)
#define rs(x) (x<<1|1)
#define endl '\n'
//用于Miller-Rabin
[[maybe_unused]] static int Prime_Number[13] = {0, 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37};

template <typename T>
int disc(T* a, int n)
{
    return unique(a + 1, a + n + 1) - (a + 1);
}

template <typename T>
T lowBit(T x)
{
    return x & -x;
}

template <typename T>
T Rand(T l, T r)
{
    static mt19937 Rand(time(nullptr));
    uniform_int_distribution<T> dis(l, r);
    return dis(Rand);
}

template <typename T1, typename T2>
T1 modt(T1 a, T2 b)
{
    return (a % b + b) % b;
}

template <typename T1, typename T2, typename T3>
T1 qPow(T1 a, T2 b, T3 c)
{
    a %= c;
    T1 ans = 1;
    for (; b; b >>= 1, (a *= a) %= c)if (b & 1)(ans *= a) %= c;
    return modt(ans, c);
}

template <typename T>
void read(T& x)
{
    x = 0;
    T sign = 1;
    char ch = getchar();
    while (!isdigit(ch))
    {
        if (ch == '-')sign = -1;
        ch = getchar();
    }
    while (isdigit(ch))
    {
        x = (x << 3) + (x << 1) + (ch ^ 48);
        ch = getchar();
    }
    x *= sign;
}

template <typename T, typename... U>
void read(T& x, U&... y)
{
    read(x);
    read(y...);
}

template <typename T>
void write(T x)
{
    if (typeid(x) == typeid(char))return;
    if (x < 0)x = -x, putchar('-');
    if (x > 9)write(x / 10);
    putchar(x % 10 ^ 48);
}

template <typename C, typename T, typename... U>
void write(C c, T x, U... y)
{
    write(x), putchar(c);
    write(c, y...);
}

template <typename T11, typename T22, typename T33>
struct T3
{
    T11 one;
    T22 tow;
    T33 three;

    bool operator<(const T3 other) const
    {
        if (one == other.one)
        {
            if (tow == other.tow)return three < other.three;
            return tow < other.tow;
        }
        return one < other.one;
    }

    T3() { one = tow = three = 0; }

    T3(T11 one, T22 tow, T33 three) : one(one), tow(tow), three(three)
    {
    }
};

template <typename T1, typename T2>
void uMax(T1& x, T2 y)
{
    if (x < y)x = y;
}

template <typename T1, typename T2>
void uMin(T1& x, T2 y)
{
    if (x > y)x = y;
}

constexpr int N = 1e5 + 10;
constexpr int SIZE = sqrt(N);
constexpr int CNT = (N + SIZE - 1) / SIZE + 1;
int cnt[CNT][N]; //每个块维护桶
deque<int> val[N]; //每个块维护双端队列
int pos[N], s[N], e[N], idx[N]; //每个点的块编号,块起始和终止,每个点对应的块内编号(从0开始,即是对应双端队列的下标编号)
int a[N];
int n, q;

inline void update(const int l, const int r)
{
    const int L = pos[l], R = pos[r];
    if (L == R)
    {
        int pre = val[L][idx[r]];
        forn(i, l, r)swap(val[L][idx[i]], pre);
        return;
    }
    //注意每个块都有一个删除和增加的数
    int pre = val[R][idx[r]];
    cnt[R][pre]--;
    cnt[L][pre]++;
    forn(i, l, e[L])swap(val[L][idx[i]], pre);
    cnt[L][pre]--;
    forn(i, L+1, R-1)
    {
        val[i].push_front(pre), cnt[i][pre]++;
        pre = val[i].back(), val[i].pop_back(), cnt[i][pre]--;
    }
    cnt[R][pre]++;
    forn(i, s[R], r)swap(val[R][idx[i]], pre);
}

//查询常规查询即可
inline int query(const int l, const int r, const int k)
{
    const int L = pos[l], R = pos[r];
    int ans = 0;
    if (L == R)
    {
        forn(i, l, r)ans += val[L][idx[i]] == k;
        return ans;
    }
    forn(i, l, e[L])ans += val[L][idx[i]] == k;
    forn(i, s[R], r)ans += val[R][idx[i]] == k;
    forn(i, L+1, R-1)ans += cnt[i][k];
    return ans;
}

int last;

inline void solve()
{
    cin >> n;
    const int blockSize = sqrt(n);
    const int blockCnt = (n + blockSize - 1) / blockSize;
    forn(i, 1, n)cin >> a[i], pos[i] = (i - 1) / blockSize + 1, idx[i] = (i - 1) % blockSize;
    forn(i, 1, blockCnt)s[i] = (i - 1) * blockSize + 1, e[i] = i * blockSize;
    e[blockCnt] = n;
    forn(i, 1, blockCnt)
    {
        forn(j, s[i], e[i])
        {
            val[i].push_back(a[j]);
            cnt[i][a[j]]++;
        }
    }
    cin >> q;
    while (q--)
    {
        int op, l, r;
        cin >> op >> l >> r;
        l = (l + last - 1) % n + 1, r = (r + last - 1) % n + 1;
        if (l > r)swap(l, r);
        if (op == 1)update(l, r);
        else
        {
            int k;
            cin >> k;
            k = (k + last - 1) % n + 1;
            cout << (last = query(l, r, k)) << endl;
        }
    }
}

signed int main()
{
    // MyFile
    Spider
    //------------------------------------------------------
    // clock_t start = clock();
    int test = 1;
    //    read(test);
    // cin >> test;
    forn(i, 1, test)solve();
    //    while (cin >> n, n)solve();
    //    while (cin >> test)solve();
    // clock_t end = clock();
    // cerr << "time = " << double(end - start) / CLOCKS_PER_SEC << "s" << endl;
}

\[
时间复杂度为 \ O(n\sqrt{n})
\]