Codeforces Round #643 (Div. 2) 题解 (ABCDE)

目录

• A. Sequence with Digits
• B. Young Explorers
• C. Count Triangles
• D. Game With Array
• E. Restorer Distance

https://codeforces.com/contest/1355

打一半和室友开黑去了哈哈哈哈哈哈反正不计分（不要学我）

A. Sequence with Digits

这题我会证！首先对于百位来说，不可能从x跳到x+2，只能从x变成x+1或者不变（因为最大变化量为 \(9\times9=81\)）

这样的话大约1000次内，百位不可避免地从9变成0，这样min的值是0，变化量min*max就是一直是0了

#include <bits/stdc++.h>
using namespace std;
#define repeat(i,a,b) for(int i=(a),_=(b);i<_;i++)
#define repeat_back(i,a,b) for(int i=(b)-1,_=(a);i>=_;i--)
int cansel_sync=(ios::sync_with_stdio(0),cin.tie(0),0);
const int N=200010; typedef long long ll;
#define int ll
int a,k;
string s;
signed main(){
	int T; cin>>T;
	while(T--){
		cin>>a>>k;
		while(--k){
			s=to_string(a);
			int x=*min_element(s.begin(),s.end())-48;
			int y=*max_element(s.begin(),s.end())-48;
			if(x==0)break;
			a+=x*y;
		}
		cout<<a<<endl;
	}
	return 0;
}

B. Young Explorers

比赛中我自始至终都以为所有探险者都要组队，然后emmm...

贪心，尽量组规模小的队，所以排个序就好了

感谢zkx巨佬提供的代码（没错，我懒了补题）

#include <bits/stdc++.h>
using namespace std;
const int N = 2e5+7;

int n;
int a[N];

inline void solve() {
  cin >> n;
  for (int i = 1; i <= n; ++i) {
    cin >> a[i];
  }
  sort(a+1, a+n+1);
  int res = 0;
  for (int i = 1, cnt = 0; i <= n; ++i) {
    ++cnt;
    if (cnt >= a[i]) {
      ++res;
      cnt = 0;
    }
  }
  cout << res << endl;
}

signed main() {
  ios::sync_with_stdio(false); cin.tie(NULL); cout.tie(NULL);
  int T = 1;
  cin >> T;
  for (int t = 1; t <= T; ++t) {
    solve();
  }
  return 0;
}

C. Count Triangles

先讲一下我的思路

\(A≤x≤B≤y≤C≤z≤D\)

因为 \(x\) 范围不大，考虑枚举 \(x\) 的值。这样对于任意满足要求的 \(y\)，都有 \(C\le z\le \min(D,x+y-1)\)，或者说，\(z\) 的方案数为 \(\max(0,\min(D,x+y-1)-C+1)\)。令这个东西为 \(f(y)=\max(0,\min(D,x+y-1)-C+1)\)，函数图像大概长这样

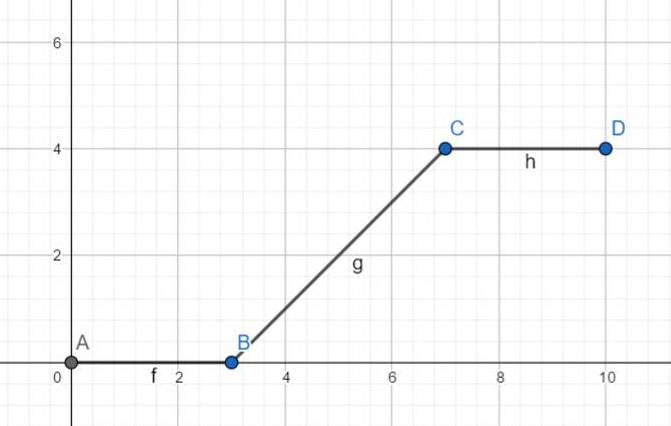
先一次函数上升，然后变成常函数。此时就能成两段讨论（一次函数和常函数），求出两个转折点什么的，太过于数学，逃了

然后放个没人能看懂的代码（经刘老师提醒，2020-5-17 11:45之前放的是错误代码，现已更正）

#include <bits/stdc++.h>
using namespace std;
#define repeat(i,a,b) for(int i=(a),_=(b);i<_;i++)
#define repeat_back(i,a,b) for(int i=(b)-1,_=(a);i>=_;i--)
int cansel_sync=(ios::sync_with_stdio(0),cin.tie(0),0);
const int N=200010; typedef long long ll;
#define int ll
int a,b,c,d;
signed main(){
	cin>>a>>b>>c>>d;
	int ans=0;
	repeat(i,a,b+1){
		int x=max(c,i+b-1); //第一个转折点
		int y=min(i+c-1,d); //第二个转折点
		int l=x-c+1,r=y-c+1;
		if(x<=y)ans+=(l+r)*(r-l+1)/2; //一次函数（等差数列求和）
		if(i+c-1>max(x-1,y))ans+=(i+c-1-max(x-1,y))*(d-c+1); //常函数
	}
	cout<<ans<<endl;
	return 0;
}

等会补个别人家的代码（雾，我作业来不及了，不补了）

D. Game With Array

这题就一sb题。如果 \(2N> S\)，这意味着序列中至少存在一个1，然后。。就NO了（还没证明）

如果 \(2N\le S\)，那就构造一个序列：\([2,2,2,...,2,(S-2(N-1))]\)，所有数都大于等于 \(2\)，所以这个序列的任意子序列之和都不可能是 \(1\)，也不可能是 \(S-1\)（因为存在子序列和为 \(S-1\) 就必然存在子序列和为 \(1\)）。而子串又是一种特殊的子序列，所以完全ojbk

更：看了一下官方题解，来证明一波 \(2N>S\) 的情况

先假定 \(K\le \dfrac S 2\)。对序列求个前缀和 \(sum[i]=\sum\limits_{j=1}^{i}a[j]\)，\(sum[0]=0\)。我们知道区间和相当于两个前缀和的差值，而这个差值不能等于 \(K\) 或者 \(S-K\)，这意味着对于每个 \(sum[i]\) 都不能出现另一个 \(sum[j]=(sum[i]+K)\%S\)（非常巧妙，如果 \(sum[i]+K\ge S\)，取模后正好等于 \(sum[i]-(S-K)\)，差值为 \(S-K\)，太牛逼了）

也就是，对每个 \(sum[i]\)，我们认为它占用了两个位置 \(sum[i]\) 和 \((sum[i]+K)\%S\)。总共 \(S\) 个座位，要坐 \(2N\) 个人，还有 \(2N>S\) 的限制，想想都不可能

#include <bits/stdc++.h>
using namespace std;
#define repeat(i,a,b) for(int i=(a),_=(b);i<_;i++)
#define repeat_back(i,a,b) for(int i=(b)-1,_=(a);i>=_;i--)
int cansel_sync=(ios::sync_with_stdio(0),cin.tie(0),0);
const int N=200010; typedef long long ll;
#define int ll
int n,s;
signed main(){
	cin>>n>>s;
	if(2*n<=s){
		cout<<"YES"<<endl;
		repeat(i,0,n-1)cout<<2<<' ';
		cout<<s-(n-1)*2<<endl;
		cout<<1<<endl;
	}
	else
		cout<<"NO"<<endl;
	return 0;
}

E. Restorer Distance

我们可以很轻松地算出，如果最终状态为每个位置高度为 \(H\) 的代价是多少。因此 \(H\) 的选取就成为本题的关键

大佬们就能一眼看出，代价对 \(H\) 存在单峰（随 \(H\) 先递减后递增），因此三分。那么为什么存在单峰呢？太难顶了，留作习题答案略（qwq）

我的三分其实就是二分，感觉能加一点速（？）感觉最快的可能是1.618优选法，但是我写不来（？）

我的方法是 \(O(n\log n\))，标程是 \((\log^2n)\) 因为处理了前缀和，get_cost() 里只要 lowerbound 一下就能算答案了，真是妙啊

#include <bits/stdc++.h>
using namespace std;
#define repeat(i,a,b) for(int i=(a),_=(b);i<_;i++)
#define repeat_back(i,a,b) for(int i=(b)-1,_=(a);i>=_;i--)
int cansel_sync=(ios::sync_with_stdio(0),cin.tie(0),0);
const int N=100010; typedef long long ll;
#define int ll
int n,A,B,C;
int a[N];
int get_cost(int h){
	int up=0,down=0;
	repeat(i,0,n)
		(h>a[i]?up:down)+=abs(a[i]-h);
	int t=min(up,down);
	return (up-t)*A+t*B+(down-t)*C;
}
signed main(){
	cin>>n>>A>>C>>B; B=min(B,A+C);
	repeat(i,0,n)
		cin>>a[i];
	int l=0,r=1e9+1;
	while(l<r){
		int x=(l+r)/2,y=x+1;
		if(get_cost(x)<get_cost(y))r=y-1;
		else l=x+1;
	}
	cout<<get_cost(l)<<endl;
	return 0;
}