POJ 2528 Mayor’s posters

Mayor's posters

Time Limit: 1000MS		Memory Limit: 65536K
Total Submissions: 37982		Accepted: 11030

Description

The citizens of Bytetown, AB, could not stand that the candidates in the mayoral election campaign have been placing their electoral posters at all places at their whim. The city council has finally decided to build an electoral wall for placing the posters and introduce the following rules:

• Every candidate can place exactly one poster on the wall.
• All posters are of the same height equal to the height of
  the wall; the width of a poster can be any integer number of bytes (byte
  is the unit of length in Bytetown).
• The wall is divided into segments and the width of each segment is one byte.
• Each poster must completely cover a contiguous number of wall segments.

They have built a wall 10000000 bytes long (such that there is
enough place for all candidates). When the electoral campaign was
restarted, the candidates were placing their posters on the wall and
their posters differed widely in width. Moreover, the candidates started
placing their posters on wall segments already occupied by other
posters. Everyone in Bytetown was curious whose posters will be visible
(entirely or in part) on the last day before elections.

Your task is to find the number of visible posters when all the
posters are placed given the information about posters' size, their
place and order of placement on the electoral wall.

Input

The
first line of input contains a number c giving the number of cases that
follow. The first line of data for a single case contains number 1 <=
n <= 10000. The subsequent n lines describe the posters in the order
in which they were placed. The i-th line among the n lines contains two
integer numbers l_i and ri which are the number of the wall
segment occupied by the left end and the right end of the i-th poster,
respectively. We know that for each 1 <= i <= n, 1 <= l_i <= ri <= 10000000. After the i-th poster is placed, it entirely covers all wall segments numbered l_i, l_i+1 ,... , ri.

Output

For each input data set print the number of visible posters after all the posters are placed.

The picture below illustrates the case of the sample input.

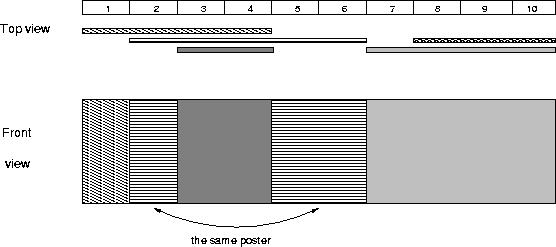
Sample Input

1
5
1 4
2 6
8 10
3 4
7 10

Sample Output

4

题目意思是在墙上贴海报,海报可以相互覆盖,问你最后可以看见几张海报。

因为数据量大，若不离散化会超时或超内存。故学习了别人写法，简单的hash后再线段树成段更新。

#include<cstdio>
#include<cstring>
#include<iostream>
#include<algorithm>
using namespace std;
#define lson l , m , rt << 1
#define rson m + 1 , r , rt << 1 | 1
#define maxn  10010
bool hash[maxn];
int li[maxn] , ri[maxn];
int X[maxn<<4];
int col[maxn<<4];
int cnt;
void PushDown(int rt)
{
    if (col[rt] != -1)
    {
        col[rt<<1] = col[rt<<1|1] = col[rt];
        col[rt] = -1;
    }
}
void update(int L,int R,int c,int l,int r,int rt)
{
    if (L<=l && r<=R)
    {
        col[rt] = c;
        return ;
    }
    PushDown(rt);
    int m =(l+r)>> 1;
    if (L<=m) update(L , R , c , lson);
    if (m<R) update(L , R , c , rson);
}
void query(int l,int r,int rt)
{
    if (col[rt] != -1)
    {
        if (!hash[col[rt]]) cnt ++;
        hash[ col[rt] ] = true;
        return ;
    }
    if (l == r) return ;
    int m = (l + r)>>1;
    query(lson);
    query(rson);
}
int Bin(int key,int n,int X[])
{
    int l = 0 , r = n - 1;
    while (l <= r)
    {
        int m = (l + r) >> 1;
        if (X[m] == key) return m;
        if (X[m] < key) l = m + 1;
        else r = m - 1;
    }
    return -1;
}
int main()
{
    int T , n;
    scanf("%d",&T);
    while (T --)
    {
        scanf("%d",&n);
        int nn = 0;
        for (int i=0; i<n; i ++)
        {
            scanf("%d%d",&li[i],&ri[i]);
            X[nn++] = li[i];
            X[nn++] = ri[i];
        }
        sort(X , X+nn);
        int m = 1;
        for (int i = 1 ; i < nn; i ++)
        {
            if (X[i] != X[i-1]) X[m ++] = X[i];
        }
        for (int i = m - 1 ; i > 0 ; i --)
        {
            if (X[i] != X[i-1] + 1) X[m ++] = X[i-1] + 1;
        }
        sort(X , X + m);
        memset(col , -1 , sizeof(col));
        for (int i = 0 ; i < n ; i ++)
        {
            int l = Bin(li[i], m, X);
            int r = Bin(ri[i], m, X);
            update(l, r, i, 0, m - 1, 1);
        }
        cnt = 0;
        memset(hash, false, sizeof(hash));
        query(0, m - 1, 1);
        printf("%d\n",cnt);
    }
    return 0;
}