poj 2553 The Bottom of a Graph(强连通分量+缩点)

题目地址：http://poj.org/problem?id=2553

The Bottom of a Graph

Time Limit: 3000MS		Memory Limit: 65536K
Total Submissions: 7881		Accepted: 3263

Description

We will use the following (standard) definitions from graph theory. Let V be a nonempty and finite set, its elements being called vertices (or nodes). Let E be a subset of the Cartesian product V×V, its elements being called edges. Then G=(V,E) is called a directed graph.  Let n be a positive integer, and let p=(e₁,...,e_n) be a sequence of length n of edges e_i∈E such that e_i=(v_i,v_i+1) for a sequence of vertices (v₁,...,v_n+1). Then p is called a path from vertex v₁ to vertex v_n+1 in G and we say that v_n+1 is reachable from v₁, writing (v₁→v_n+1).  Here are some new definitions. A node v in a graph G=(V,E) is called a sink, if for every node w in G that is reachable from v, v is also reachable from w. The bottom of a graph is the subset of all nodes that are sinks, i.e.,bottom(G)={v∈V|∀w∈V:(v→w)⇒(w→v)}. You have to calculate the bottom of certain graphs.

Input

The input contains several test cases, each of which corresponds to a directed graph G. Each test case starts with an integer number v, denoting the number of vertices of G=(V,E), where the vertices will be identified by the integer numbers in the set V={1,...,v}. You may assume that 1<=v<=5000. That is followed by a non-negative integer e and, thereafter, e pairs of vertex identifiers v₁,w₁,...,v_e,w_e with the meaning that (v_i,w_i)∈E. There are no edges other than specified by these pairs. The last test case is followed by a zero.

Output

For each test case output the bottom of the specified graph on a single line. To this end, print the numbers of all nodes that are sinks in sorted order separated by a single space character. If the bottom is empty, print an empty line.

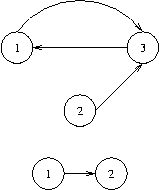
Sample Input

3 3
1 3 2 3 3 1
2 1
1 2
0

Sample Output

1 3
2

Source

Ulm Local 2003

 

【题解】：

　　找到入度为0的所有强连通分量，将其中的点排序后输出

　　这题跟poj 2186 很类似

　　

【code】：

　　

 /**
 Judge Status:Accepted      Memory:1488K
 Time:125MS      Language:G++
 Code Length:2443B   Author:cj
 */
 #include<iostream>
 #include<stdio.h>
 #include<string.h>
 #include<stack>
 #include<vector>
 #include<algorithm>

 #define N 10010
 using namespace std;

 vector<int> G[N];
 stack<int> stk;
 int pre[N],lowlink[N],sccno[N],scc_cnt,dfn_clock,out[N],counter[N];

 void DFN(int u)  //tarjan算法
 {
     lowlink[u] = pre[u] = ++dfn_clock;
     stk.push(u);
     int i;
     for(i=;i<G[u].size();i++)
     {
         int v = G[u][i];
         if(!pre[v])
         {
             DFN(v);
             lowlink[u] = min(lowlink[u],lowlink[v]);
         }
         else if(!sccno[v])
         {
             lowlink[u] = min(lowlink[u],pre[v]);
         }
     }
     if(lowlink[u]==pre[u])
     {
         scc_cnt++;  //强连通图的个数标记
         while()
         {
             int x = stk.top();
             stk.pop();
             sccno[x] = scc_cnt;
             if(x==u)    break;
         }
     }
 }

 void findscc(int n)
 {
     int i;
     scc_cnt = dfn_clock = ;
     memset(pre,,sizeof(pre));
     memset(lowlink,,sizeof(lowlink));
     memset(sccno,,sizeof(sccno));
     for(i=;i<=n;i++)
         if(!pre[i])
             DFN(i);
 }

 int main()
 {
     int n,m;
     while(~scanf("%d",&n)&&n)
     {
         scanf("%d",&m);
         int i;
         for(i=;i<=n;i++)
             G[i].clear();
         for(i=;i<m;i++)
         {
             int a,b;
             scanf("%d%d",&a,&b);
             G[a].push_back(b);  // 得到图
         }
         findscc(n);  //查找强连通图
         int j;
         memset(out,,sizeof(out));
         memset(counter,,sizeof(counter));

         for(i=;i<=n;i++)  //遍历一边图，查找统计个点缩点后的出度
         {
             for(j=;j<G[i].size();j++)
             {
                 int v = G[i][j];
                 if(sccno[i]!=sccno[v])
                 {
                     out[sccno[i]]++;  //出度
                 }
             }
         }
         for(i=;i<=scc_cnt;i++)
         {
             if(!out[i])  //出度为0的强连通分量
             {
                 counter[i] = ;  //标记
             }
         }

         int pl = ;
         for(i=;i<=n;i++)
             if(counter[sccno[i]])  //是否被标记，从下到大
             {
                 if(pl)  printf(" %d",i);
                 else printf("%d",i);
                 pl = ;
             }
         putchar();
     }
     return ;
 }