atcoder ABC233

B

题意

给一个字符串， 可以把第一个字母移到最后， 也可以把最后一个字母放第一个， 问字典序最大最小的字符串。

题解

把第一个放最后一个， 相当于把最后一个放第一个执行n-1次， 那么我们不妨只进行第一步操作， 把所有的结果都算出来， 排序即可； 注：提取string的子串方法：a.substr(i, j); 从第i位开始， 长度为j的字符串(开头是0)；

D

题意

构造一个n的全排列， 使\(a_i\)在\(b_i\)前面；

题解

非常简单， 建边判环即可， 判环和记录答案都可以用topsort, 不过统计答案的时候要用堆优化, 应该可以写到一个函数里面

代码

#include <bits/stdc++.h>
using namespace std;
typedef long long ll;
const int INF = 0x3f3f3f3f;
const int N = 2e5 + 10;
const int M = 5e3 + 10;
const int eps = 1e-6; 
template < typename T > inline void read(T &x) {
	x = 0; T ff = 1, ch = getchar();
	while (!isdigit(ch)) {
		if (ch == '-') ff = -1;
		ch = getchar();
	}
	while (isdigit(ch)) {
		x = (x << 1) + (x << 3) + (ch ^ 48);
		ch = getchar();
	}
	x *= ff;
}
int n, m, c[N], du[N], vi[N];
map < int, int > p[N];
vector < int > v[N], a;
inline bool topsort() {
	queue < int > q;
	for (int i = 1; i <= n; ++i)
		if (c[i] == 0) q.push(i);
	while (!q.empty()) {
		int x = q.front();
		q.pop();
		for (int i = 0; i < v[x].size(); ++i) {
			int y = v[x][i];
			--c[y];
			if (c[y] == 0) q.push(y);
		}
	}
	for (int i = 1; i <= n; ++i)
		if (c[i]) return false;
	return true;
} 
inline void solve() {
	priority_queue < int > q;
	for (int i = 1; i <= n; ++i)
		if (du[i] == 0) q.push(-i);
	while (!q.empty()) {
		int x = -q.top();
		q.pop();
		a.push_back(x);
		for (int i = 0; i < v[x].size(); ++i) {
			int y = v[x][i];
			--du[y];
			if (du[y] == 0) q.push(-y);
		}
	}
	for (int i = 0; i < n; ++i) printf("%d ", a[i]);
}
int main() {
	read(n), read(m);
	for (int i = 1; i <= m; ++i) {
		int x, y;
		read(x), read(y);
		if (p[x][y]) continue;
		p[x][y] = true;
		v[x].push_back(y);
		++du[y];
		++c[y];
	}
	if (!topsort()) puts("-1");
	else solve();
	return 0;
}

---

E

题意

题目不是很好理解， 有个x*y的网格， 要放入三个面积不小于a, b, c且边长都为整数的矩形， 判断是否成立。

题解

当有两个矩形的时候， 存在一条线， 把两个矩形分开。 当三个矩形的时候， 存在一条线， 分成一边一个矩形，一边两个矩形。 那我们就枚举这个矩形，在枚举x或y,使这条边充分利用， 算出len=\(\lceil\frac{S}{x}\rceil\), 或 len=\(\lceil\frac{S}{y}\rceil\), 从而把边长减去len, 转换成两个矩形的问题， 同上

代码

#include <bits/stdc++.h>
using namespace std;
typedef long long ll;
const int INF = 0x3f3f3f3f;
const int N = 2e5 + 10;
const int M = 5e3 + 10;
const int eps = 1e-6; 
template < typename T > inline void read(T &x) {
	x = 0; T ff = 1, ch = getchar();
	while (!isdigit(ch)) {
		if (ch == '-') ff = -1;
		ch = getchar();
	}
	while (isdigit(ch)) {
		x = (x << 1) + (x << 3) + (ch ^ 48);
		ch = getchar();
	}
	x *= ff;
}
ll n, m, a, b, c;
inline bool solve2(ll x, ll y, ll u, ll v) {
	for (int i = 0; i < 2; ++i) {
		ll len = (u + x - 1) / x;
		if (len < y && x * (y - len) >= v) return true;
		swap(x, y);
	}
	return false;
}
inline bool solve3(ll x, ll y, ll u, ll v, ll w) {
	for (int i = 0; i < 2; ++i) {
		for (int j = 0; j < 3; ++j) {
			ll len = (u + x - 1) / x;
			if (len < y && solve2(x, y - len, v, w)) return true;
			swap(u, v);
			swap(v, w);
		}
		swap(x, y);
	}
	return false;
}
int main() {
	read(n), read(m), read(a), read(b), read(c);
	puts(solve3(n, m, a, b, c) ? "Yes" : "No");
	return 0;
}

F

在看这道题之前, 我们先引入一道题

题目

我们再引入一个题解

题解

题解是链上的做法, 引申到树上即可.(吐槽一波, csp我竟然看错题了)

上题



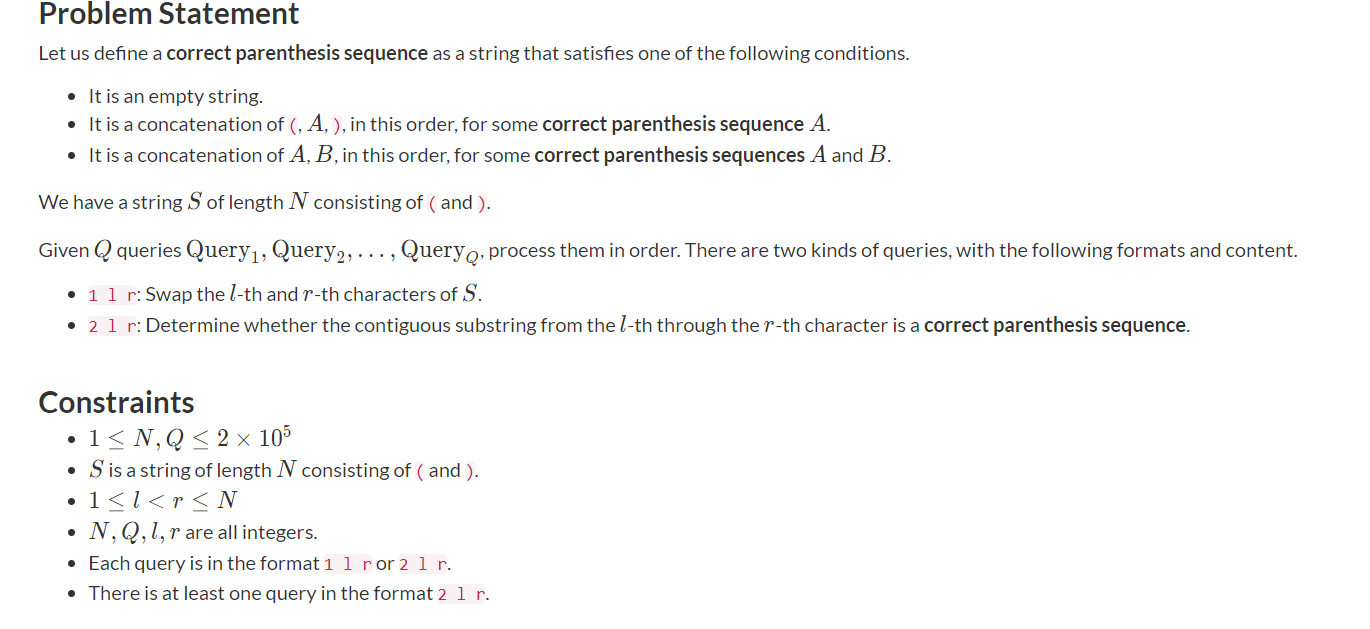
题目的意思是, 有一个长度为n的括号序列, 有两个操作, 一是交换l, r的括号, 二是求l到r之间是不是完美匹配. 有了上面的铺垫, 我们很显然知道, 一段区间是合法的, 必须满足, a[l - 1] = a[r], 且a[l ~ r - 1] >= a[l - 1] (a[r]), 那我们交换两个不同的括号有什么影响呢, 假如是左边的左括号和右边的右括号交换, 那么a[l ~ r - 1], 全部减去2, 反之同理, 看到这个, 那么我们就知道要用数据结构来维护这个a数组了, 线段树显然可以, 当然需要懒标记.

代码

#include <bits/stdc++.h>
using namespace std;
//typedef long long ll;
const int INF = 0x3f3f3f3f;
const int N = 2e5 + 10;
const int M = 1e6 + 10;
//const int mod = 1e9 + 7;
//const double eps = 1e-6; 
template < typename T > inline void read(T &x) {
	x = 0; T ff = 1, ch = getchar();
	while (!isdigit(ch)) {
		if (ch == '-') ff = -1;
		ch = getchar();
	}
	while (isdigit(ch)) {
		x = (x << 1) + (x << 3) + (ch ^ 48);
		ch = getchar();
	}
	x *= ff;
}
char ch[N];
int n, m, a[N];
struct tree {
	int l, r;
	int dat, lazy;
}t[N << 2];
inline void build(int x, int l, int r) {
	t[x].l = l, t[x].r = r;
	if (l == r) {
		t[x].dat = a[l];
		return;
	}
	int mid = l + r >> 1;
	build(x << 1, l, mid);
	build(x << 1 | 1, mid + 1, r);
	t[x].dat = min(t[x << 1].dat, t[x << 1 | 1].dat);
}
inline void push_down(int x) {
	if (t[x].lazy != 0) {
		t[x << 1].lazy += t[x].lazy;
		t[x << 1 | 1].lazy += t[x].lazy;
		t[x << 1].dat += t[x].lazy;
		t[x << 1 | 1].dat += t[x].lazy;
//		t[x].dat = min(t[x << 1].dat, t[x << 1 | 1].dat);
		t[x].lazy = 0;
	}
} 
inline void change(int x, int L, int R, int c) {
	int l = t[x].l, r = t[x].r;
	if (L <= l && R >= r) {
		t[x].dat += c;
		t[x].lazy += c;
		return;
	}
	push_down(x);
	int mid = l + r >> 1;
	if (mid >= L) change(x << 1, L, R, c);
	if (mid < R) change(x << 1 | 1, L, R, c);
	t[x].dat = min(t[x << 1].dat, t[x << 1 | 1].dat);
}
inline int query(int x, int L, int R) {
	int l = t[x].l, r = t[x].r;
	if (l >= L && r <= R) return t[x].dat;
	push_down(x);
	int ans = INF;
	int mid = l + r >> 1;
	if (mid >= L) ans = min(ans, query(x << 1, L, R));
	if (mid < R) ans = min(ans, query(x << 1 | 1, L, R));
//	t[x].dat = (t[x << 1].dat, t[x << 1 | 1].dat);
	return ans;
}
int main() {
	read(n); read(m);
	scanf("%s", ch + 1);
	for (int i = 1; i <= n; ++i) {
		if (ch[i] == '(') a[i] = a[i - 1] + 1;
		else a[i] = a[i - 1] - 1;
	}
	build(1, 0, n);
	for (int i = 1; i <= m; ++i) {
		int op, l, r;
		read(op), read(l), read(r);
		if (op == 1) {
			if (ch[l] == ch[r]) continue;
			if (ch[l] == '(') change(1, l, r - 1, -2);
			else change(1, l, r - 1, 2);
			swap(ch[l], ch[r]);
		} else {
			int x, y, z;
			x = query(1, l - 1, l - 1);
			y = query(1, l, r);
			z = query(1, r, r);
			if (x == y && y == z) puts("Yes");
			else puts("No");
		}
	}
	return 0;
}