leetcode-回溯②-难题

题10:

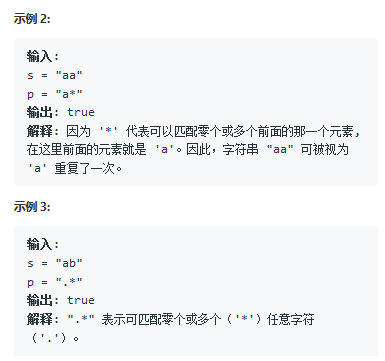
回溯：另：动态规划复杂度更低

class Solution:
    def isMatch(self, s: str, p: str) -> bool:
        def helper(s,p):
            if not p:
                return not bool(s)
            if len(p)>=2 and p[1]=="*":
                if s and (p[0] == "." or p[0] == s[0]) :
                    return helper(s[1:],p) or helper(s,p[2:])
                else:
                    return helper(s,p[2:])
            else  :
                if s and (p[0] == "." or p[0] == s[0]) :
                    return helper(s[1:],p[1:])
            return False
        return helper(s,p)

题37：

题52：

class Solution:
    def solveNQueens(self, n: int) -> List[List[str]]:
        res = []
        s = "." * n
        def backtrack(i, tmp,col,z_diagonal,f_diagonal):
            if i == n:
                res.append(tmp)
                return
            for j in range(n):
                if j not in col and i + j not in  z_diagonal and i - j not in f_diagonal:
                    backtrack(i+1,tmp + [s[:j] + "Q" + s[j+1:]], col | {j}, z_diagonal |{i + j} , f_diagonal |{i - j}  ) 

        backtrack(0,[],set(),set(),set())
        return res