HDU 5299 Circles Game

HDU 5299

思路：

圆扫描线+树上删边博弈

圆扫描线有以下四种情况，用set维护扫描线与圆的交点，重载小于号

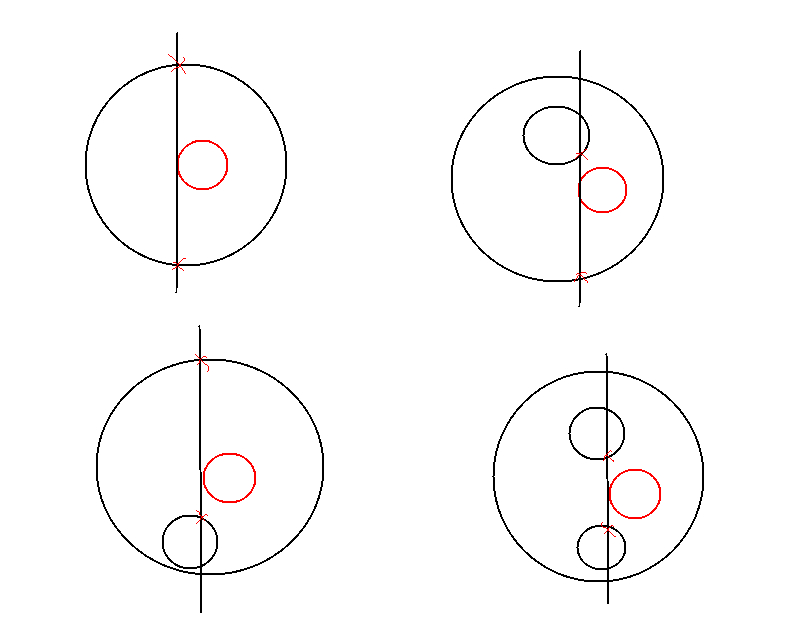
代码：

#pragma GCC optimize(2)
#pragma GCC optimize(3)
#pragma GCC optimize(4)
#include<bits/stdc++.h>
using namespace std;
#define y1 y11
#define fi first
#define se second
#define pi acos(-1.0)
#define LL long long
//#define mp make_pair
#define pb push_back
#define ls rt<<1, l, m
#define rs rt<<1|1, m+1, r
#define ULL unsigned LL
#define pll pair<LL, LL>
#define pli pair<LL, int>
#define pii pair<int, int>
#define piii pair<pii, int>
#define pdd pair<double, double>
#define mem(a, b) memset(a, b, sizeof(a))
#define debug(x) cerr << #x << " = " << x << "\n";

const int N = 2e4 + ;
int nowx;
struct circle {
    int x, y, r;
}p[N];
double Y(int id, int ty) {
    if(ty == ) return p[id].y - sqrt(p[id].r*1.0*p[id].r - (nowx-p[id].x)*1.0*(nowx-p[id].x));
    else return p[id].y + sqrt(p[id].r*1.0*p[id].r - (nowx-p[id].x)*1.0*(nowx-p[id].x));
}
struct node {
    int id, ty;
    bool operator < (const node &rhs) const {
        if(id == rhs.id) return ty < rhs.ty;
        else return Y(id, ty) < Y(rhs.id, rhs.ty);
    }
};
set<node> s;
vector<int> g[N];
int T, n, dp[N], fa[N], sg[N];
piii t[N*];
void dfs(int u, int o) {
    sg[u] = ;
    for (int i = ; i < g[u].size(); ++i) {
        int v = g[u][i];
        if(v != o) {
            dfs(v, u);
            sg[u] ^= sg[v] + ;
        }
    }
}
int main() {
    p[].x = p[].y = ;
    p[].r = ;
    s.insert({, });
    s.insert({, });
    scanf("%d", &T);
    while(T--) {
        scanf("%d", &n);
        for (int i = ; i <= n; ++i) scanf("%d %d %d", &p[i].x, &p[i].y, &p[i].r);
        for (int i = ; i <= n; ++i) {
            t[i].fi.fi = p[i].x - p[i].r;
            t[i].fi.se = ;
            t[i].se = i;
            t[n+i].fi.fi = p[i].x + p[i].r;
            t[n+i].fi.se = ;
            t[n+i].se = i;
        }
        sort(t+, t++*n);
        for (int i = ; i <= *n; ++i) {
            nowx = t[i].fi.fi;
            int id = t[i].se;
            node tmp = {id, };
            if(t[i].fi.se == ) {
                auto l = s.lower_bound(tmp); --l;
                auto r = s.upper_bound(tmp);
                if((*l).id == (*r).id) {
                    dp[id] = dp[(*l).id] + ;
                    fa[id] = (*l).id;
                }
                else if(dp[(*l).id] >= dp[(*r).id]) {
                    dp[id] = dp[(*l).id];
                    fa[id] = fa[(*l).id];
                }
                else {
                    dp[id] = dp[(*r).id];
                    fa[id] = fa[(*r).id];

                }
                g[fa[id]].pb(id);
                s.insert({id, });
                s.insert({id, });
            }
            else {
                s.erase({id, });
                s.erase({id, });
            }
        }
        dfs(, );
        if(sg[]) printf("Alice\n");
        else printf("Bob\n");
        for (int i = ; i <= n; ++i) g[i].clear(), sg[i] = fa[i] = dp[i] = ;
    }
    return ;
}