poj 1459 网络流问题`EK

Power Network

Time Limit: 2000MS		Memory Limit: 32768K
Total Submissions: 24930		Accepted: 12986

Description

A power network consists of nodes (power stations, consumers and dispatchers) connected by power transport lines. A node u may be supplied with an amount s(u) >= 0 of power, may produce an amount 0 <= p(u) <= p_max(u) of power, may consume an amount 0 <= c(u) <= min(s(u),c_max(u)) of power, and may deliver an amount d(u)=s(u)+p(u)-c(u) of power. The following restrictions apply: c(u)=0 for any power station, p(u)=0 for any consumer, and p(u)=c(u)=0 for any dispatcher. There is at most one power transport line (u,v) from a node u to a node v in the net; it transports an amount 0 <= l(u,v) <= l_max(u,v) of power delivered by u to v. Let Con=Σ_uc(u) be the power consumed in the net. The problem is to compute the maximum value of Con.


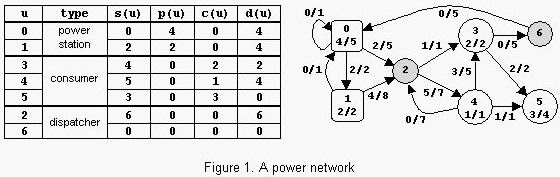
An example is in figure 1. The label x/y of power station u shows that p(u)=x and p_max(u)=y. The label x/y of consumer u shows that c(u)=x and c_max(u)=y. The label x/y of power transport line (u,v) shows that l(u,v)=x and l_max(u,v)=y.
The power consumed is Con=6. Notice that there are other possible
states of the network but the value of Con cannot exceed 6.

Input

There
are several data sets in the input. Each data set encodes a power
network. It starts with four integers: 0 <= n <= 100 (nodes), 0
<= np <= n (power stations), 0 <= nc <= n (consumers), and 0
<= m <= n^2 (power transport lines). Follow m data triplets
(u,v)z, where u and v are node identifiers (starting from 0) and 0 <=
z <= 1000 is the value of l_max(u,v). Follow np doublets (u)z, where u is the identifier of a power station and 0 <= z <= 10000 is the value of p_max(u).
The data set ends with nc doublets (u)z, where u is the identifier of a
consumer and 0 <= z <= 10000 is the value of c_max(u).
All input numbers are integers. Except the (u,v)z triplets and the (u)z
doublets, which do not contain white spaces, white spaces can occur
freely in input. Input data terminate with an end of file and are
correct.

Output

For
each data set from the input, the program prints on the standard output
the maximum amount of power that can be consumed in the corresponding
network. Each result has an integral value and is printed from the
beginning of a separate line.

Sample Input

2 1 1 2 (0,1)20 (1,0)10 (0)15 (1)20
7 2 3 13 (0,0)1 (0,1)2 (0,2)5 (1,0)1 (1,2)8 (2,3)1 (2,4)7
         (3,5)2 (3,6)5 (4,2)7 (4,3)5 (4,5)1 (6,0)5
         (0)5 (1)2 (3)2 (4)1 (5)4

Sample Output

15
6

Hint

The sample input contains two data sets. The first data set encodes a network with 2 nodes, power station 0 with pmax(0)=15 and consumer 1 with cmax(1)=20, and 2 power transport lines with lmax(0,1)=20 and lmax(1,0)=10. The maximum value of Con is 15. The second data set encodes the network from figure 1.

Source

题意：
给几个发电站，给几个消耗站，再给几个转发点。
发电站只发电，消耗站只消耗电，转发点只是转发电，再给各个传送线的传电能力。
问你消耗站能获得的最多电是多少。

思路：增加一个超级源点，和超级汇点。。把所给的发电站都和超级源点相连，把所给的消耗战都和超级汇点相连。。用EK求最大流。

#include<stdio.h>
#include<string.h>
#include<queue>
#include<iostream>
#include<algorithm>
using namespace std;
int start,end;
int edge[][];
bool vis[];
int low[],head[];
 
int bfs(){
    memset(head,-,sizeof(head));
    memset(vis,false,sizeof(vis));
    memset(low,-,sizeof(low));
    head[start]=;
    vis[start]=true;
    low[start]=0x7fffffff;
    queue<int>q;
    q.push(start) ;
    while(!q.empty()){
         int u=q.front();
         q.pop();
         for(int i=start;i<=end;i++){
            if(!vis[i]&&edge[u][i]){
                head[i]=u;
                vis[i]=true;
                low[i]=min(low[u],edge[u][i]);
                q.push(i);
            }
         }
 
    }
    if(low[end]!=-)
        return low[end];
    else
        return ;
}
 
int getmax(){
   int total=;
   int minflow;
   while(minflow=bfs()){
      for(int i=end;i!=start;i=head[i]){
          edge[head[i]][i]-=minflow;
          edge[i][head[i]]+=minflow;
 
      }
        total+=minflow;
   }
   return total;
}
 
int main(){
     int n,np,nc,m;
     while(scanf("%d%d%d%d",&n,&np,&nc,&m)!=EOF){
            int u,v,w;
        memset(edge,,sizeof(edge));
        for(int i=;i<=m;i++){
            scanf(" (%d,%d)%d",&u,&v,&w);
             edge[u+][v+]+=w;
        }
        for(int i=;i<=np;i++){
            scanf(" (%d)%d",&u,&w);
            edge[][u+]+=w;
        }
        for(int i=;i<=nc;i++){
             scanf(" (%d)%d",&v,&w);
             edge[v+][n+]+=w;
        }
        start=;
        end=n+;
        int ans=getmax();
        printf("%d\n",ans);
     }
     return ;
}