HDU 4686 Arc of Dream（递归矩阵加速）

标题效果：你就是给你一程了两个递推公式公式，第一个让你找到n结果项目。

注意需要占用该公式的复发和再构造矩阵。

Arc of Dream

Time Limit: 2000/2000 MS (Java/Others)    Memory Limit: 65535/65535 K (Java/Others)

Total Submission(s): 2092    Accepted Submission(s): 664

Problem Description

An Arc of Dream is a curve defined by following function:




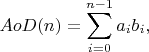
where

a₀ = A0

a_i = a_i-1*AX+AY

b₀ = B0

b_i = b_i-1*BX+BY

What is the value of AoD(N) modulo 1,000,000,007?

 

Input

There are multiple test cases. Process to the End of File.

Each test case contains 7 nonnegative integers as follows:

N

A0 AX AY

B0 BX BY

N is no more than 10¹⁸, and all the other integers are no more than 2×10⁹.

 

Output

For each test case, output AoD(N) modulo 1,000,000,007.

 

Sample Input

1
1 2 3
4 5 6
2
1 2 3
4 5 6
3
1 2 3
4 5 6

 

Sample Output

4
134
1902

 

#include <algorithm>
#include <iostream>
#include <stdlib.h>
#include <string.h>
#include <iomanip>
#include <stdio.h>
#include <string>
#include <queue>
#include <cmath>
#include <stack>
#include <map>
#include <set>
#define eps 1e-10
///#define M 1000100
#define LL __int64
///#define LL long long
///#define INF 0x7ffffff
#define INF 0x3f3f3f3f
#define PI 3.1415926535898
#define zero(x) ((fabs(x)<eps)?

0:x)

#define mod 1000000007

const int maxn = 210;

using namespace std;

struct matrix
{
    LL f[10][10];
};

matrix mul(matrix a, matrix b, int n)
{
    matrix c;
    memset(c.f, 0, sizeof(c.f));
    for(int i = 0; i < n; i++)
    {
        for(int j = 0; j < n; j++)
        {
            for(int k = 0; k < n; k++) c.f[i][j] += a.f[i][k]*b.f[k][j];
            c.f[i][j] %= mod;
        }
    }
    return c;
}

matrix pow_mod(matrix a, LL b, int n)
{
    matrix s;
    memset(s.f, 0 , sizeof(s.f));
    for(int i = 0; i < n; i++) s.f[i][i] = 1LL;
    while(b)
    {
        if(b&1) s = mul(s, a, n);
        a = mul(a, a, n);
        b >>= 1;
    }
    return s;
}

matrix Add(matrix a,matrix b, int n)
{
    matrix c;
    for(int i = 0; i < n; i++)
    {
        for(int j = 0; j < n; j++)
        {
            c.f[i][j] = a.f[i][j]+b.f[i][j];
            c.f[i][j] %= mod;
        }
    }
    return c;
}

int main()
{
    LL n;
    LL a, ax, ay;
    LL b, bx, by;
    while(~scanf("%I64d",&n))
    {
        scanf("%I64d %I64d %I64d",&a, &ax, &ay);
        scanf("%I64d %I64d %I64d",&b, &bx, &by);
        a %= mod;
        ax %= mod;
        ay %= mod;
        b %= mod;
        bx %= mod;
        by %= mod;
        LL ff = a*b%mod;
        LL x = (a*ax+ay)%mod;
        LL y = (b*bx+by)%mod;
        LL pp = (x*y)%mod;
        if(n == 0)
        {
            puts("0");
            continue;
        }
        matrix c;
        memset(c.f, 0 ,sizeof(c.f));
        c.f[0][0] = ax*bx%mod;
        c.f[0][1] = ax*by%mod;
        c.f[0][2] = ay*bx%mod;
        c.f[0][3] = ay*by%mod;
        ///c.f[0][4] = 1LL;
        c.f[1][1] = ax;
        c.f[1][3] = ay;
        c.f[2][2] = bx;
        c.f[2][3] = by;
        c.f[3][3] = 1LL;
        c.f[4][0] = 1LL;
        c.f[4][4] = 1LL;
        matrix d = pow_mod(c, n-1LL, 5);
        LL sum = 0LL;

        sum += ((d.f[4][0]*pp%mod)+(d.f[4][4]*ff%mod))%mod;
        sum += ((d.f[4][1]*x%mod) + (d.f[4][2]*y%mod) + d.f[4][3]%mod)%mod;
        printf("%I64d\n",(sum+mod)%mod);
    }
    return 0;
}

版权声明：本文博客原创文章，博客，未经同意，不得转载。