POJ1753Flip Game(DFS + 枚举)

Flip Game

Time Limit: 1000MS		Memory Limit: 65536K
Total Submissions: 37050		Accepted: 16122

Description

Flip game is played on a rectangular 4x4 field with two-sided pieces placed on each of its 16 squares. One side of each piece is white and the other one is black and each piece is lying either it's black or white side up. Each round you flip 3 to 5 pieces, thus changing the color of their upper side from black to white and vice versa. The pieces to be flipped are chosen every round according to the following rules:

1. Choose any one of the 16 pieces.
2. Flip the chosen piece and also all adjacent pieces to the left, to the right, to the top, and to the bottom of the chosen piece (if there are any).

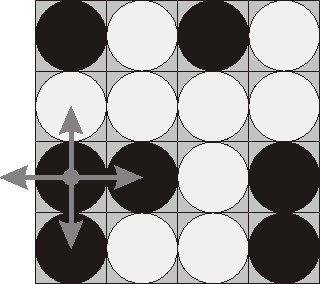Consider the following position as an example:

bwbw
wwww
bbwb
bwwb
Here "b" denotes pieces lying their black side up and "w" denotes pieces lying their white side up. If we choose to flip the 1st piece from the 3rd row (this choice is shown at the picture), then the field will become:

bwbw
bwww
wwwb
wwwb
The goal of the game is to flip either all pieces white side up or all pieces black side up. You are to write a program that will search for the minimum number of rounds needed to achieve this goal.

Input

The input consists of 4 lines with 4 characters "w" or "b" each that denote game field position.

Output

Write to the output file a single integer number - the minimum number of rounds needed to achieve the goal of the game from the given position. If the goal is initially achieved, then write 0. If it's impossible to achieve the goal, then write the word "Impossible" (without quotes).

Sample Input

bwwb
bbwb
bwwb
bwww

Sample Output

4

没点思路-_-

转载请注明出处：優YoU  http://user.qzone.qq.com/289065406/blog/1299076400

提示：翻转棋，可以建模为多叉树

本题难点有两个，一个就是不要以全黑（或全白）作为目标进行搜索，而是要把全黑（或全白）作为“根”，去搜索树叶，看看是否有 输入的棋盘状态。

另一个难点需要一点数学功底，就是要知道 树 的最大高度，这是“状态不存在”的判断标准

提示：其实每格棋子最多只可以翻转一次（实际是奇数次，但这没意义），只要其中一格重复翻了2次(不论是连续翻动还是不连翻动)，那么它以及周边的棋子和没翻动时的状态是一致的，由此就可以确定这个棋盘最多只能走16步，最多只能有翻出2^16种状态

其实也想过dfs，找不到终止状态，对，就是16就ok了，因为如果每一个状态都翻转了一遍，那么你在翻转一个牌，那么就和他之前没翻转是一样的，所以没必要了

#include <iostream>
#include <cstdio>
#include <cstring>
#include <algorithm>
using namespace std;
bool chess[][];
int step,flag;
int r[] = {-,,,};
int c[] = {,,-,};
int judge_all()
{
    for(int i = ; i <= ; i++)
    {
        for(int j = ; j <= ; j++)
        {
            if(chess[i][j] != chess[][])
                return false;
        }
    }
    return true;
}
void flip(int row, int col)
{
    chess[row][col] = !chess[row][col];
    for(int i = ; i < ; i++)
    {
        int fx = row + r[i];
        int fy = col + c[i];
        if(fx >  && fx <=  && fy >  && fy <= )
            chess[fx][fy] = !chess[fx][fy];
    }
}
void dfs(int row, int col, int deep)
{
    if(deep == step)  // 到达了翻转的指定次数，就判断是否都一样，然后返回
    {
        flag = judge_all();
        return;
    }
    if(flag || row > )
        return;
    flip(row, col);
    if(col < )
    {
        dfs(row, col + , deep + );  //如果这一行还可以翻转，就判断下一个
    }
    else
    {
        dfs(row + , , deep + );
    }

    flip(row, col);   //回溯，把原来的转过来

    if(col < )
    {
        dfs(row, col + , deep); //回溯时要判断上一步是否已经结束，因此传的是deep，判断当前状态，如果没结束，就按row,col+1变化
    }
    else
    {
        dfs(row + , , deep);
    }
    return;
}
int main()
{
    char s[];
    while(scanf("%s", s) != EOF)
    {
        memset(chess, false, sizeof(chess));
        for(int i = ; i < ; i++)
        {
            if(s[i] == 'b')
            {
                chess[][i + ] = true;
            }
        }
        for(int i = ; i <= ; i++)
        {
            scanf("%s", s);
            for(int j = ; j < ; j++)
            {
                if(s[j] == 'b')
                    chess[i][j + ] = true;
            }
        }
        flag = ;
        for(step = ; step <= ; step++)
        {
            dfs(,,);
            if(flag)
                break;
        }
        if(flag)
            printf("%d\n", step);
        else
            printf("Impossible\n");
    }

    return ;
}