POJ 2553 The Bottom of a Graph Tarjan找环缩点(题解解释输入)

Description

We will use the following (standard) definitions from graph theory. Let V be a nonempty and finite set, its elements being called vertices (or nodes). Let E be a subset of the Cartesian product V×V, its elements being called edges. Then G=(V,E) is called a directed graph.
Let n be a positive integer, and let p=(e₁,...,e_n) be a sequence of length
n of edges e_i∈E such that
e_i=(v_i,v_i+1) for a sequence of vertices
(v₁,...,v_n+1). Then
p is called a path from vertex v₁ to vertex
v_n+1 in G and we say that
v_n+1 is reachable from
v₁, writing (v₁→v_n+1).

Here are some new definitions. A node v in a graph G=(V,E) is called a sink, if for every node
w in G that is reachable from v, v is also reachable from
w. The bottom of a graph is the subset of all nodes that are sinks, i.e.,
bottom(G)={v∈V|∀w∈V:(v→w)⇒(w→v)}. You have to calculate the bottom of certain graphs.

Input

The input contains several test cases, each of which corresponds to a directed graph
G. Each test case starts with an integer number v, denoting the number of vertices of
G=(V,E), where the vertices will be identified by the integer numbers in the set
V={1,...,v}. You may assume that 1<=v<=5000. That is followed by a non-negative integer
e and, thereafter, e pairs of vertex identifiers v₁,w₁,...,v_e,w_e
with the meaning that (v_i,w_i)∈E. There are no edges other than specified by these pairs. The last test case is followed by a zero.

Output

For each test case output the bottom of the specified graph on a single line. To this end, print the numbers of all nodes that are sinks in sorted order separated by a single space character. If the bottom is empty, print an empty
line.

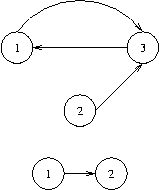
Sample Input

3 3
1 3 2 3 3 1
2 1
1 2
0

Sample Output

1 3
2

定义：点v是汇点须满足 --- 对图中任意点u，若v可以到达u则必有u到v的路径；若v不可以到达u，则u到v的路径可有可无。

题意：在n个点m条边的有向图里面，问有多少个点是汇点。
解释一下输入：分别是V顶点数，E边数，下一行每两个点是一条边的出入点。

思路：很明显的Tarjan缩点，满足题意的汇点就是缩点以后出度为0的点。

 #include<cstdio>
 #include<algorithm>
 using namespace std;
 const int maxn = 5e3+;
 int stack[maxn],dfn[maxn],low[maxn],head[maxn],dfs_num,top;
 int color[maxn],col_num,in[maxn],out[maxn],mm[maxn],a[maxn];
 bool vis[maxn];
 class edge
 {
 public:
     int to,next;
 }e[maxn*maxn];
 inline int gmin(int a,int b)
 {
     return a<b?a:b;
 }
 void Tarjan ( int x ) {
          dfn[ x ] = ++dfs_num ;
          low[ x ] = dfs_num ;
          vis [ x ] = true ;//是否在栈中
          stack [ ++top ] = x ;
          for ( int i=head[ x ] ; i!= ; i=e[i].next ){
                   int temp = e[ i ].to ;
                   if ( !dfn[ temp ] ){
                            Tarjan ( temp ) ;
                            low[ x ] = gmin ( low[ x ] , low[ temp ] ) ;
                  }
                  else if ( vis[ temp ])low[ x ] = gmin ( low[ x ] , dfn[ temp ] ) ;
          }
          if ( dfn[ x ]==low[ x ] ) {//构成强连通分量
                   vis[ x ] = false ;
                   color[ x ] = ++col_num ;//染色
                   while ( stack[ top ] != x ) {//清空
                            color [stack[ top ]] = col_num ;
                            vis [ stack[ top-- ] ] = false ;
                  }
                  top -- ;
          }
 }

 int main()
 {
     int n,m;
     while(scanf("%d",&n)){
     if(!n)break;
     scanf("%d",&m);
     col_num=dfs_num=top=;
     for(int i=;i<=n;i++)
         head[i]=in[i]=out[i]=dfn[i]=;
     for(int i=;i<=m;i++)
     {
         int x,y;
         scanf("%d%d",&x,&y);
         e[i].next=head[x];
         e[i].to=y;
         head[x]=i;
     }
     for(int i=;i<=n;i++)if(!dfn[i])Tarjan(i);
     for(int i=;i<=n;i++)
     {
         for(int j=head[i];j;j=e[j].next)
         {
             int t=e[j].to;
             if(color[i]!=color[t])
             {
                 out[color[i]]++;
                 in[color[t]]++;
             }
         }
     }
     int k=,ans=;
     for(int i=;i<=col_num;i++)
         if(!out[i])mm[++k]=i;
     for(int i=;i<=k;i++)
         for(int j=;j<=n;j++)
         if(mm[i]==color[j])a[++ans]=j;
     sort(a+,a+ans+);
     //printf("%d %d %d\n",k,ans,col_num);忘记初始化 debug多组样例一直过不了而加的..
     for(int i=;i<=ans;i++)
         printf("%d%c",a[i],i!=ans?' ':'\n');
     }
     return ;
 }