POJ 1286 Pólya定理
| Time Limit: 1000MS | Memory Limit: 10000K | |
| Total Submissions: 9162 | Accepted: 3786 |
Description
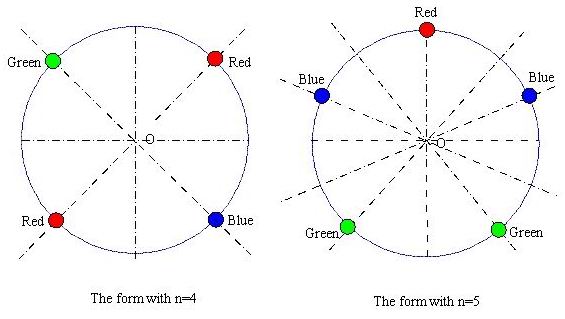
Input
-1 denotes the end of the input file.
Output
Sample Input
4
5
-1
Sample Output
21
39
题意:n个珠子串成一个圆,用三种颜色去涂色,问一共有多少种不同的涂色方法(不同的涂色方法被定义为:如果这种涂色情况翻转,旋转不与其他情况相同就为不同。)
思路:这道题其实就是一个最简单的板子题。要想明白Polya定理首先要知道置换,置换群和轮换的概念,可以参考这里(用例子很好理解)。
项链可以进行旋转和翻转。
翻转:如果n是奇数,则存在n中置换,每种置换包含n/2+1种循环(即轮换)。
如果n是偶数,如果对称轴过顶点,则存在n/2种置换,每种置换包含n/2种循环(即轮换)
如果对称轴不过顶点,则存在n/2种置换,每种置换包含n/2+1种循环(即轮换)
旋转:n个点顺时针或者逆时针旋转i个位置的置换,轮换个数为gcd(n,i)
代码:
#include"bits/stdc++.h"
#define db double
#define ll long long
#define vec vector<ll>
#define mt vector<vec>
#define ci(x) scanf("%d",&x)
#define cd(x) scanf("%lf",&x)
#define cl(x) scanf("%lld",&x)
#define pi(x) printf("%d\n",x)
#define pd(x) printf("%f\n",x)
#define pl(x) printf("%lld\n",x)
//#define rep(i, x, y) for(int i=x;i<=y;i++)
#define rep(i, n) for(int i=0;i<n;i++)
const int N = 1e3+ ;
const int mod = 1e9 + ;
//const int MOD = mod - 1;
const int inf = 0x3f3f3f3f;
const db PI = acos(-1.0);
const db eps = 1e-;
using namespace std;
ll gcd(ll x,ll y){
return y==?x:gcd(y,x%y);
}
ll qpow(ll x,ll n)
{
ll ans=;
x%=mod;
while(n){
if(n&) ans=ans*x;
x=x*x;
n>>=;
}
return ans;
} int main()
{
ll n;
while(cin>>n&&n!=-)
{
if(!n) puts("");
else
{
ll ans=;
for(ll i=;i<=n;i++) ans+=qpow(3ll,gcd(n,i));
if(n&) ans+=qpow(3ll,n/+)*n;
else ans+=qpow(3ll,n/+)*(n/)+qpow(3ll,n/)*(n/);
pl(ans//n);
}
}
return ;
}
POJ 1286 Pólya定理的更多相关文章
- poj 1286 polya定理
Necklace of Beads Description Beads of red, blue or green colors are connected together into a circu ...
- poj 2409(polya定理模板)
题意:给你n种颜色和m个小球,问你有多少种不同的方案! 分析:作为模板.. 代码实现: #include <iostream> #include <cstdio> #inclu ...
- poj 1286 Necklace of Beads & poj 2409 Let it Bead(初涉polya定理)
http://poj.org/problem?id=1286 题意:有红.绿.蓝三种颜色的n个珠子.要把它们构成一个项链,问有多少种不同的方法.旋转和翻转后同样的属于同一种方法. polya计数. 搜 ...
- POJ 1286 Necklace of Beads(Polya定理)
点我看题目 题意 :给你3个颜色的n个珠子,能组成多少不同形式的项链. 思路 :这个题分类就是polya定理,这个定理看起来真的是很麻烦啊T_T.......看了有个人写的不错: Polya定理: ( ...
- POJ 2409 Let it Bead(Polya定理)
点我看题目 题意 :给你c种颜色的n个珠子,问你可以组成多少种形式. 思路 :polya定理的应用,与1286差不多一样,代码一改就可以交....POJ 1286题解 #include <std ...
- POJ 2409 Let it Bead:置换群 Polya定理
题目链接:http://poj.org/problem?id=2409 题意: 有一串n个珠子穿起来的项链,你有k种颜色来给每一个珠子染色. 问你染色后有多少种不同的项链. 注:“不同”的概念是指无论 ...
- POJ 1286 【POLYA】
题意: 给你三种颜色的珠子,每次给你N,问在旋转,翻转之后视作相同的情况下,能组成多少种不同的项链. 思路: 让我们借这道题拯救一下我对POLYA定理的理解... sigma(m^(gcd(i,n)) ...
- poj 1286 Necklace of Beads (polya(旋转+翻转)+模板)
Description Beads of red, blue or green colors are connected together into a circular necklace of ...
- POJ 2409 Let it Bead (Polya定理)
题意 用k种颜色对n个珠子构成的环上色,旋转翻转后相同的只算一种,求不等价的着色方案数. 思路 Polya定理 X是对象集合{1, 2, --, n}, 设G是X上的置换群,用M种颜色染N种对象,则不 ...
随机推荐
- 处理http请求时,如何处理url的参数
1.@PathVariable 获取url中的数据 这种写法显得简洁 也可以把参数写在前面 @RequestParam 获取请求参数的值 适合传统的get提交参数的获取 给参数id设置一个默认值 不传 ...
- BZOJ4653: [Noi2016]区间(线段树 双指针)
题意 题目链接 Sol 按照dls的说法,一般这一类的题有两种思路,一种是枚举一个点\(M\),然后check它能否成为答案.但是对于此题来说好像不好搞 另一种思路是枚举最小的区间长度是多少,这样我们 ...
- HTML:::before和::after伪元素的用法
随笔 - 366 文章 - 0 评论 - 392 ::before和::after伪元素的用法 一.介绍 css3为了区分伪类和伪元素,伪元素采用双冒号写法. 常见伪类——:hover,:li ...
- 【转载】每天一个Linux命令
目 录 每天一个linux命令(1) : ls 命令 每天一个linux命令(2) : cd 命令 每天一个linux命令(3) : pwd 命令 每天一个linux命令(4) : mkdi ...
- 通过CSS3实现:鼠标悬停图片360度旋转效果
效果很好玩,代码很简单: 效果: 鼠标放置在图片上:360度顺时针旋转 鼠标离开图片:图片260度逆时针旋转 只要将下面代码拷贝过去,并将图片改成你想要的就可以看到效果 <!doctype ht ...
- struts2表单提交Date数据无法接收
问题:在Struts2环境下,提交含有Date类型数据表单,但是在action中没有接收到:String就可以直接接收到: --网络搜索后,说Struts2可以自己转,但是目前没发现有: 然后在狂搜, ...
- mac 下使用 ikbcG87 及使用 karabiner 改大小写键
前言 一直使用 mac ,对于机械键盘还处于刚入坑阶段,作为小白还是选择能够兼容 mac 的机械键盘,于是选择了 ikbcG87 "双子座" 1.使用 ikbc G87 mac 模 ...
- springmvc--jsp页面乱码
最近想做一个平台,花时间学习springmvc.现在把零散的问题做总结 A页面写数据,一个post请求,B页面负责数据接收和显示,但是在B页面中输入中文确乱码了 A页面如下 B页面展示如下 B页面的j ...
- 使用extentreports美化报告
无意之间在整理testng 报告输出的文档时,发现一个美化testng的报告的插件,感觉确实“漂亮”,但是还不确定是否实用,案例来自官方网站自己添了一些内容,更改了存放路径,本地目前已确定可正常运行, ...
- PHPmailer群发Gmail的常见问题
博主小白一枚,phpmailer只会一些基本的用法,就这样一个邮件的群发功能也难住了我一周,下面把我遇到的问题给大家总结一下 1.Could not authenticate 首先,如果你没有使用循环 ...
