Radar Installation 贪心

Language:
Default

Radar Installation

Time Limit: 1000MS		Memory Limit: 10000K
Total Submissions: 42461		Accepted: 9409

Description

Assume the coasting is an infinite straight line. Land is in one side of coasting, sea in the other. Each small island is a point locating in the sea side. And any radar installation, locating on the coasting, can only cover d distance, so an island in the sea can be covered by a radius installation, if the distance between them is at most d.

We use Cartesian coordinate system, defining the coasting is the x-axis. The sea side is above x-axis, and the land side below. Given the position of each island in the sea, and given the distance of the coverage of the radar installation, your task is to write a program to find the minimal number of radar installations to cover all the islands. Note that the position of an island is represented by its x-y coordinates.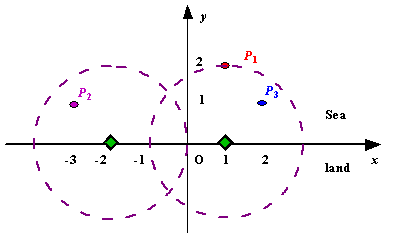 
 
Figure A Sample Input of Radar Installations

Input

The input consists of several test cases. The first line of each case contains two integers n (1<=n<=1000) and d, where n is the number of islands in the sea and d is the distance of coverage of the radar installation. This is followed by n lines each containing two integers representing the coordinate of the position of each island. Then a blank line follows to separate the cases.

The input is terminated by a line containing pair of zeros

Output

For each test case output one line consisting of the test case number followed by the minimal number of radar installations needed. "-1" installation means no solution for that case.

Sample Input

3 2
1 2
-3 1
2 1

1 2
0 2

0 0

Sample Output

Case 1: 2
Case 2: 1

 #include<stdio.h>
 #include<string.h>
 #include<math.h>
 #include<algorithm>
 #include<iostream>
 #include<stack>
 #include<math.h>
 using namespace std;

 struct node
 {
     double start;
     double end;
 }coor[];//记录每个区间的端点
 int cmp(const struct node a,const struct node b)
 {
     return a.start < b.start;
 }
 int t,r;
 stack <node> st;//用栈存每个区间，

 int cal(int ans)
 {
     while(!st.empty())
         st.pop();
     for(int i = t-; i >= ; i--)
         st.push(coor[i]);
     while(st.size() >= )//当栈中至少存在两个区间时
     {
         struct node tmp1 = st.top();
         st.pop();
         struct node tmp2 = st.top();
         if(tmp1.end >= tmp2.start)//当取出的两个区间有公共部分时
         {
             st.pop();//tmp2出栈
             struct node tmp;
             tmp.start = max(tmp1.start, tmp2.start);//注意取公共部分时，起始点取较大者
             tmp.end = min(tmp1.end, tmp2.end);//终点取较小者
             st.push(tmp);//将公共部分入栈
             ans--;//每两个区间交一次，雷达个数减一次
         }
     }
     return ans;
 }
 int main()
 {

     int cor_x[],cor_y[];
     double add;
     int cnt = ;
     while(~scanf("%d %d",&t,&r))
     {
         int ok = ;//判断小岛的坐标是否合法，
         if(t ==  && r == ) break;
         for(int i = ; i < t; i++)
         {
             scanf("%d %d",&cor_x[i],&cor_y[i]);
             if(cor_y[i] > r)//若小岛纵坐标大于半径则不合法
                 ok = ;
         }
         if(ok == )
         {
             printf("Case %d: -1\n", cnt++);
             continue;
         }
         for(int i = ; i < t; i++)
         {
             //以每个小岛为圆心，r为半径画圆，coor[]存该圆与x轴相交的区间
             add = sqrt(r*r-cor_y[i]*cor_y[i]);
             coor[i].start = cor_x[i] - add;
             coor[i].end = cor_x[i] + add;
         }
         sort(coor,coor+t,cmp);//对这些区间按起始点从小到大排序
         int ans = t;
         ans = cal(ans);
         printf("Case %d: %d\n",cnt++,ans);
     }
     return ;
 }