[LeetCode] 119. Pascal's Triangle II 杨辉三角之二
Given a non-negative index k where k ≤ 33, return the kth index row of the Pascal's triangle.
Note that the row index starts from 0.

In Pascal's triangle, each number is the sum of the two numbers directly above it.
Example:
Input: 3
Output: [1,3,3,1]
Follow up:
Could you optimize your algorithm to use only O(k) extra space?
杨辉三角想必大家并不陌生,应该最早出现在初高中的数学中,其实就是二项式系数的一种写法。
1
1 1
1 2 1
1 3 3 1
1 4 6 4 1
1 5 10 10 5 1
1 6 15 20 15 6 1
1 7 21 35 35 21 7 1
1 8 28 56 70 56 28 8 1
杨辉三角形第n层(顶层称第0层,第1行,第n层即第 n+1 行,此处n为包含0在内的自然数)正好对应于二项式 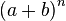 展开的系数。例如第二层 1 2 1 是幂指数为2的二项式
展开的系数。例如第二层 1 2 1 是幂指数为2的二项式 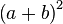 展开形式
展开形式 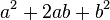 的系数。
的系数。
杨辉三角主要有下列五条性质:
- 杨辉三角以正整数构成,数字左右对称,每行由1开始逐渐变大,然后变小,回到1。
- 第
 行的数字个数为
行的数字个数为 个。
个。 - 第
 行的第
行的第 个数字为组合数
个数字为组合数 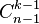 。
。 - 第
 行数字和为
行数字和为 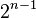 。
。 - 除每行最左侧与最右侧的数字以外,每个数字等于它的左上方与右上方两个数字之和(也就是说,第
 行第
行第 个数字等于第
个数字等于第 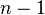 行的第
行的第 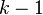 个数字与第
个数字与第 个数字的和)。这是因为有组合恒等式:
个数字的和)。这是因为有组合恒等式: 。可用此性质写出整个杨辉三角形。
。可用此性质写出整个杨辉三角形。
由于题目有额外限制条件,程序只能使用 O(k) 的额外空间,那么这样就不能把每行都算出来,而是要用其他的方法, 我最先考虑用的是第三条性质,算出每个组合数来生成第n行系数,代码请参见评论区一楼。本地调试输出前十行,没啥问题,拿到 OJ 上测试,程序在第 18 行跪了,中间有个系数不正确。那么问题出在哪了呢,仔细找找,原来出在计算组合数那里,由于算组合数时需要算连乘,而整型数 int 的数值范围只有 -32768 到 32768 之间,那么一旦n值过大,连乘肯定无法计算。而丧心病狂的 OJ 肯定会测试到成百上千行,所以这个方法不行。那么我们再来考虑利用第五条性质,除了第一个和最后一个数字之外,其他的数字都是上一行左右两个值之和。那么我们只需要两个 for 循环,除了第一个数为1之外,后面的数都是上一次循环的数值加上它前面位置的数值之和,不停地更新每一个位置的值,便可以得到第n行的数字,具体实现代码如下:
class Solution {
public:
vector<int> getRow(int rowIndex) {
vector<int> res(rowIndex + );
res[] = ;
for (int i = ; i <= rowIndex; ++i) {
for (int j = i; j >= ; --j) {
res[j] += res[j - ];
}
}
return res;
}
};
Github 同步地址:
https://github.com/grandyang/leetcode/issues/119
类似题目:
参考资料:
https://leetcode.com/problems/pascals-triangle-ii/
https://leetcode.com/problems/pascals-triangle-ii/discuss/38420/Here-is-my-brief-O(k)-solution
LeetCode All in One 题目讲解汇总(持续更新中...)
[LeetCode] 119. Pascal's Triangle II 杨辉三角之二的更多相关文章
- [LeetCode] 119. Pascal's Triangle II 杨辉三角 II
Given an index k, return the kth row of the Pascal's triangle. For example, given k = 3,Return [1,3, ...
- [LeetCode] Pascal's Triangle II 杨辉三角之二
Given an index k, return the kth row of the Pascal's triangle. For example, given k = 3,Return [1,3, ...
- Leetcode#118. Pascal's Triangle(杨辉三角)
题目描述 给定一个非负整数 numRows,生成杨辉三角的前 numRows 行. 在杨辉三角中,每个数是它左上方和右上方的数的和. 示例: 输入: 5 输出: [ [1], [1,1], [1,2, ...
- LeetCode 118. Pascal's Triangle (杨辉三角)
Given numRows, generate the first numRows of Pascal's triangle. For example, given numRows = 5,Retur ...
- LeetCode 119 Pascal's Triangle II
Problem: Given an index k, return the kth row of the Pascal's triangle. For example, given k = 3,Ret ...
- Leetcode 119 Pascal's Triangle II 数论递推
杨辉三角,这次要输出第rowIndex行 用滚动数组t进行递推 t[(i+1)%2][j] = t[i%2][j] + t[i%2][j - 1]; class Solution { public: ...
- LeetCode 119. Pascal's Triangle II (杨辉三角之二)
Given an index k, return the kth row of the Pascal's triangle. For example, given k = 3,Return [1,3, ...
- leetcode 119 Pascal's Triangle II ----- java
Given an index k, return the kth row of the Pascal's triangle. For example, given k = 3,Return [1,3, ...
- Java [Leetcode 119]Pascal's Triangle II
题目描述: Given an index k, return the kth row of the Pascal's triangle. For example, given k = 3,Return ...
随机推荐
- 三、ForkJoin分析
ForkJoin分析 一.ForkJoin ForkJoin是由JDK1.7后提供多线并发处理框架.ForkJoin的框架的基本思想是分而治之.什么是分而治之?分而治之就是将一个复杂的计算,按照设 ...
- (转)dnSpy 强大的.Net反编译软件
目录 1. Debug外部引用的Dll文件2. 调试应用程序3. 修改exe文件的内容 作者:D.泡沫 一说起.net的反编译软件,大家首先想到的就是Reflector,ILSpy,dotPeek等等 ...
- JSP是Servlet详解
前言:前一段时间写了好多Servlet和JSP相关的博客,自以为理解的差不多了,岂不知人外有人,天外有天,代码外还有源码,受高人点拨,看了一下Servlet源码,感触颇深,再也不敢说懂了,不明白生活的 ...
- CSS教程详解
CSS学习笔记 一.CSS基础 1.CSS简介 层叠:一层一层的: 样式表:很多的属性和样式 CSS语法: <style> 选择器 { 属性名:属性值; 属性名:属性值; …… } &l ...
- SetApartmentState(ApartmentState state).Ensure that your Main function has STAThreadAttribute marked on it. This exception is only raised if a debugger is attached to the process
System.Threading.ThreadStateException: 'Current thread must be set to single thread apartment (STA) ...
- linux内核级同步机制--futex
在面试中关于多线程同步,你必须要思考的问题 一文中,我们知道glibc的pthread_cond_timedwait底层是用linux futex机制实现的. 理想的同步机制应该是没有锁冲突时在用户态 ...
- 最新版windows安装支持输入shell命令的工具cygwin教程
首先去官网下载自己对应系统32位或64位系统版本安装包:https://cygwin.com/install.html 下载好后按提示一步一步安装,直到这一步: 初次安装,这里是空的,没有下载的镜像链 ...
- ANDROID培训准备资料之项目结构简单介绍
Android Studio项目结构初步主要介绍下面几个文件夹,后续再补充 (1)java文件夹的介绍 (2)Res文件夹的介绍 (3)R文件的介绍 (4)Manifests文件夹的介绍 我们先看看整 ...
- MySQL的select(极客时间学习笔记)
查询语句 首先, 准备数据, 地址是: https://github.com/cystanford/sql_heros_data, 除了id以外, 24个字段的含义如下: 查询 查询分为单列查询, 多 ...
- spring的组件使用
源代码下载:https://www.lanzous.com/i5p4mvc * 组件扫描 * @Component:表示这个类需要在应用程序中被创建 * @ComponentScan:自动发现应用程序 ...
