POJ3087:Shuffle'm Up(模拟）

http://poj.org/problem?id=3087

Description

A common pastime for poker players at a poker table is to shuffle stacks of chips. Shuffling chips is performed by starting with two stacks of poker chips, S₁ and S₂, each stack containing C chips. Each stack may contain chips of several different colors.

The actual shuffle operation is performed by interleaving a chip from S₁ with a chip from S₂ as shown below for C = 5:

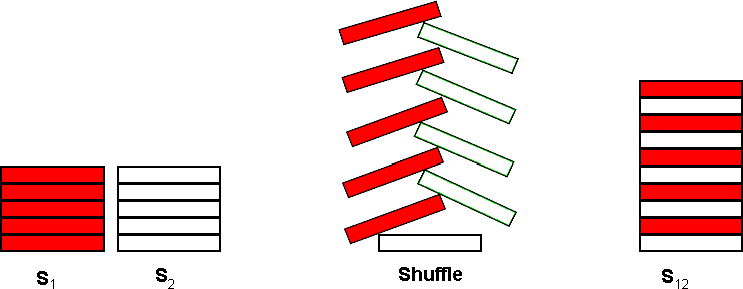
The single resultant stack, S₁₂, contains 2 * C chips. The bottommost chip of S₁₂ is the bottommost chip from S₂. On top of that chip, is the bottommost chip from S₁. The interleaving process continues taking the 2^nd chip from the bottom of S₂ and placing that on S₁₂, followed by the 2^nd chip from the bottom of S₁ and so on until the topmost chip from S₁ is placed on top of S₁₂.

After the shuffle operation, S₁₂ is split into 2 new stacks by taking the bottommost C chips from S₁₂ to form a new S₁ and the topmost C chips from S₁₂ to form a new S₂. The shuffle operation may then be repeated to form a new S₁₂.

For this problem, you will write a program to determine if a particular resultant stack S₁₂ can be formed by shuffling two stacks some number of times.

Input

The first line of input contains a single integer N, (1 ≤ N ≤ 1000) which is the number of datasets that follow.

Each dataset consists of four lines of input. The first line of a dataset specifies an integer C, (1 ≤ C ≤ 100) which is the number of chips in each initial stack (S₁ and S₂). The second line of each dataset specifies the colors of each of the C chips in stack S₁, starting with the bottommost chip. The third line of each dataset specifies the colors of each of the C chips in stack S₂ starting with the bottommost chip. Colors are expressed as a single uppercase letter (A through H). There are no blanks or separators between the chip colors. The fourth line of each dataset contains 2 * C uppercase letters (A through H), representing the colors of the desired result of the shuffling of S₁ and S₂ zero or more times. The bottommost chip’s color is specified first.

Output

Output for each dataset consists of a single line that displays the dataset number (1 though N), a space, and an integer value which is the minimum number of shuffle operations required to get the desired resultant stack. If the desired result can not be reached using the input for the dataset, display the value negative 1 (−1) for the number of shuffle operations.

Sample Input

2
4
AHAH
HAHA
HHAAAAHH
3
CDE
CDE
EEDDCC

Sample Output

1 2
2 -1

题目大意：

已知两堆牌s1和s2的初始状态， 其牌数均为c，按给定规则能将他们相互交叉组合成一堆牌s12，再将s12的最

底下的c块牌归为s1，最顶的c块牌归为s2，依此循环下去。现在输入s1和s2的初始状态 以及 预想的最终状态

s12问s1 s2经过多少次洗牌之后，最终能达到状态s12，若永远不可能相同，则输出"-1"。

题目解析：
水题一道却放在bfs训练计划中，害我不敢做。最主要的部分就是状态记录，然后判重。

若s1和s2在洗牌后的状态，是前面洗牌时已经出现过的一个状态，且这个状态不是预想的状态S12，就说明无论怎样再洗牌都不可能达到S12了，因为这个洗牌操作已经陷入了一个“环”。

如果状态没有重复过，则一直模拟洗牌，直至s12出现。

记录状态可以用map<string,int>q。

Map的缺省值为0

#include <iostream>
#include <string.h>
#include <stdlib.h>
#include <stdio.h>
#include <map>
using namespace std;
int n;
int main()
{
    int T,sum,K=;
    scanf("%d",&T);
    char s1[],s2[],s12[];
    char d[];
    while(T--)
    {
        ++K;
        sum=;
        scanf("%d",&n);
        scanf("%*c%s%s%s",s1,s2,s12);
        map<string,int>q;
        q[s12]=;
        while()
        {
            int z=;
            for(int i=; i<n; i++)
            {
                d[z++]=s2[i];
                d[z++]=s1[i];
            }
            d[z]='\0';
            sum++;
            if(strcmp(d,s12)==)
            {
                printf("%d %d\n",K,sum);
                break;
            }
            else if(q[d]!=)
            {
                printf("%d -1\n",K);
                break;
            }
            q[d]=;
            for(int i=; i<n; i++)
            {
                s1[i]=d[i];
            }
            s1[n]='\0';
            for(int i=,j=n; j<z; j++,i++)
                s2[i]=d[j];
            s2[n]='\0';
        }
    }
    return ;
}