【HDU 5572 An Easy Physics Problem】计算几何基础

2015上海区域赛现场赛第5题。

题目连接：http://acm.hdu.edu.cn/showproblem.php?pid=5572

题意：在平面上，已知圆(O, R)，点B、A（均在圆外），向量V。

一个小球从A出发，沿速度向量V匀速前进，若撞到圆则发生弹性碰撞，沿“反射方向”继续匀速前进。问A点能否经过B点。

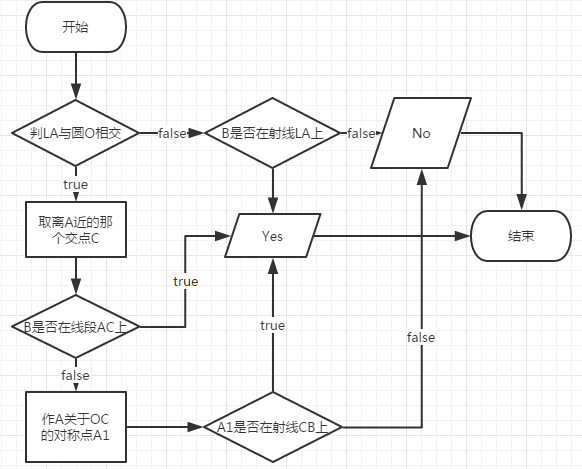
题目读懂了，把所有情况都考虑全，流程图就出来了，然后直接套模版即可（注意有些功能可剪裁~）

我的第一道计算几何，WA了一个星期啊。。。原因有姿势不对，精度不对，还有输出不对的。

不过借此积累了入门的一些模版，向量运算等等，见代码。

下面这个版本可以说是参考网上群巨的代码拼凑着写出的，不过期间发现OJ上的数据没有覆盖所有情况，导致大家各种姿势过的都有，看得云里雾里。

之后自己一遍遍整理思路，考虑各种情况，把别人的代码自己不太赞同的地方强行改成了自己的思路，于是有了下面这个AC的代码。

 #include <cstdio>
 #include <cmath>
 #include <algorithm>
 using namespace std;

 const double eps = 1e-;

 int cmp(double x){
     return x < -eps ? - : (x>eps);
 }

 double pow2(double x){
     return x * x;
 }

 double mySqrt(double x){
     return sqrt(max((double), x));
 }

 struct Vec
 {
     double x, y;
     Vec(){}
     Vec(double xx, double yy):x(xx), y(yy){}

     Vec& operator=(const Vec& v){
         x = v.x;
         y = v.y;
         return *this;
     }

     double norm(){
         return sqrt(pow2(x) + pow2(y));
     }
     //返回单位向量
     Vec unit(){
         return Vec(x, y) / norm();
     }
     //判等
     friend bool operator==(const Vec& v1, const Vec& v2){
         return cmp(v1.x - v2.x)== && cmp(v1.y - v2.y)==;
     }

     //+, -, 数乘
     friend Vec operator+(const Vec& v1, const Vec& v2){
         return Vec(v1.x + v2.x, v1.y + v2.y);
     }
     friend Vec operator-(const Vec& v1, const Vec& v2){
         return Vec(v1.x - v2.x, v1.y - v2.y);
     }
     friend Vec operator*(const Vec& v, const double c){
         return Vec(c*v.x, c*v.y);
     }
     friend Vec operator*(const double c, const Vec& v){
         return Vec(c*v.x, c*v.y);
     }
     friend Vec operator/(const Vec& v, const double c){
         return Vec(v.x/c, v.y/c);
     }
 };

 int T;
 int ans;
 Vec O, A, V, B, C, B1;
 int R;

 //点乘
 double dot(const Vec v1, const Vec v2){
     return v1.x*v2.x + v1.y*v2.y;
 }
 //叉乘的模长
 double product(const Vec v1, const Vec v2){
     return v1.x*v2.y - v1.y*v2.x;
 }

 //点p到直线v1,v2的投影
 Vec project(Vec& v1, Vec& v2, Vec& p){
     Vec v = v2 - v1;
     return v1 + v * dot(v, p-v1) / dot(v, v);
 }
 //点p关于直线v1,v2的对称点
 Vec mirrorPoint(Vec& v1, Vec& v2, Vec& p){
     Vec c = project(v1, v2, p);
     //printf("project: %lf, %lf\n", c.x, c.y);
     return (double)*c - p;
 }

 //判断点p是否在线段v1,v2上
 bool onSeg(Vec& v1, Vec& v2, Vec& p){
     if(cmp(product(p-v1, v2-v1))== && cmp(dot(p-v1, p-v2))<=)
         return true;
     return false;
 }

 bool calc_C(){
     //将AC表示为关于t的参数方程
     //则与圆的方程联立得到关于t的一元二次方程, a,b,c为一般式的三个系数
     double a = pow2(V.x) + pow2(V.y);
     double b = *V.x*(A.x - O.x) + *V.y*(A.y - O.y);
     double c = pow2(A.x - O.x) + pow2(A.y - O.y) - pow2(R);
     double delta = pow2(b) - *a*c;
     if(cmp(delta) <= ) return false;
     else{
         double t1 = (-b - mySqrt(delta))/(*a);
         double t2 = (-b + mySqrt(delta))/(*a);
         double t;
         if(cmp(t1) >= ) t = t1;
         if(cmp(t2) >=  && t2 < t1) t = t2;//取较小的那个，即为离A近的那个交点
         C.x = A.x + V.x*t;
         C.y = A.y + V.y*t;
         return true; //有交点
     }
 }

 int main()
 {
     freopen("5572.txt", "r", stdin);
     scanf("%d", &T);
     for(int ca = ; ca <= T; ca++){
         scanf("%lf%lf%d", &O.x, &O.y, &R);
         scanf("%lf%lf%lf%lf", &A.x, &A.y, &V.x, &V.y);
         scanf("%lf%lf", &B.x, &B.y);
         if(calc_C()){
             if(onSeg(A, C, B)) ans = ;
             else{
                 //printf("has intersection (%lf, %lf)\n", C.x, C.y);
                 Vec A1 = mirrorPoint(O, C, A);
                   // printf("A: %lf, %lf\n", A.x, A.y);
                   // printf("A1: %lf, %lf\n", A1.x, A1.y);
                 //printf("B1 (%lf, %lf)\n", B1.x, B1.y);
                 if(A==A1){
                     Vec u = B - O;
                     Vec v = C - O;
                     // printf("OB: %lf %lf\n", u.unit().x, u.unit().y);
                     // printf("OC: %lf %lf\n", v.unit().x, v.unit().y);
                     if(u.unit() == v.unit()){
                         ans = ;
                     }else ans = ;
                 }else {
                     Vec u = B - C;
                     Vec v = A1 - C;
                     if(u.unit() == v.unit()){
                         ans = ;
                     }
                     else ans = ;
                 }
             }
         }else{//运动方向不变，则AB与V同向才可碰到B
             //printf("no intersection\n");
             Vec temp = B - A;
             if(temp.unit() == V.unit())
                 ans = ;
             else ans = ;
         }
         printf("Case #%d: %s\n", ca, ans ? "Yes" : "No");
     }
     return ;
 }

ver1

然后呢，意识到自己实在太弱了，之前的尝试好比盲人摸象，该找些系统的资料好好学一学，于是学习了《训练指南》白书的计算几何入门部分，作者的讲解深入浅出，代码规范清晰且经得起推敲，真是初学者的福音。（即使一些代码没给出，也都可以利用高中学过的知识推出）。于是然后就有了下面这个版本，完全按照上面流程图的思路实现。

这个版本开始一直WA，不明原因，开始比对上一版本做修改，最后发现把eps由1e-10改成1e-8就过了。几何题精度真是个问题，应该需要试才能知道。

 //按照训练指南白书上模版写的新姿势，更好理解
 #include <cstdio>
 #include <vector>
 #include <cmath>
 using namespace std;

 const double eps = 1e-; //1e-10会WA，注意调整精度，过大过小都不行
 int dcmp(double x){
     if(fabs(x) < eps) return ;
     return x <  ? - : ;
 }
 double mySqrt(double x){
     return sqrt(max((double), x));
 }

 struct Point
 {
     double x, y;
     Point(double x=, double y=):x(x), y(y){}
     Point& operator = (Point p){
         x = p.x;
         y = p.y;
         return *this;
     }
 };

 typedef Point Vector;

 Vector operator + (Vector A, Vector B){ return Vector(A.x + B.x, A.y + B.y);}
 Vector operator - (Point A, Point B){ return Vector(A.x - B.x, A.y - B.y);}
 Vector operator * (Vector A, double p){ return Vector(A.x * p, A.y * p);}
 Vector operator / (Vector A, double p){ return Vector(A.x / p, A.y / p);}

 double dot(Vector A, Vector B){ return A.x * B.x + A.y * B.y;}
 double length(Vector A){ return mySqrt(dot(A, A));}
 double cross(Vector A, Vector B){ return A.x * B.y - A.y * B.x;}

 struct Line
 {
     Point p;
     Vector v;
     Line(Point p, Vector v):p(p), v(v){}
     Point getPoint(double t){
         return Point(p.x + v.x*t, p.y + v.y*t);
     }
 };

 struct Circle
 {
     Point c;
     double r;
     Circle(Point c, double r):c(c), r(r){}
 };

 int getLineCircleIntersection(Line L, Circle C, Point& P){ //返回t较小的那个点
     double a = L.v.x;
     double b = L.p.x - C.c.x;
     double c = L.v.y;
     double d = L.p.y - C.c.y;

     double e = a*a + c*c;
     double f = *(a*b + c*d);
     double g = b*b + d*d - C.r*C.r;

     double delta = f*f - *e*g;

     if(dcmp(delta) <= ) return ;

     double t = (-f - mySqrt(delta)) / (*e);
     P = L.getPoint(t);
     return ;
 }

 bool onRay(Point A, Line L){//点A在射线L(p,v)上，不含端点
     Vector w = A - L.p;
     if(dcmp(cross(w, L.v))== && dcmp(dot(w, L.v))>) return true;
     return false;
 }

 bool onSeg(Point A, Point B, Point C){//点A在线段BC上
     return dcmp(cross(B-A, C-A))== && dcmp(dot(B-A, C-A))<;
 }

 Point project(Point A, Line L){
     return L.p + L.v * (dot(L.v, A - L.p)/dot(L.v, L.v));
 }
 Point mirrorPoint(Point A, Line L){
     Vector D = project(A, L);
     //printf("project: %lf, %lf\n", D.x, D.y);
     return D + (D - A);
 }

 int main()
 {
     int T;
     int ans = ;
     double R;
     Point O, A, B;
     Vector V;
     freopen("5572.txt", "r", stdin);
     scanf("%d", &T);
     for(int ca = ; ca <= T; ca++){
         scanf("%lf%lf%lf", &O.x, &O.y, &R);
         scanf("%lf%lf%lf%lf", &A.x, &A.y, &V.x, &V.y);
         scanf("%lf%lf", &B.x, &B.y);
         Line LA = Line(A, V);
         Circle yuanO = Circle(O, R);
         Point C;
         if(getLineCircleIntersection(LA, yuanO, C)){
             if(onSeg(B, A, C)) ans = ;
             else{
                 Line OC = Line(O, Vector(C.x - O.x, C.y - O.y));
                 Point A1 = mirrorPoint(A, OC);
                 // printf("%lf, %lf\n", C.x, C.y);
                 // printf("%lf, %lf\n", A1.x, A1.y);
                 Line CB = Line(C, Vector(B.x - C.x, B.y - C.y));

                 if(onRay(A1, CB)){
                      ans = ;

                 }
                 else ans = ;
             }
         }else{
             if(onRay(B, LA)) ans = ;
             else ans = ;
         }
         printf("Case #%d: %s\n", ca, ans ? "Yes" : "No");
     }

     return ;
 }