【SP2916】Can you answer these queries V - 线段树

题面

You are given a sequence \(a_1,a_2,...,a_n\). (\(|A[i]| \leq 10000 , 1 \leq N \leq 10000\)). A query is defined as follows: Query(x1,y1,x2,y2) = \(Max{a_i+a_{i+1}+...+a_j;x_1 \leq i \leq y_1 , x_2 \leq j \leq y_2}\) and \(x_1 \leq x_2 , y_1 \leq y_2\). Given \(m\) queries (\(1 \leq M \leq 10000\)), your program must output the results of these queries.

题意

求所有左右端点分别在区间 \([x_1,y_1]\) 与 \([x_2,y_2]\) 的区间的最大连续子段和的最大值

思路

１° 两个区间不相交

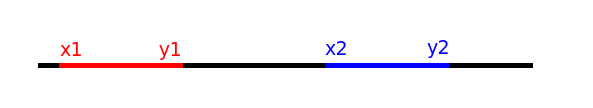
答案显然是左边区间的 rmax+中间不重叠部分的 sum+右边区间的 lmax，即：\([x_1,y_1].rmax+[y_1,x_2].sum+[x_2,y_2].lmax\)

２° 两个区间相交

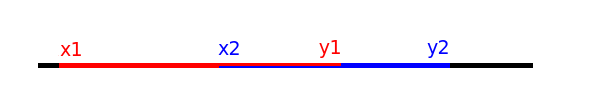
答案就会有三种情况

·① 答案区间为区间相交部分，即：\([x_2,y_1].max\)

·② 答案区间的左端点在相交部分左部，取相交部分左边的 rmax 和剩下区间的 lmax，再减掉加了两次的左边相交节点

即：\([x_1,x_2].rmax+[x_2,y_2].lmax-a_{x_2}\)

·③ 答案区间的右端点在相交部分右部，取相交部分右边的 lmax 和剩下区间的 rmax，再减掉加了两次的右边相交节点

即：\([y_1,y_2].lmax+[x_1,y_1].rmax-a_{y_1}\)

代码

/************************************************
*Author        :  lrj124
*Created Time  :  2019.09.27.21:55
*Mail          :  1584634848@qq.com
*Problem       :  spoj2916
************************************************/
#include <algorithm>
#include <cstring>
#include <cstdio>
using namespace std;
const int maxn = 10000 + 10;
struct seg { int l,r,sum,max; } tree[maxn<<2];
int T,n,q,a[maxn];
inline void pushup(int root) {
	tree[root].sum = tree[root<<1].sum+tree[root<<1|1].sum;
	tree[root].l = max(tree[root<<1].l,tree[root<<1|1].l+tree[root<<1].sum);
	tree[root].r = max(tree[root<<1|1].r,tree[root<<1].r+tree[root<<1|1].sum);
	tree[root].max = max(tree[root<<1].r+tree[root<<1|1].l,max(tree[root<<1].max,tree[root<<1|1].max));
}
inline void build(int l,int r,int root) {
	if (l == r) {
		tree[root] = { a[l],a[l],a[l],a[l] };
		return;
	}
	int mid = l+r>>1;
	build(l,mid,root<<1);
	build(mid+1,r,root<<1|1);
	pushup(root);
}
inline seg query(int l,int r,int ql,int qr,int root) {
	if (ql > qr) return {0,0,0,0};
	if (ql <= l && r <= qr) return tree[root];
	int mid = l+r>>1;
	if (mid >= qr) return query(l,mid,ql,qr,root<<1);
	if (ql > mid) return query(mid+1,r,ql,qr,root<<1|1);
	seg lson = query(l,mid,ql,qr,root<<1),rson = query(mid+1,r,ql,qr,root<<1|1),ans;
	ans = { max(lson.l,rson.l+lson.sum),max(rson.r,lson.r+rson.sum),rson.sum+lson.sum,max(lson.r+rson.l,max(lson.max,rson.max)) };
	return ans;
}
inline int solve(int l1,int r1,int l2,int r2) {
	if (r1 < l2) return query(1,n,l1,r1,1).r+query(1,n,r1+1,l2-1,1).sum+query(1,n,l2,r2,1).l;
	int ans = query(1,n,l2,r1,1).max;
	if (l1 < l2) ans = max(ans,query(1,n,l1,l2,1).r+query(1,n,l2,r2,1).l-a[l2]);
	if (r2 > r1) ans = max(ans,query(1,n,l1,r1,1).r+query(1,n,r1,r2,1).l-a[r1]);
	return ans;
}
int main() {
	for (scanf("%d",&T);T--;) {
		memset(tree,0,sizeof(tree));
		scanf("%d",&n);
		for (int i = 1;i <= n;i++) scanf("%d",&a[i]);
		build(1,n,1);
		for (scanf("%d",&q);q--;) {
			int l1,r1,l2,r2; scanf("%d%d%d%d",&l1,&r1,&l2,&r2);
			printf("%d\n",solve(l1,r1,l2,r2));
		}
	}
	return 0;
}